Unizor - Function Limit - Steepness and Constant 'e'

Unizor - Creative Minds through Art of Mathematics - Math4Teens

Steepness and Constant e

Another important concept we will be dealing with is a concept of a steepness of a curve that represents the graph of a real function y=f(x).

The concept of steepness of a curve at some point was introduced first in the chapter of Algebra dedicated to exponential functions. We have defined it, approximately, as a ratio of the increment of the Y-coordinate along the curve to an increment of the X-coordinate as we step forward from the original point where we want to measure the steepness.

We have also indicated that the smaller increment of X-coordinate (that is, the closer the next point on a curve lies to the point where we measure the steepness) - the better our approximation would be.

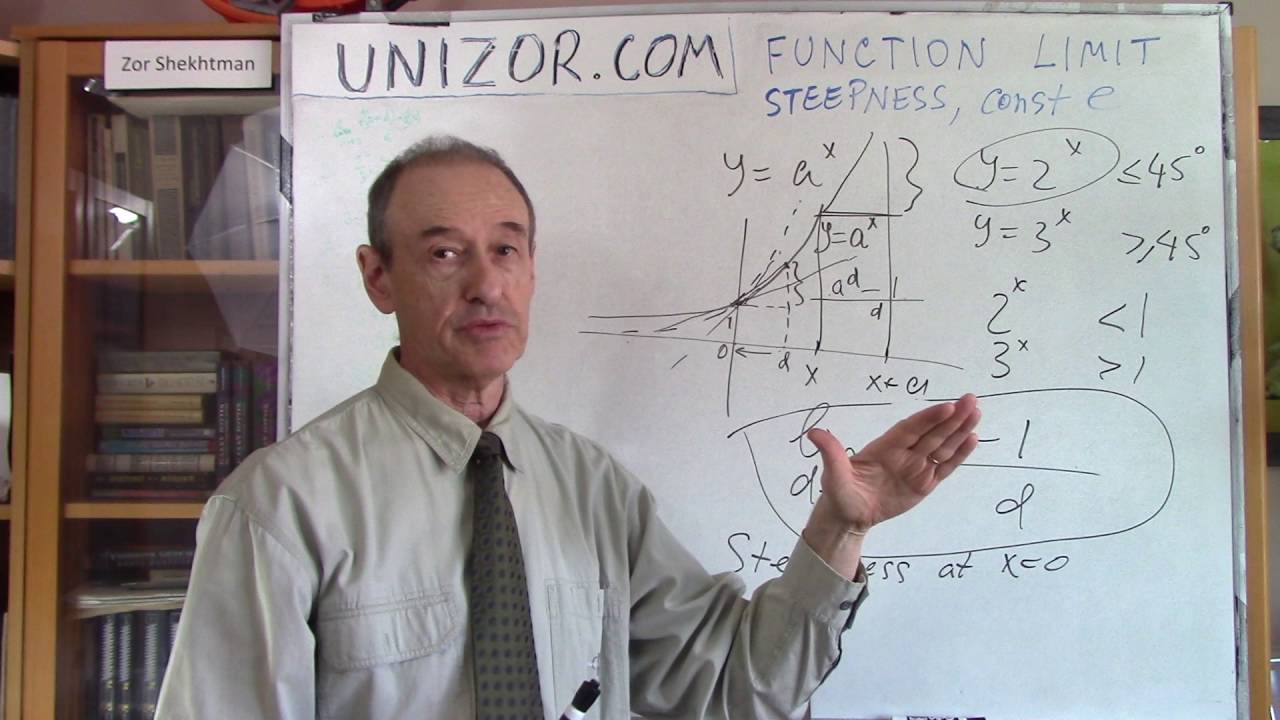
Using the terminology we are now familiar with, the steepness of a curve that represents the graph of a real function y=f(x) or, simply, the steepness of a real function y=f(x) at some point x=r is a limit of the ratio of f(r+d)−f(r) to d as d→0,
that is,
lim(d→0)[f(r+d)−f(r)] /d

When we introduced the exponential functions y=ax in the chapter "Algebra - Exponential Function", we have proven that the steepness of function y=a^x at point x=0 depends on the value of its base a, and, for a=2 the steepness of function y=2^x is less than 1, while for a=3 the steepness of function y=3^x is greater than 1.

Assuming that the steepness is smoothly increasing, as we increase the base from a=2 to a=3, it is reasonably to assume the existence of such base between these two values, for which the steepness at point x=0 is equal exactly to 1.

This value, obviously, is not an integer and, as can be proven, not even rational. Traditionally it is designated by the letter e (probably, in honor of a famous mathematician Euler who extensively researched its properties) and is considered as a fundamental mathematical constant, like π.
The approximate value of this constant is 2.71.

Now we can state that
lim(d→0)[er+d−er] /d = 1

The above can be accepted as a definition of a number e. There are a few others, all equivalent to this one, that is each definition can be proven based on another definition.
Here are a few.
lim(n→∞)(1+1/n)^n = e
lim(d→0)(1+d)^(1/d) = e
limn→∞[n·(n!)^(-1/n)] = e
limn→∞(1/0!+1/1!+...+1/n!) = e
The last one can be formulated using infinite summation sign as
Σ(1/n!) = e (sum for n=0, 1,...to ∞)

In this course we will assume the definition of number e as a base of an exponential function y=e^x that has a steepness of 1 at point x=0.

It means that we consider as given the following statement, constituting the defining property of number e.

For any, however small, positive ε exists δ such that
IF |d| ≤ δ
THEN |(e^d−e^0)/d − 1| ≤ ε
or, considering e^0=1,
|(e^d−1)/d − 1| ≤ ε

For those math purists, the existence and uniqueness of this number e was not rigorously addressed. We just relied on the monotonic smoothness of steepness of an exponential function y=a^x at point x=0 as we increase the base from a=2, when this steepness is less than 1, to a=3, when it is greater than 1.

[theoremus]

Thank you Zor. I recommend that you omit angle, when describing steepness. Just use the ratio of rise to run.

[ZorShekhtman]

We assume that studying Mathematics makes people more creative, more logical, simply smarter. Please share this video with your friends to make humanity smarter.

This lecture is a part of the educational Web site UNIZOR.COM dedicated to presentation of advanced course of Mathematics and Physics for teenagers interested in studying these subjects as tools to develop their creativity, analytic thinking, logic and intelligence.
Viewers are encouraged to go directly to UNIZOR.COM for the latest curriculum and other educational materials, including videos, detailed notes to them, exams etc.