L07.3 Conditional Expectation & the Total Expectation Theorem

preview_player
Показать описание
MIT RES.6-012 Introduction to Probability, Spring 2018
Instructor: John Tsitsiklis

License: Creative Commons BY-NC-SA
  1. MIT OpenCourseWare
  2. Conditional Expectation
  3. Total Expectation Theorem
Рекомендации по теме
L07.3 Conditional Expectation 0:06:10

L07.3 Conditional Expectation & the Total Expectation Theorem

L13.2 Conditional Expectation 0:04:31

L13.2 Conditional Expectation as a Random Variable

The Conditional Expectation 0:04:11

The Conditional Expectation of X given that Y=y

L07.2 Conditional PMFs 0:10:48

L07.2 Conditional PMFs

Lecture 26 Conditional 0:47:49

Lecture 26 Conditional Expectation + Sum of Two Random Variables

L09.3 Conditioning Example 0:03:08

L09.3 Conditioning Example

L07.5 Example 0:04:44

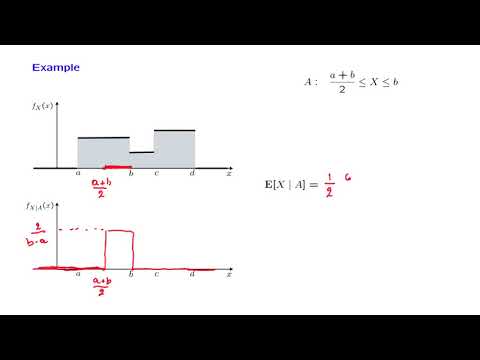
L07.5 Example

L06.4 Conditional PMFs 0:07:31

L06.4 Conditional PMFs & Expectations Given an Event

[Chapter 7] #5 0:03:24

[Chapter 7] #5 Conditional expectation

Conditional Variance: Example 0:08:48

Conditional Variance: Example 1

MA 381: Section 0:07:18

MA 381: Section 8.3: Conditional Probability Density Function for Continuous Random Variables

eco420 L07 3 0:09:33

eco420 L07 3

Probability Video 4.5: 0:25:40

Probability Video 4.5: Pairs of Random Variables - Conditional Expectation

Joint Distributions(3): Conditioning 0:15:34

Joint Distributions(3): Conditioning for Expected Values and Variances

computing conditional expectations 0:11:36

computing conditional expectations 03 conditional expectation given a simple sigma algebra

L07.8 The Hat 0:16:09

L07.8 The Hat Problem

Probabilistic Analysis - 0:49:42

Probabilistic Analysis - Lecture 36 (IE 523)

Conditioning on Continuous 0:06:52

Conditioning on Continuous Random Variables

Probability 4.7 Conditional 0:17:43

Probability 4.7 Conditional Expectation (2022)

S07.3 Independence of 0:06:51

S07.3 Independence of Random Variables Versus Independence of Events

Proof: Mean Independence 0:06:10

Proof: Mean Independence Implies Uncorrelatedness

Abstract Bayes' Formula 0:35:34

Abstract Bayes' Formula and Conditional Expectation

08-09. Conditional expectation 0:22:00

08-09. Conditional expectation - Conditioning: the matching rounds problem.

mod09lec71 - Conditional 0:24:56

mod09lec71 - Conditional Expectation

Комментарии
Автор

At the end of the lecture, the professor said the harder mathematical work is needed to show that the total expectation theorem also holds for the case when the discrete r.v. ranging over an infinite set.

My intuition about this is that the hardness originates from the absolute convergence or conditional convergence of infinite series. If you fix Y=y, the size of sub-universe can also be infinite. That means sometimes you can reorder the terms to make the summation to converge or to diverge. Hence the condition E[|x|]<∞ is needed.

For the case of total probability theorem, the absolute convergence is guaranteed by the probability axioms. This is my understanding of the hardness and please correct me if I am wrong.

LeeiFJaw
Автор

The infinite set of values of y can be uncountable?

tahacasablanca