filmov
tv
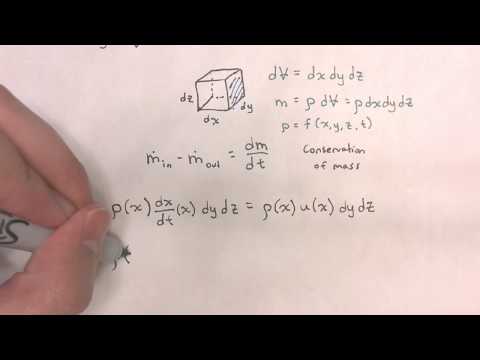
Derivation of the Mass Continuity Equation

Показать описание
In this video, we will derive the mass continuity equation by having a look at a simple Control Volume (CV). This derivation will then be used for a subsequent video to derive the Navier-Stokes Equations. Animations from Grant Sanderson (aka. 3Blue1Brown) are included.
ONLINE PRESENCE
================
SUPPORT MY WORK
=================
RECOMMENDATIONS
==================
🎵 Cinematic Drama - Epic Royalty Free Background Music
––––––––––––––––––––––––––––––
Contact:
#NavierStokes
#MassConservation
#Mathematics
Time Stamps
----------------------
0:00 - 1:11 : Intro
1:12 - 3:32 : Reynold's Transport Theorem
3:33 - 4:25 : Differential Form
4:26 - 4:55 : Fundamental Equations of Fluid Mechanics
4:56 - 5:19 : Conservation of Mass
5:20 - 6:00 : Momentum Equation
6:01 - 6:08 : Recap Terminology
6:09 - 6:20 : Control Volume (CV)
6:21 - 6:33 : Conservation of Mass (in words)
6:34 - 10:55 : Derivation of the Mass Continuity Equation
10:56 - 14:58 : Explanation of the Divergence
14:59 - End : Outro
Комментарии
 0:15:45
0:15:45
 0:18:36
0:18:36
 0:19:01
0:19:01
 0:06:04
0:06:04
 0:06:46
0:06:46
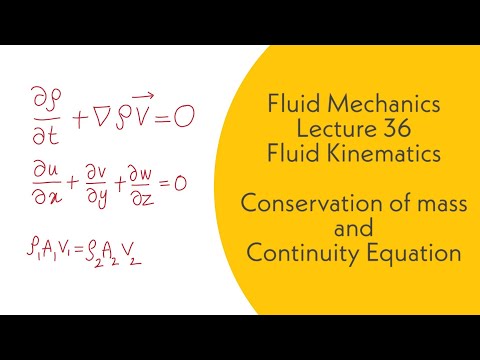 0:18:38
0:18:38
 0:09:32
0:09:32
 0:08:09
0:08:09
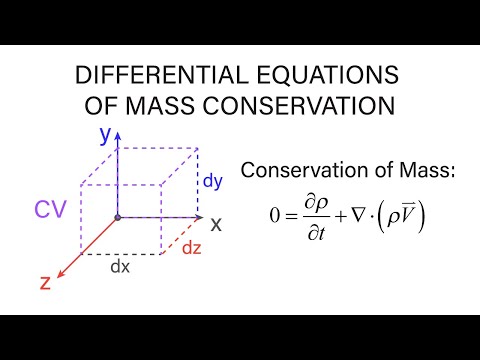 0:10:17
0:10:17
 0:03:51
0:03:51
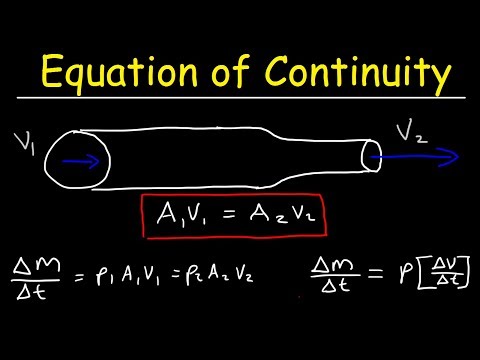 0:14:01
0:14:01
 0:04:05
0:04:05
 0:09:27
0:09:27
 0:36:40
0:36:40
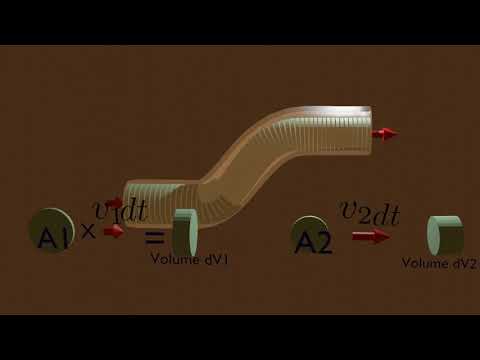 0:01:58
0:01:58
 0:24:17
0:24:17
 0:16:52
0:16:52
 0:51:11
0:51:11
 0:13:06
0:13:06
 0:09:37
0:09:37
 0:14:11
0:14:11
 0:03:49
0:03:49
 0:20:49
0:20:49
 0:55:52
0:55:52