16 - What do Imaginary & Complex Roots of Equations Mean?

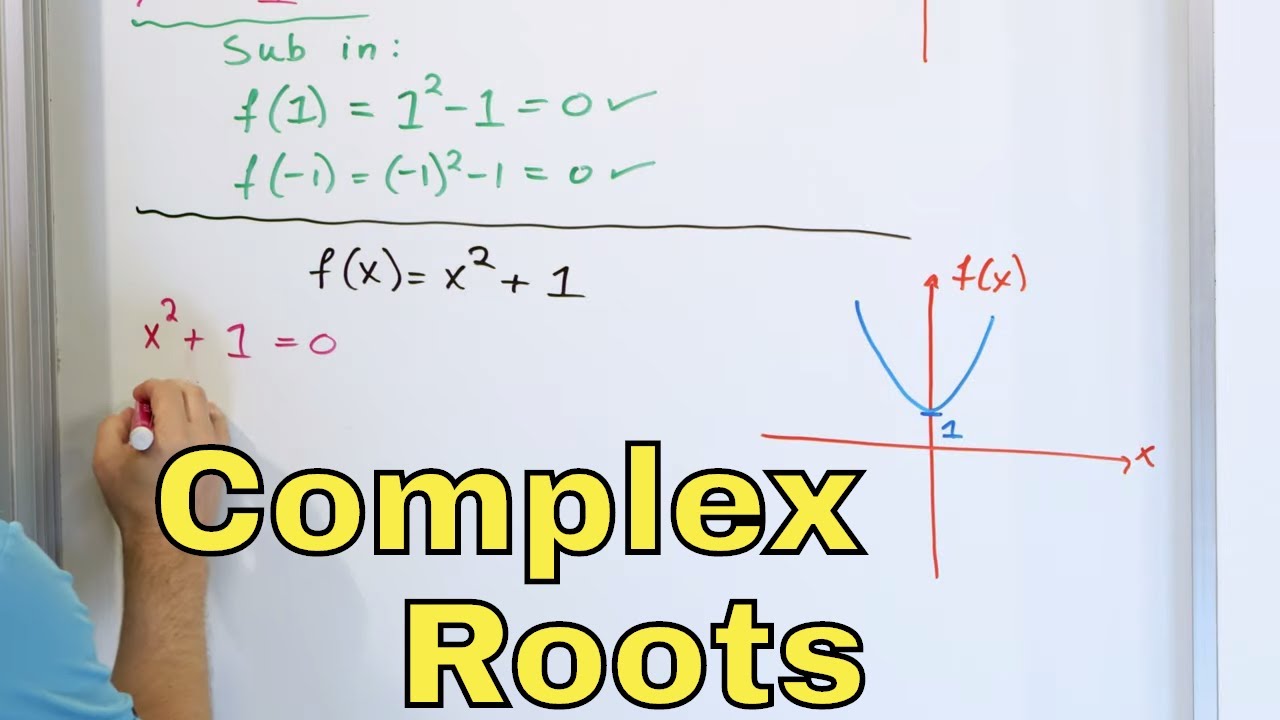
In this lesson, we will discuss complex roots of polynomial equations. Specifically, we want to understand what a complex root physically means. Complex roots arise when the quadratic has no crossing points along the x-axis.

We discuss the idea that all functions can take as inputs complex numbers, so the complex roots are just those values that drive the function to zero in the complex plane.

[DavidRodriguez-errq]

Yes, your presentations are contributing to students that will create big things. Your teachings, insights, and presentations have helped so many people and more to come. Thank you and God bless.

[dandan]

I'm speculating -- and wondering why you didn't make it clear -- that what you're showing along the f(z) axis is, in fact, the corresponding absolute value, namely: |f(z)| = |a+bi| = sqrt(a^2+b^2). The comments below seem to indicate that viewers are confused by the "f(z)" axis, rightfully observing that it's impossible to visualize f(z) using a single axis, seeing that f(z) is a complex number just like z.

[acho]

Absolutely amazing AND mind-blowing! Thank you for your work, this cleared up a lot! So basically the complex dimention is like the third dimention?

[poojaandprajwal]

The short talk you had with the viewers at the beginning made me feel motivated and pushed me to complete this entire vid. Totally satisfied!!

[poiesh]

Happy math teacher from Sweden that saw this for the first time. Amazing explanation that solved a huge issue when I teach this in upper secondary school!

[amjaddahabreh]

Thanks so much for making the Ambiguity of Math a piece of cake. Really thanks...

[colinl]

Best explanation of imaginary numbers I have ever seen - thanks.

[stewartmoore]

This is brilliant. Intuitive explanations always help in maths.

[wodeyaeric]

Jason, i am back here after some time. You teach this math so easily.

[zack_]

! 'Complex' thing made simple. The 3D computer animation of complex number roots is especially cool and makes this otherwise abstract and boring subject fun and easily understood! Superb video, thank you vert much!

[woodpalletprojects]

This was a wonderful explanation. Thank you for making imaginary concepts more understandable. This video was exactly what I was looking for. The visuals brought the whole concept together.

[nabilharrar]

I am lost for words, this video is absolutely mind boggling! Will never be able to look at mathematics the same

[josphatbanda]

It's amazing and motivating that maths can be taught that way. Thanks very much.

[cliffordmorris]

Brilliant graphical help to see the plane. All schools should show it as part of their courses. You are a fantastic teacher.

[kossyoto]

Brilliant and absolutely stunning! What a great explanation and visualization. The way you express your thoughts through the language is mind blowing, your explanation is incredibly clear throughout the whole lesson which is very very rare. Thanks for all the effort you made to create this program just so we are able to see the beauty of the complex numbers. One of the best videos I’ve ever seen!

[flobydemacongo]

Thanks a lot. I am 52 and I finally grab the concept of imaginary numbers. 🎉🎉🎉🎉

[susanstjohn]

I am 75 and have finally after years of teaching Scienec understand complex numbers and roots

[Dan-ltvm]

Thank you very much for the wonderful video! I was wondering if you might be able to clarify how to interpret f(z) in that computer program.

[mrjnutube]

Absolutely top drawer. You made it such easy to conceptualize!

[nobleidowu]

I am glad, I finally find solace in this site. @39+ I can still learn what I have ever wished to. Thanks for the great job.