Calculate the angle X and justify | Learn how to Solve the Geometry problem Quickly

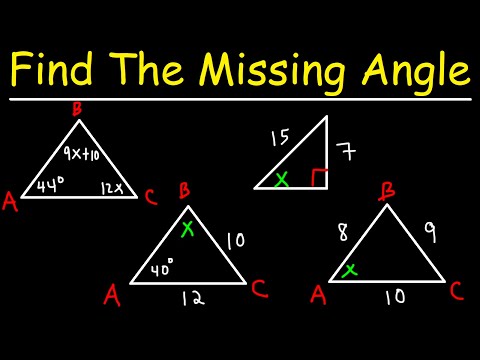
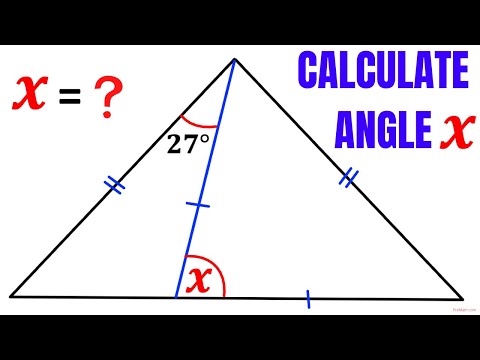
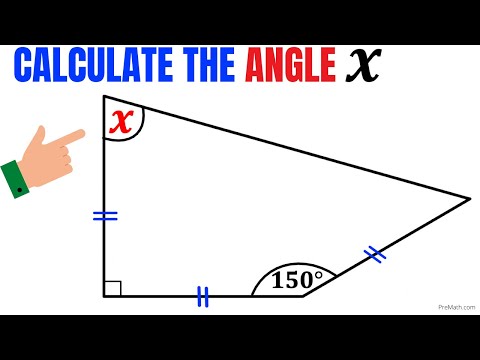
Learn how to find the angle X in the given diagram. Solve this tricky geometry problem by using the isosceles triangle properties and Exterior Angle Theorem.

Need help with finding the angle X in this complex Geometry question ? You're in the right place!

I have over 20 years of experience teaching Mathematics at American schools, colleges, and universities. Learn more about me at

Calculate the angle X and justify | Learn how to Solve the Geometry problem Quickly

#FindAngleX #Geometry #GeometryMath #ExteriorAngleTheorem
#OlympiadMathematics #CollegeEntranceExam #OlympiadPreparation
#PythagoreanTheorem #Pythagorean #Triangle #Triangles
#HowtoCalculatethedistance #Findthedistanceofthelinesegment #LineSegment #length #distance #blackpenredpen #ComplementaryAngles #OlympiadMathematics
#FindtheAngleX #HowtoSolvethisTrickyGeometryProblemQuickly #IsoscelesTriangleProperty
#IsoscelesTriangle #IsoscelesTriangles #Isosceles #Triangle #Triangles

Olympiad Mathematics
pre math
Po Shen Loh
Learn how to find the angle X
Isosceles Triangles
Triangle
premath
premaths
Exterior Angle Theorem

Subscribe Now as the ultimate shots of Math doses are on their way to fill your minds with the knowledge and wisdom once again.

[solimana-soli]

Knowing that alfa+beta=127, for the reasons mentioned, I have considered the quadrilateral EFCD from which
X + 53 + 180 - alpha + 180 - beta = 360 that easily leads to x=74

[alster]

Tricky at first but after seeing the Angle Addition Postulate and the substitution technique, the problem became a piece of cake.


God bless from The Philippines 🇵🇭

[bigm]

Thanks Professor, you crushed those triangles in your vicelike grip!🥂

[dye]

Let us split E into <AEL=<LED=a, x, <BER=<REF=b, where L on AC and R on BC, this makes <ELC=<ERC=90. Now we have <REL=127=a+b+x, and also 2a+2b+x=180, so a+b=53, x=74

[theoyanto]

Superb!!!, more like this please...
I sure need the practice, you just seem to wave your hands and the solution appears, such great detailed explanation. Thanks again

[lindafromcalifornia]

You are such a blessing to so many. Keep up your great work. 👍

[MrPhilippos]

Consider the quadrilateral CDEF :
X+53 + y1 + y2 = 360 or
X + 53 + (180 - a) + (180 - b) = 360 or
X = ( a + b ) - 53 or ( exterior angle )
X = 127 - 53 = 74 😊

[jasonk]

Wow. You walked us through that like it was a walk in the park! You made it look so easy. That was kind of a fun journey.

[johnspathonis]

I tackled this from a geometric direction. Since the question was general in nature then the answer must work for all positions of E along AB. Let CA equal CB in length and let E be located mid length.. The rest is just fill in the spaces.

[jim]

Excellent! Love the logical application of geometric theorems to solve the problem.

[annatygrys]

Superb, sir! I choice "the long way around Robinhood's barn" to receive a system of 3 equations with three unknowns :x, a and b. The first step was obvious :The exterior angle 127=a +b. Thus a=127-b Later on I used two other exterior angles :180-2a +x =2b and 180-2b +x=2a As next I substituted 180 - 2(127-b)+x=2b 180-254+2b+x=2b (-74) =(-x) x=74

[fried_ady]

We can see that 127 is an exterior angle to triangle CAB, so then it will be the sum of angle A and angle B (external angle postulate). That means angle A and angle B add up to 127. Now, we look at triangle AED and triangle BEF. Since they are both isoceles, angle A will equal angle EDA, and angle B will equal angle EBF. So, if we were to add these two triangles together, we would get a sum of 360 degrees. 360= angle A + angle A (EDA, reflexive property) + angle B + angle B (EFB, reflexive property) + angle DEA and angle FEB. Since there are two pairs of angle A + B, which add up to 127, we can substitute and simplify. Therefore, 360=254+angle EFB+ angle DEA. Therefore, angles EFB + DEA have a sum of 360-254=106. Then we can look at the straight angle E. It consists of X + DEA + EFB. Substituting the sum of DEA and EFB, we get x+106=180, thus x =74 degrees.

[roycuyler]

You are a very good instructor, sir. Thank you.

[vandanakumari]

Yayyy New video!
Thank you 💕 professor

[murdock]

Absolutely stunning, awesome, many thanks, Sir!

[honestadministrator]

Herein.
angle ABC + angle CAB = 127°
Hereby angle AED + angle BEF
= (180° - 2 angle DAE)
+ (180° - 2 angle FBE)
= 360° - 2 x 127° = 106°
Hereby x°
= 180° - (angle AED + angle BEF)
= 74°

[santiagoarosam]

The initial conditions of the problem do not prevent me from considering AC=CB → ∆ACB is isosceles just like ∆ADE and ∆EFB → Drawing a parallel to CB through vertex A, it is verified that ∠A=127º/2 → ∠A=∠B =127º/2 → ∠AED=∠BEF=180º -(2*127º/2)=180º -127º=53º → ∠DEF=180º -53º -53º= 74º=X
Thanks for the curious problem and best regards

[sngmn]

Ninty nine percent of all your videos are EASY? but easy for me butnot for my scoolers. You are great, thanx u

[abdulwahab_]

The conditions are not given but AC // EF and interior angle is 53 so according to corresponding angles angleF is 53 and since FBE is isosceles so angle B is also 53 and angle FEB will be 74. According to corresponding angles angle A is 74 also and angle D is also 74 since ADE is isosceles and angle E will be 32. So x will be 180 - 74 -32 = 74

[sameralkhabaz]

Thanks professor, Which program do you use to draw diagram?