filmov
tv
Mean Value Theorem For Integrals
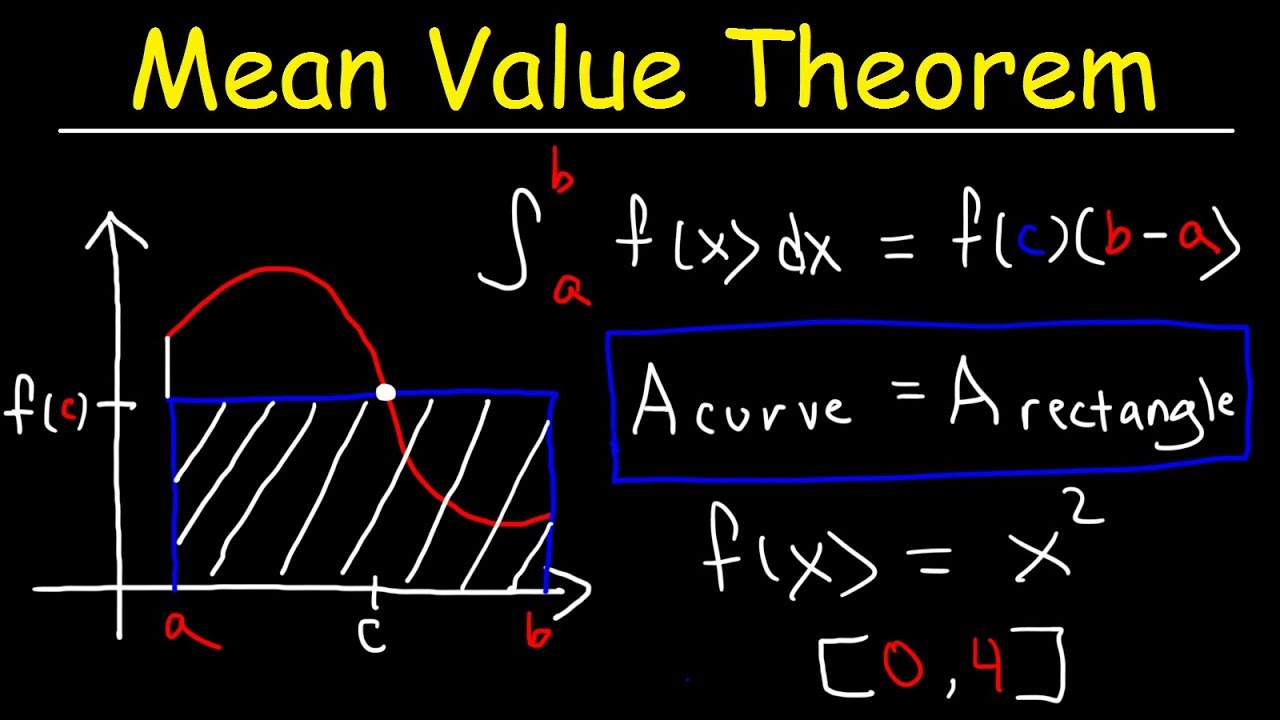
Показать описание
This calculus video tutorial provides a basic introduction into the mean value theorem for integrals. It explains how to find the value of c in the closed interval [a, b] guaranteed by the mean value theorem where the area under the curve is equal to the area of the rectangle at x = c. This video contains plenty of examples and practice problems. You need to be familiar with the process of finding antiderivatives and evaluating definite integrals.
Antiderivatives:
Fundamental Theorem - Part 1:
Fundamental Theorem - Part 2:
Net Change Theorem:
Mean Value Theorem - Integrals:
________________________________
Average Value of a Function:
U-Substitution - Indefinite Integrals:
U-Substitution - Definite Integrals:
1st Order Differential Equations:
Initial Value Problem:
________________________________
Area Between Two Curves:
Disk and Washer Method:
Volume By The Shell Method:
Volume By Cross Sections:
Arc Length Calculus Problems:
__________________________________
Calculus Final Exam and Video Playlists:
Full-Length Videos and Worksheets:
Antiderivatives:
Fundamental Theorem - Part 1:
Fundamental Theorem - Part 2:
Net Change Theorem:
Mean Value Theorem - Integrals:
________________________________
Average Value of a Function:
U-Substitution - Indefinite Integrals:
U-Substitution - Definite Integrals:
1st Order Differential Equations:
Initial Value Problem:
________________________________
Area Between Two Curves:
Disk and Washer Method:
Volume By The Shell Method:
Volume By Cross Sections:
Arc Length Calculus Problems:
__________________________________
Calculus Final Exam and Video Playlists:
Full-Length Videos and Worksheets:
Комментарии
 0:14:27
0:14:27
 0:07:24
0:07:24
 0:09:07
0:09:07
 0:12:09
0:12:09
 0:06:07
0:06:07
 0:07:20
0:07:20
 0:12:41
0:12:41
 0:04:13
0:04:13
 0:24:23
0:24:23
 0:06:32
0:06:32
 0:03:15
0:03:15
 0:06:32
0:06:32
 0:07:22
0:07:22
 0:08:36
0:08:36
 0:16:33
0:16:33
 0:02:27
0:02:27
 0:05:46
0:05:46
 0:19:29
0:19:29
 0:04:32
0:04:32
 0:10:01
0:10:01
 0:14:00
0:14:00
 0:03:43
0:03:43
 0:07:09
0:07:09
 0:08:02
0:08:02