The Double Dual Space – Tensors #9

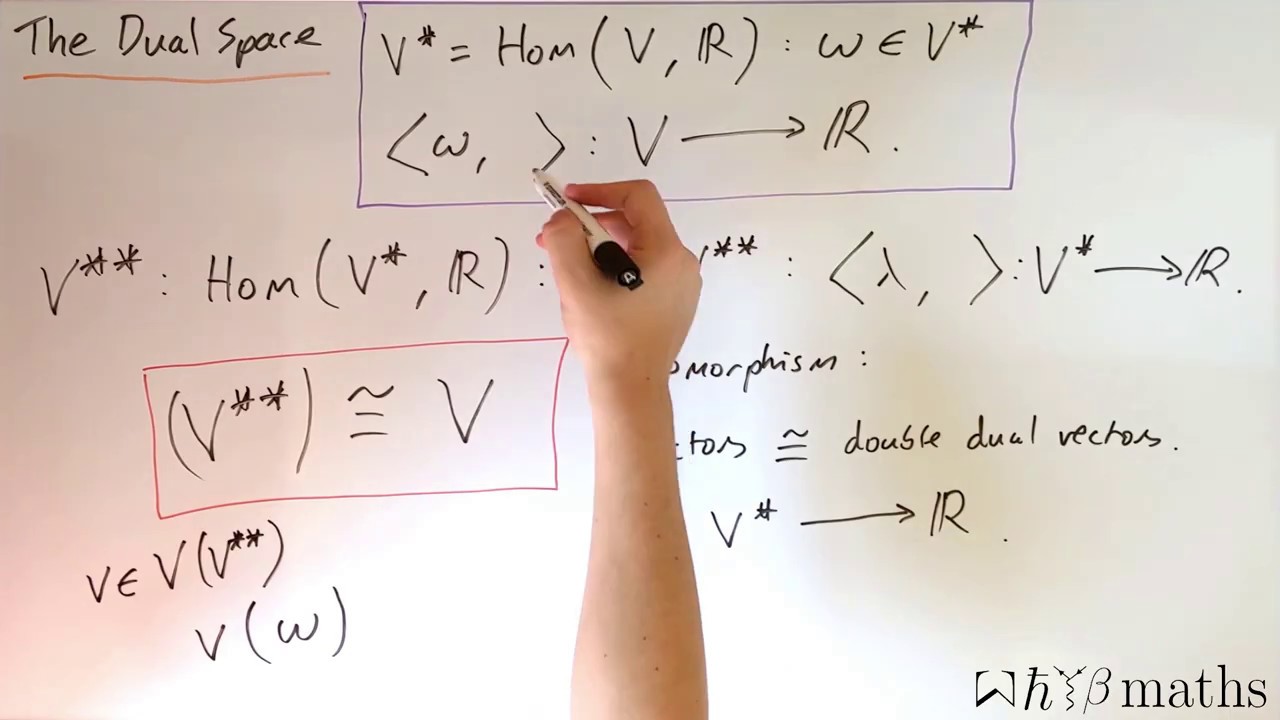
preview_player
Показать описание

This is a fairly informal discussion of how we realise that the double dual space (in the finite case) can be regarded as isomorphic to (the same as) the original vector space. We therefore realise that whilst dual vectors are linear maps from vectors into R, we can equally view vectors as linear maps from dual vectors into R.

Apologies for the harsh "ess" sounds, tried to filter it as much as possible but my mic isn't great!!
  1. WHYB maths
  2. Maths
  3. Math
  4. Physics
  5. Tensor
  6. Vector
Рекомендации по теме
Dual Space 0:11:24

Dual Space

The Double Dual 0:05:00

The Double Dual Space – Tensors #9

Double Dual 0:15:58

Double Dual

Double Dual Vector 0:20:21

Double Dual Vector Spaces Part 1

The Dual Space 0:16:14

The Dual Space

Linear Algebra -Dual 0:18:59

Linear Algebra -Dual Space,Dual Basis and Double Dual

#HelpUsExist - Double 0:00:15

#HelpUsExist - Double Dual Space

Double Dual Vector 0:28:38

Double Dual Vector Spaces Part 2

Polestar 3 '25 0:07:07

Polestar 3 '25 Long Range Dual Motor || Exterior & Interior

Linear Algebra: dual 0:52:11

Linear Algebra: dual space, double dual, dual basis and annihilator of subspace, 9-11-24

Linear Algebra Lecture 0:11:44

Linear Algebra Lecture 3.2 The Double Dual

Lecture 137 (Second 0:14:21

Lecture 137 (Second Dual Space)

Advanced Linear Algebra, 0:23:36

Advanced Linear Algebra, Lecture 1.5: Dual vector spaces

Linear Algebra, Lecture 0:50:46

Linear Algebra, Lecture 4 (Transpose of a Linear Operator, and Double Dual Space)

Duality in Linear 0:27:12

Duality in Linear Algebra: Dual Spaces, Dual Maps, and All That

DUAL BASIS LINEAR 0:20:54

DUAL BASIS LINEAR ALGEBRA | Dual Basis Examples by Dr Vineeta Negi

Dual space Meaning 0:00:29

Dual space Meaning

Linear Algebra - 0:18:53

Linear Algebra - Dual Space, Dual Basis, Double Dual

The Dual Space 0:12:16

The Dual Space – Tensors #8

Lecture 6: The 1:20:58

Lecture 6: The Double Dual and the Outer Measure of a Subset of Real Numbers

Dot products and 0:14:12

Dot products and duality | Chapter 9, Essence of linear algebra

Lecture 15: Operators, 0:33:10

Lecture 15: Operators, duals, dual basis

Dual Spaces 0:27:45

Dual Spaces

Dual Basis - 0:11:26

Dual Basis - Dual Space - Linear Algebra - Chapter 8 - BA/BSC Final Year

Комментарии
Автор

This went full circle really fast lol
Really love this quick-video format, I'll be sure to check out the details of the double dual space
Love your work man

gluonsmx
Автор

Thank you. I wish we could have gone deeper into this, though, explaining the equality of V and V**.

drlangattxdotnet
Автор

Am I right in coming to the conclusion that "V* = Hom(V, \mathbb{R}) iff V = Hom(V*, \mathbb{R})?

paradigmshift
Автор

But can you do it with a Z-module M. And the dual M* = Hom(M, Z)?

InfiniteQuest