Calculus 1 Exam Review Part7'

In this live stream, we will review various problems likely to appear in your Final Exam.
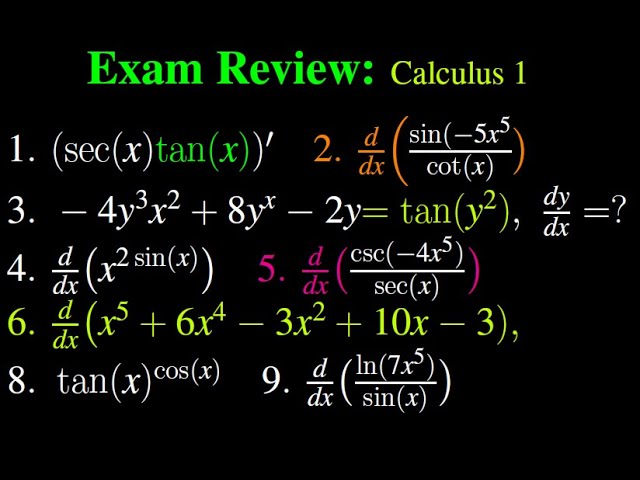
Calculus 1 Exam Review
Continuity Problem 1: Determine if the following function is continuous at \magenta x=-1. If not, determine the type of discontinuity it has:

g(x) = \left \{\begin{array}{ll} \disp \frac{ -1 -x }{ -3 -2x + 4x^2 + 3x^3 } &\,\, \hbox{if} \,\, x\neq-1\\
3x-5&\,\, \hbox{if} \,\, x = -1
\end{array} \right.

In problem 1: Find the antiderivative of the function e^( x )e^( x )^(3).

Problem 2: Compute the following limit.\\
\lim_{x \rightarrow 3 }\frac{\sqrt{46 + x}-7}{-3 + x}=\magenta ? \no

Problem 3: Compute the following limit.\\
\lim_{x \rightarrow 3 }\frac{9 -6x + x^2}{9 -6x -1x^2 + 4x^3}\\

Problem 3: Find derivative of f(x) = \frac{ 8x-9 }{ -10x+5 } using the definition.
Continuity Problem 1: Determine if the following function is continuous at \magenta x=-6. If not, determine the type of discontinuity it has:

g(x) = \left \{\begin{array}{ll} \disp \frac{ -6 -x }{ -18 -9x -19x^2 -3x^3 } &\,\, \hbox{if} \,\, x\neq-6\\
5x-5&\,\, \hbox{if} \,\, x = -6
\end{array} \right.



{ Problem 5}}

9y^3x^2-4y^2x-7y = \cot(y). \\

\disp \frac{ dy }{ dx } = \,\, \magenta ?

Indeterminate Form: Compute the limit \\ \lim_{x\to 0^+} \frac{ \cos(6x)+(-1)e^{ -3x } }{ (-3)\sin(x) } .
{ Problem 7 }}\\ \[ \frac{d}{dx}\bigg( 6x^2+7x+1 \bigg). \] Find the tangent line at x = 1.
Problem 8 }}\\ Determine the derivative \frac{d}{dx}\bigg( x^2^{\cot(x)} \bigg) \\
Continuity Problem 1: Determine if the following function is continuous at \magenta x=8. If not, determine the type of discontinuity it has:

g(x) = \left \{\begin{array}{ll} \disp \frac{ 8 -x }{ 64 -16x + x^2 } &\,\, \hbox{if} \,\, x\neq8\\
9x+9&\,\, \hbox{if} \,\, x = 8
\end{array} \right.

In problem 9: Find the antiderivative of the function csc^2( x ) (-1)cot(x)^(7).

Problem 10: Compute the following limit.\\
\lim_{x \rightarrow -4 }\frac{4 + x}{\sqrt{13 + x}-3}=\magenta ? \no

Problem 11: Compute the following limit.\\
\lim_{x \rightarrow 3 }\frac{-12 + x -5x^2 + 2x^3}{3 -x}\\

Problem 3: Find derivative of f(x) = \frac{ -9x-6 }{ 3x-6 } using the definition.
Continuity Problem 1: Determine if the following function is continuous at \magenta x=1. If not, determine the type of discontinuity it has:

g(x) = \left \{\begin{array}{ll} \disp \frac{ 2x -6x^2 + 4x^3 }{ 1 -2x + x^2 } &\,\, \hbox{if} \,\, x\neq1\\
-7x&\,\, \hbox{if} \,\, x = 1
\end{array} \right.



{ Problem 13}}

-3y^2x-7y = \csc( y ) . \\

\disp \frac{ dy }{ dx } = \,\, \magenta ?

Indeterminate Form: Compute the limit \\ \lim_{x\to 0^+} \frac{ \ln(\tan(7x)+1) }{ (-3)\tan(x) } .
{ Problem 15 }}\\ \[ \frac{d}{dx}\bigg( -7x^3-4x^2+3x-3 \bigg). \] Find the tangent line at x = 0.
Problem 16 }}\\ Determine the derivative \frac{d}{dx}\bigg( \sin(x)^{\sin(x)} \bigg) \\
Continuity Problem 1: Determine if the following function is continuous at \magenta x=8. If not, determine the type of discontinuity it has:

g(x) = \left \{\begin{array}{ll} \disp \frac{ 8 -x }{ -8 + x -24x^2 + 3x^3 } &\,\, \hbox{if} \,\, x\neq8\\
3x+6&\,\, \hbox{if} \,\, x = 8
\end{array} \right.

In problem 17: Find the antiderivative of the function sec^2( x ) sin(tan(x)).

Problem 18: Compute the following limit.\\
\lim_{x \rightarrow 3 }\frac{\sqrt{6 + x}-3}{-3 + x}=\magenta ? \no

Problem 19: Compute the following limit.\\
\lim_{x \rightarrow -3 }\frac{-3 -x}{-9 + 9x + 7x^2 + x^3}\\

Problem 3: Find derivative of f(x) = \frac{ -6x-1 }{ -6x+7 } using the definition.
Continuity Problem 1: Determine if the following function is continuous at \magenta x=6. If not, determine the type of discontinuity it has:

g(x) = \left \{\begin{array}{ll} \disp \frac{ 6 -x }{ 12 + 4x -25x^2 + 4x^3 } &\,\, \hbox{if} \,\, x\neq6\\
-7x-8&\,\, \hbox{if} \,\, x = 6
\end{array} \right.



{ Problem 21}}

-8y^3x^2+y^2x-9y = \csc( y ) . \\

\disp \frac{ dy }{ dx } = \,\, \magenta ?

Indeterminate Form: Compute the limit \\ \lim_{x\to 0^+} \frac{ \cos(2x)+(-1)e^{ -3x } }{ -3x } .
{ Problem 23 }}\\ \[ \frac{d}{dx}\bigg( 5x^3-x^2+8x+8 \bigg). \] Find the tangent line at x = 0.
Problem 24 }}\\ Determine the derivative \frac{d}{dx}\bigg( \tan(x)^{e^{ x }} \bigg) \\
Continuity Problem 1: Determine if the following function is continuous at \magenta x=-3. If not, determine the type of discontinuity it has:

g(x) = \left \{\begin{array}{ll} \disp \frac{ -3 + 1x + 7x^2 + x^3 }{ 9 + 6x + x^2 } &\,\, \hbox{if} \,\, x\neq-3\\
x+5&\,\, \hbox{if} \,\, x = -3
\end{array} \right.

In problem 25: Find the antiderivative of the function csc^2( x ) ((-1)sin(cot(x))).

Problem 26: Compute the following limit.\\
\lim_{x \rightarrow -4 }\frac{4 + x}{\sqrt{68 + x}-8}=\magenta ? \no

Problem 27: Compute the following limit.\\
\