filmov
tv
Worked example: Motion problems with derivatives | AP Calculus AB | Khan Academy

Показать описание
Keep going! Check out the next lesson and practice what you’re learning:
Finding (and interpreting) the velocity and acceleration given position as a function of time.
Khan Academy is a nonprofit organization with the mission of providing a free, world-class education for anyone, anywhere. We offer quizzes, questions, instructional videos, and articles on a range of academic subjects, including math, biology, chemistry, physics, history, economics, finance, grammar, preschool learning, and more. We provide teachers with tools and data so they can help their students develop the skills, habits, and mindsets for success in school and beyond. Khan Academy has been translated into dozens of languages, and 15 million people around the globe learn on Khan Academy every month. As a 501(c)(3) nonprofit organization, we would love your help! Donate or volunteer today!
Finding (and interpreting) the velocity and acceleration given position as a function of time.
Khan Academy is a nonprofit organization with the mission of providing a free, world-class education for anyone, anywhere. We offer quizzes, questions, instructional videos, and articles on a range of academic subjects, including math, biology, chemistry, physics, history, economics, finance, grammar, preschool learning, and more. We provide teachers with tools and data so they can help their students develop the skills, habits, and mindsets for success in school and beyond. Khan Academy has been translated into dozens of languages, and 15 million people around the globe learn on Khan Academy every month. As a 501(c)(3) nonprofit organization, we would love your help! Donate or volunteer today!
Worked example: Motion problems with derivatives | AP Calculus AB | Khan Academy
Worked example: motion problems (with definite integrals) | AP Calculus AB | Khan Academy
Average velocity and speed worked example | One-dimensional motion | AP Physics 1 | Khan Academy
How To Solve Projectile Motion Problems In Physics
How To Solve Any Projectile Motion Problem (The Toolbox Method)
Two Dimensional Motion Problems - Physics
Kinematics Part 3: Projectile Motion
Motion problems: when a particle is speeding up | AP Calculus AB | Khan Academy
Exporting 3D Projects to Unreal Engine Made Easy | Part 3 (Free Course)
Dynamics - Lesson 2: Rectilinear Motion Example Problem
Curvilinear Motion: Normal and Tangential components (Learn to solve any problem)
Kinematics Part 1: Horizontal Motion
Solving Projectile Motion Problems in Physics - [1-4-7]
CALCULATIONS IN MOTION - PHYSICS
How to Solve Projectile Motion Problems (Step by Step)
Rectilinear Motion Problems - Distance, Displacement, Velocity, Speed & Acceleration
Rigid Bodies Relative Motion Analysis: Velocity Dynamics (Learn to solve any question step by step)
Rectilinear Kinematics: Erratic Motion (learn to solve any problem step by step)
Absolute Dependent Motion: Pulleys (learn to solve any problem)
Physics - Acceleration & Velocity - One Dimensional Motion
How To Solve Simple Harmonic Motion Problems In Physics
how to solve a sample equation of motion problem with worked solution
How to solve any projectile motion question
Using equations of motion (1 step numerical)
Комментарии
 0:04:58
0:04:58
 0:08:32
0:08:32
 0:04:52
0:04:52
 0:28:19
0:28:19
 0:13:02
0:13:02
 0:12:30
0:12:30
 0:07:06
0:07:06
 0:08:57
0:08:57
 1:00:56
1:00:56
 0:09:17
0:09:17
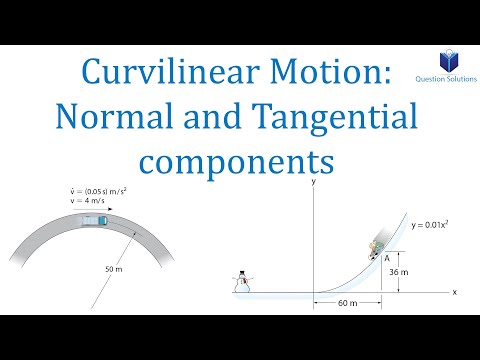 0:05:54
0:05:54
 0:06:38
0:06:38
 0:25:19
0:25:19
 0:14:37
0:14:37
 0:09:36
0:09:36
 0:16:14
0:16:14
 0:07:21
0:07:21
 0:10:16
0:10:16
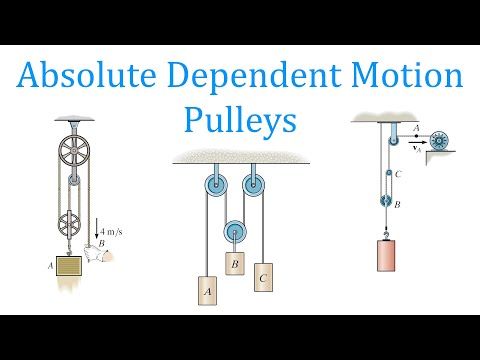 0:08:01
0:08:01
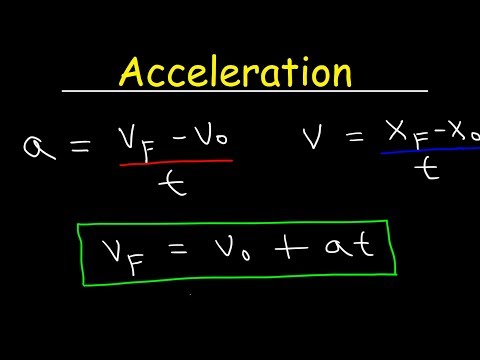 0:18:58
0:18:58
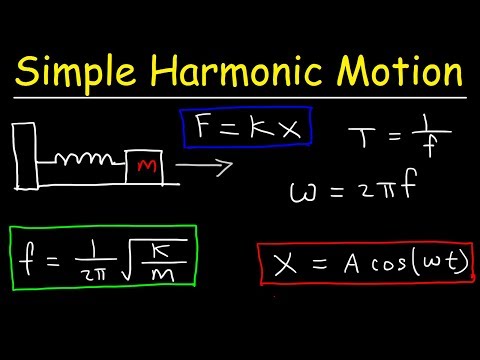 0:14:11
0:14:11
 0:03:18
0:03:18
 0:22:25
0:22:25
 0:11:54
0:11:54