filmov
tv
Square inside a triangle | Math Olympiad Geometry Problem
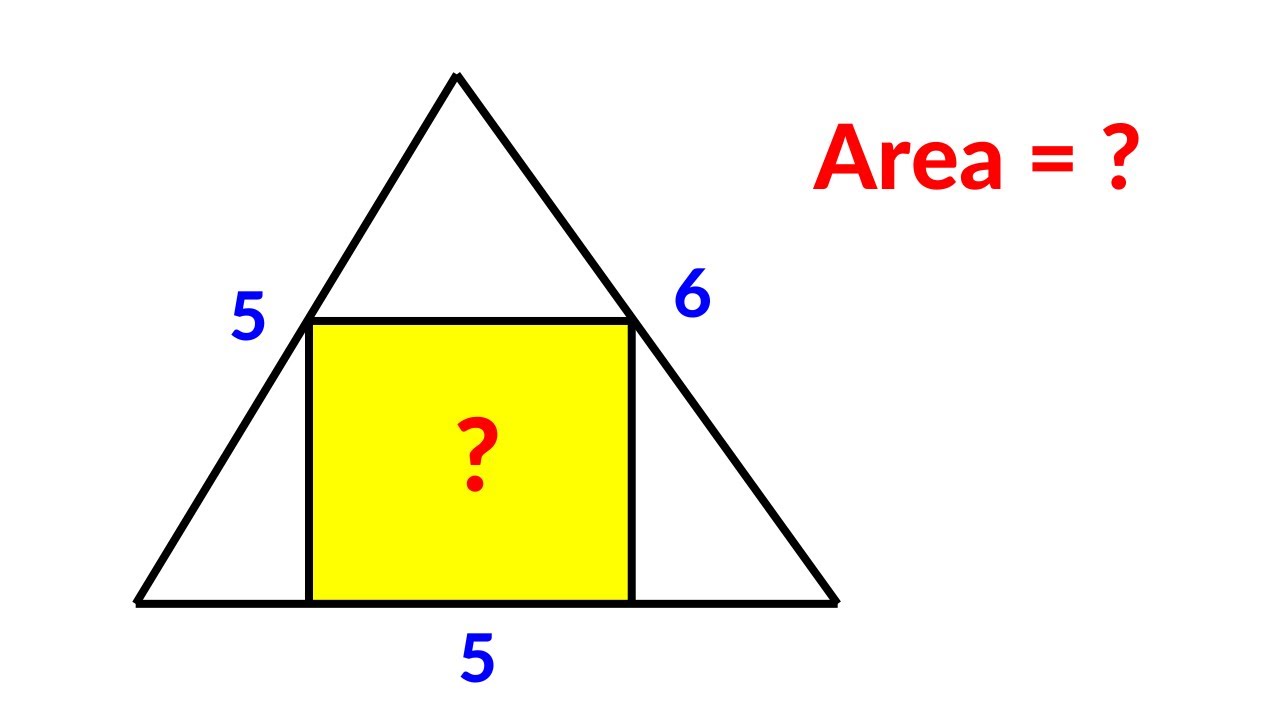
Показать описание
Square inside a triangle | Math Olympiad Geometry Problem
MY OTHER CHANNELS
••••••••••••••••••••••••••••••••
--------------------------------------------------------------------------------
Join the channel to become a member
MY OTHER CHANNELS
••••••••••••••••••••••••••••••••
--------------------------------------------------------------------------------
Join the channel to become a member
How to find side length of a square inside a triangle | Area of square inside a triangle
Find the Area of a Square Located Inside a Right Triangle - 4 Methods
Square inside right triangle problem | Geometry | Advanced math problems | Mathematics
Find the area of the square inside the triangle (Solution Hint Video)
Square inside a triangle | Math Olympiad Geometry Problem
Square inside triangle
Square inside a Equilateral Triangle | Find the ratio of areas #geometrymath
How to Find the Area of the Shaded Region | Triangle in a Square | Math with Mr. J
Geometry: Area of the region inside the triangle but outside the semicircle
Square In A 3-4-5 Triangle Puzzle
Square Inscribed in a Right Triangle. Geometry video.
Find the Area of the Larger Square Inside of a Right Triangle | Step-by-Step Explanation
Can You Calculate the Area of a Triangle that is Inside of a Square? | Step-by-Step Tutorial
Finding the Side Length of a Square Inscribed in a Right Triangle
Geometry, Level 2 of 6, Example 1, Square inscribed by right triangle
Find Angle of a triangle in a square A difficult problem with interesting solution PRMO RMO INMO IMO
Square Inscribed in a Triangle. Geometry video.
Find area of the Green Square inscribed in the right triangle | Important Geometry skills explained
How many degrees are inside a Triangle?
SQUARE INSIDE A TRIANGLE | #shorts | #mathssir | #TRIANGLE | #SQUARE | #geometry | #mathstricks |
AutoCAD 2D tutorial challenge exercise Draw maximum size square inside a triangle
Find the Area of the Green Square in this Scalene Triangle | Quick & Simple Tutorial
Constructing a square of area equal to a given triangle.
Side of a square inside right angled triangle | important concept | #shorts #maths #geometry #quant
Комментарии
 0:05:54
0:05:54
 0:13:18
0:13:18
 0:05:22
0:05:22
 0:00:15
0:00:15
 0:14:38
0:14:38
 0:04:45
0:04:45
 0:05:49
0:05:49
 0:04:18
0:04:18
 0:04:47
0:04:47
 0:02:29
0:02:29
 0:01:30
0:01:30
 0:08:50
0:08:50
 0:13:24
0:13:24
 0:02:47
0:02:47
 0:03:07
0:03:07
 0:02:35
0:02:35
 0:01:24
0:01:24
 0:09:27
0:09:27
 0:01:35
0:01:35
 0:00:58
0:00:58
 0:08:53
0:08:53
 0:04:54
0:04:54
 0:06:21
0:06:21
 0:01:00
0:01:00