filmov
tv
Square inside right triangle problem | Geometry | Advanced math problems | Mathematics
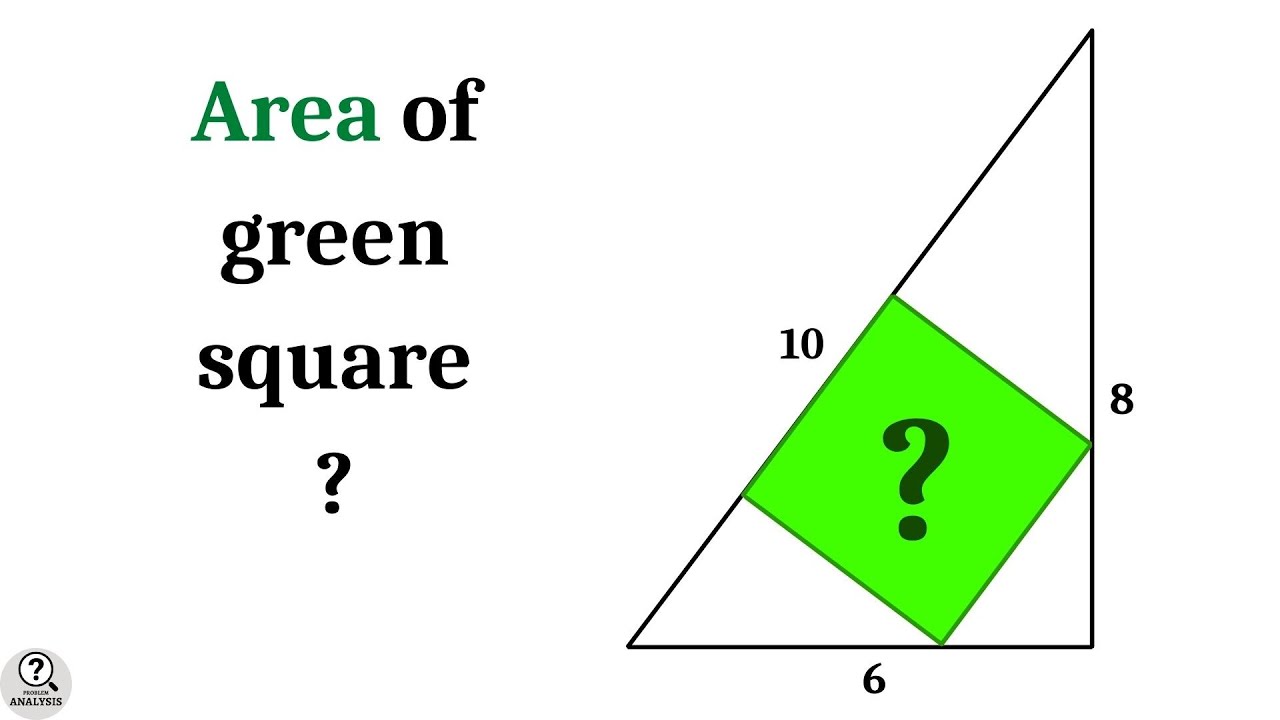
Показать описание
There are a bunch of problems which are seem to be very advanced, but only basic knowledge are required to solve. This problem is an application of the concept of similar triangles. Basic ideas about right triangles and similarity of triangles are needed to solve this problem.
Any queries regarding the subject or videos are invited.
Instagram ID : problem_analysis
#maths #mathematics #triangle #square #area #algebra #calculation #problems #geometry
Any queries regarding the subject or videos are invited.
Instagram ID : problem_analysis
#maths #mathematics #triangle #square #area #algebra #calculation #problems #geometry
Square inside right triangle problem | Geometry | Advanced math problems | Mathematics
Square inside right triangle problem | Geometry | Advanced math problems | Mathematics
Find the Area of a Square Located Inside a Right Triangle - 4 Methods
Geometry, Level 2 of 6, Example 1, Square inscribed by right triangle
Square In A 3-4-5 Triangle Puzzle
Square Inscribed in a Right Triangle. Geometry video.
Find the Area of the Larger Square Inside of a Right Triangle | Step-by-Step Explanation
finding the side length of a square inscribed in a right triangle
Mathematics Grade 10 November 2022 Paper 2 Memo Term 4 Exam @mathszoneafricanmotives
Find the area of the square inside the triangle (Solution Hint Video)
Calculate the length of the of square that inserted inside a right angle triangle #geometry
How to find side length of a square inside a triangle | Area of square inside a triangle
Square inside triangle
Find the side length of an inscribed square in a 'Right Triangle' | Similar triangles || ...
Find Angle of a triangle in a square A difficult problem with interesting solution PRMO RMO INMO IMO
Can you solve this innocent looking triangle problem?
Square Inscribed in a Triangle. Geometry video.
Isosceles triangle inside square | Find the angle | Advanced math problems | Mathematics
The Most Important Geometry Outcome | SQUARE inside RIGHT ANGLE TRIANGLE | Iscosceles Right Angle
2 Circles 1 Square
Circle and tangent inside square | Right triangle | Advanced math problems | Geometry | Mathematics
Find the Area of the Green Square in the right Triangle and Semicircle | Step-by-Step Tutorial
Area of a Rectangle, Triangle, Circle & Sector, Trapezoid, Square, Parallelogram, Rhombus, Geome...
3-4-5 Right Triangle Surprise in Every Square! 👀
Комментарии
 0:05:22
0:05:22
 0:00:39
0:00:39
 0:13:18
0:13:18
 0:03:07
0:03:07
 0:02:29
0:02:29
 0:01:30
0:01:30
 0:08:50
0:08:50
 0:02:47
0:02:47
 1:06:29
1:06:29
 0:00:15
0:00:15
 0:06:34
0:06:34
 0:05:54
0:05:54
 0:04:45
0:04:45
 0:05:47
0:05:47
 0:02:35
0:02:35
 0:05:30
0:05:30
 0:01:24
0:01:24
 0:05:26
0:05:26
 0:09:41
0:09:41
 0:03:35
0:03:35
 0:05:52
0:05:52
 0:07:22
0:07:22
 0:20:35
0:20:35
 0:00:27
0:00:27