filmov
tv
Laplace Transform and Piecewise or Discontinuous Functions

Показать описание
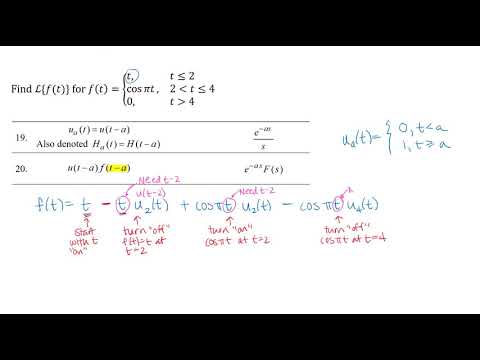
This video deals particularly with how the Laplace Transform works with piecewise functions, a type of discontinuous functions. The easiest example of which is the step function or heaviside function and we use that example to deal with the more complicated cases. Indeed, one of the big advantages of the Laplace Transform is that it deals with discontinuous functions so well converting them into a continuous function over in "Laplaceland" (a magical place). We will use these formulas as well to deal with inverse laplace transforms.
****************************************************
Other Course Playlists:
***************************************************
► Want to learn math effectively? Check out my "Learning Math" Series:
►Want some cool math? Check out my "Cool Math" Series:
****************************************************
*****************************************************
This video was created by Dr. Trefor Bazett. I'm an Assistant Teaching Professor at the University of Victoria.
BECOME A MEMBER:
MATH BOOKS & MERCH I LOVE:
Комментарии
 0:06:27
0:06:27
 0:04:44
0:04:44
 0:12:00
0:12:00
 0:09:21
0:09:21
 0:05:12
0:05:12
 0:01:43
0:01:43
 0:12:02
0:12:02
 0:00:46
0:00:46
 0:24:15
0:24:15
 0:08:45
0:08:45
 0:07:30
0:07:30
 0:10:14
0:10:14
 0:06:36
0:06:36
 0:08:02
0:08:02
 0:16:06
0:16:06
 0:06:09
0:06:09
 0:06:21
0:06:21
 0:03:34
0:03:34
 0:03:43
0:03:43
 0:05:23
0:05:23
 0:07:05
0:07:05
 0:16:55
0:16:55
 0:30:28
0:30:28
 0:27:54
0:27:54