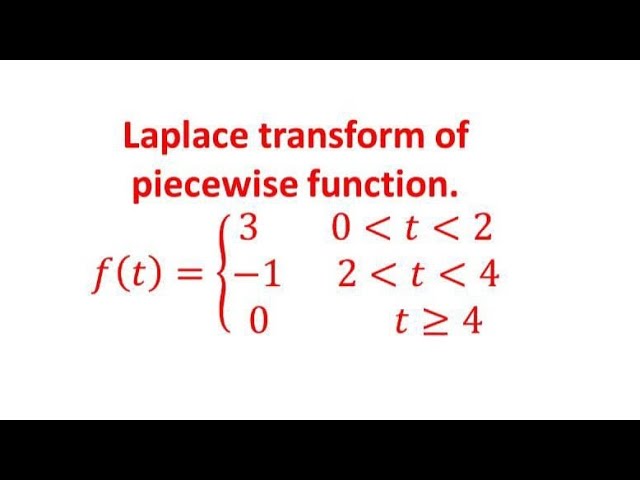
laplace transform, laplace transform of piecewise function

In mathematics, a piecewise-defined function (also called a piecewise function, a hybrid function, or definition by cases) is a function defined by multiple sub-functions, where each sub-function applies to a different interval in the domain. Piecewise definition is actually a way of expressing the function, rather than a characteristic of the function itself.In mathematics, the Laplace transform, named after its inventor Pierre-Simon Laplace, is an integral transform that converts a function of a real variable (often time) to a function of a complex variable  (complex frequency). The transform has many applications in science and engineering because it is a tool for solving differential equations. In particular, it transforms linear differential equations into algebraic equations and convolution into multiplication
Laplace transform of e^at.

[MimranSajid-kxvo]

Please share the next lec like application of laplace derivative

[coordina-lpdr]

According to the Laplace Transformation F(t) function defined on [0, positive infinity] then each piecewise subintervel will not be continuous?Here 0<t<2 and 2<t<4 at the point 2 is not continuous.Please clear my concept.

[alperenyogun]

hello, i had a question sir,
what if we have equality in our limits like; f(t)=3 when 0<=t<=2. how can we express the equation when we solve this?