filmov
tv
Cool math with the number 2025 for the new year!

Показать описание
The Simplest Math Problem No One Can Solve - Collatz Conjecture
15 Mind Blowing Math Facts | Insane but True
Amazing Math Hacks
The Most Wholesome Math Equation
If you are a genius solve this! Math Game Challenge!!!
Large Numbers
Magic with numbers | Number tricks | Smart Learning Tube
Game Theory: The Cool Math Games ARG Goes To Some Dark Places...
#maths #mathstricks #algebra #math #foryou #equation #fyp #mathshorts #shorts #short #mathematics
simple math
The Oldest Unsolved Problem in Math
If you are genius solve this | Cool Maths Game
I Can Guess Your Phone Number (10 digits) - Math Tricks Magic - I Can Guess Your Number Trick
Animation vs. Math
I Will Predict Your Number - Math Magic Trick
Math Magic
Math Challenge****
Nastya and dad are learning math
Something Strange Happens When You Keep Squaring
9 TIMES TABLE #shorts #math #maths #mathematics
Wendy Alex and Lyndon Learn Math & Numbers for the School Exam | Fun Kids Videos
@Numberblocks - Cool Maths Games | Learn to Count
Read Anyone's Mind With This EASY Math Trick
10 Math Tricks School Didn't Teach You But Should Have
Комментарии
 0:22:09
0:22:09
 0:22:32
0:22:32
 0:00:16
0:00:16
 0:00:16
0:00:16
 0:00:28
0:00:28
 0:00:58
0:00:58
 0:04:05
0:04:05
 0:19:29
0:19:29
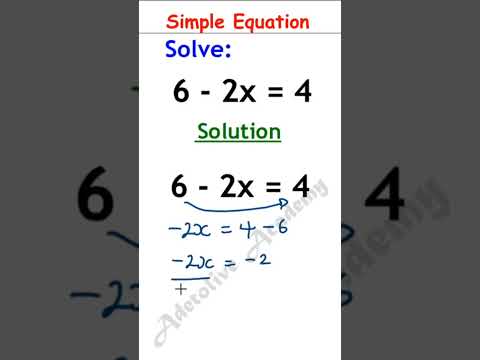 0:00:22
0:00:22
 0:00:12
0:00:12
 0:31:33
0:31:33
 0:01:54
0:01:54
 0:01:59
0:01:59
 0:14:03
0:14:03
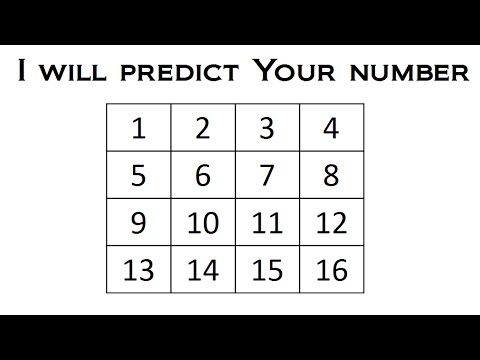 0:02:50
0:02:50
 0:19:31
0:19:31
 0:03:15
0:03:15
 0:03:46
0:03:46
 0:33:06
0:33:06
 0:00:23
0:00:23
 0:15:05
0:15:05
 0:14:54
0:14:54
 0:02:45
0:02:45
 0:10:41
0:10:41