UW Combinatorics Seminar: Florian Frick -- October 7, 2020

Title: The topological Tverberg problem beyond prime powers
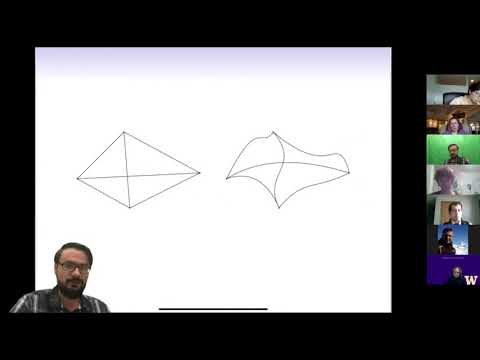
Abstract: Given d and q the topological Tverberg problem asks for the minimal n such that any continuous map from the n-dimensional simplex to ℝd identifies q points from pairwise disjoint faces. For q a prime power n is (q−1)(d+1). The lower bound follows from a general position argument, the upper bound from equivariant topological methods. It was shown recently that for q with at least two distinct prime divisors the lower bound may be improved. For those q non-trivial upper bounds had been elusive. I will show that n is at most q(d+1)−1 for all q. I had previously conjectured this to be optimal unless q is a prime power. This is joint work with Pablo Soberón.