What does Fraction Division Really Mean?

Are you struggling to understand what fraction division really means? Do you find yourself getting lost in the math jargon? If so, then this video is for you!

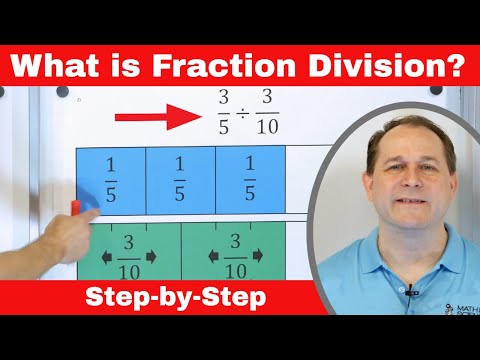
In this tutorial, we will break down the concept of fraction division into simple terms that anyone can understand. We will start with the basics of fractions and how they are represented. Then, we will move on to division and how it applies to fractions.

You will learn the key terms and definitions that are essential to understanding fraction division. We will also go through a few examples to help illustrate the concept and show you how to solve fraction division problems step-by-step.

This video is perfect for anyone who is struggling with fractions, whether you are a student in school or just someone who wants to brush up on your math skills. By the end of this video, you will have a solid understanding of what fraction division really means and how to solve problems on your own.

So, whether you're new to fractions or just need a refresher, be sure to check out this video and improve your math skills today!

[AnshuRaj-uhyd]

Sir I am from India and I like your explanation but it's my humble request that please explain Quadratic equation and linear inequation

[fortyacres]

For examples that do not result in a whole number, you could use strip diagrams and split the different-sized parts so that they are the same. This leads to an interesting discussion because many students are told that you do not need to find a common denominator when dividing fractions. However, the 'invert and multiply' or 'cross multiply' methods both result in the answer using the numerators of the from the equivalent fractions if you had found a common denominator. If students see this before the method or 'trick, ' then the 'invert and multiply' method makes more sense.

for example: (2/3) ÷ (4/5)

in the strip diagram: cut the the thirds into fifths. cut the fifths into thirds. then, it is a problem of fitting 12 parts in to 10. This is just 10/12

common denominator: (2/3) ÷ (4/5) can be written as (10/15) ÷ (12/15). This is fitting 12 parts of the same size (fifteenths) into 10 parts of the same size (fifteenths). (the size of the common part does really matter)

invert and multiply: (2/3) ÷ (4/5) = (2/3) x (5/4) = 10/12. The two parts from 2/3 get split into fifths, producing 10 (2x5). The 4 parts from 4/5 get split into thirds, producing 12 (4x3). At the end of the day, the 10 is the numerator on the equivalent fraction for 2/3 and the 12 is the numerator on the equivalent fraction for 4/5.

The same strip diagram applies to all of these.

Interesting observation: Some students will say the answer to (2/3 ) ÷ (4/5) is 'zero' because 4/5 does not fit into 2/3 if the question is 'how many times 4/5 goes into 2/3?' Of course, the good teacher will reply: Correct, the whole 4/5 will not fit. However, what fraction of 4/5 will fit?

thanks for the videos.

[simpleman]

Perfect example of what this is & how it works.

[dheeru]

Thanks a lot sir I'm grateful to you i appreciate you..

[Njitagwo_Shiro]

How do I make sure the boundaries alignn?

[realrider]

Sir how to exactly start preparations for Electrical course like electronics, electrical machines, circuit theory and all?

[rizeandrealize]

You are a great teacher. Your explanation is always easy to follow. Thank you, Sir ❤

[naderhumood]

Great professor...Thank you very much indeed. ❤

[hemrajue]

Picture division of fractions always won't give us a whole number. Moreover, we won't get an accurate answer, if picture divisions not aligned properly.. What, if we build the common denominator and go for the picture division?

[adminbabakov]

Thank you. I am from Tatarstan . Respect you