filmov
tv
Linear Programming 3: Graphical Solution – with negative coefficients

Показать описание
This video shows how to graphically solve a maximization LP model that has
1) constraints with negative coefficients
2) fractional plotting points
LP model Solved:
Max -3X + 12Y
s.t.
7X - 1Y ≤ 3
-3X + 6Y ≤ 10
3X + 4Y ≤ 9
3X + 3Y ≥ 3
X, Y ≥ 0
Linear Programming 1: Graphical Solution -Extreme/Corner Points
Linear Programming 2: Graphical Solution - Minimization
Linear Programming 4: Slack/Surplus, Binding Constraints, Standard Form
Linear Programming 5: Alternate solutions, Infeasibility, Unboundedness, & Redundancy
1) constraints with negative coefficients
2) fractional plotting points
LP model Solved:
Max -3X + 12Y
s.t.
7X - 1Y ≤ 3
-3X + 6Y ≤ 10
3X + 4Y ≤ 9
3X + 3Y ≥ 3
X, Y ≥ 0
Linear Programming 1: Graphical Solution -Extreme/Corner Points
Linear Programming 2: Graphical Solution - Minimization
Linear Programming 4: Slack/Surplus, Binding Constraints, Standard Form
Linear Programming 5: Alternate solutions, Infeasibility, Unboundedness, & Redundancy
Linear Programming 3: Graphical Solution – with negative coefficients
LP Graphical Method (Multiple/Alternative Optimal Solutions)
Linear Programming 2: Graphical Solution - Minimization Problem
Linear Programming 1: Maximization -Extreme/Corner Points (LP)
How to Solve a Linear Programming Problem Using the Graphical Method
Linear Programming - Graphical Solution | Don't Memorise
Linear Programming 5: Alternate solutions, Infeasibility, Unboundedness, & Redundancy
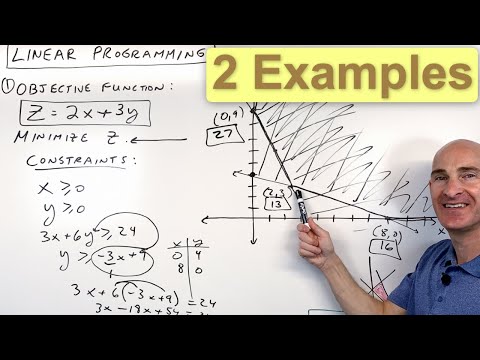
Linear Programming (Optimization) 2 Examples Minimize & Maximize
[Part 3] Graphical Solution Method to LPs
Linear Programming
Minimize LPP using Graphical Solution | Linear Programming Problem | Operational Research
Graphical Solution of Simultaneous Linear Equations
Solving Linear Programming Minimization Problem using Graphical Method
Linear Programming Problem (Minimize) - Graphical solution of LPP
Inequalities: Region on Graph
Tutorial for Linear Programming 2 - Graphical Solution.mp4
LPP Graphical Method - 4 Negative sign in constraints and objective function
MS: Linear Programming Excel Solutions Part 4: Modeling and Graphical Solutions (Minimization LP)
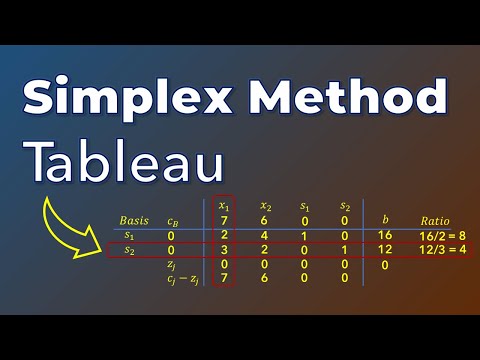
Intro to Simplex Method | Solve LP | Simplex Tableau
Minimization of Graphical Solution With Different Inequalities in LPP #5
Lec-1 Graphical Method | Linear Programming Problem | Unique Optimal Solution | Operations Research
LPP - Graphical Method [#2] [Minimization & Maximization with 3 Constraints] :-by kauserwise
Linear Programming - Graphical Solution Example
Linear Programming, Lecture 1. Introduction, simple models, graphic solution
Комментарии
 0:05:52
0:05:52
 0:05:27
0:05:27
 0:04:48
0:04:48
 0:05:43
0:05:43
 0:11:49
0:11:49
 0:07:16
0:07:16
 0:03:43
0:03:43
 0:15:08
0:15:08
![[Part 3] Graphical](https://i.ytimg.com/vi/-OnRFdLTTlE/hqdefault.jpg) 0:07:39
0:07:39
 0:33:20
0:33:20
 0:18:07
0:18:07
 0:09:39
0:09:39
 0:10:23
0:10:23
 0:03:13
0:03:13
 0:07:20
0:07:20
 0:01:14
0:01:14
 0:10:59
0:10:59
 0:23:35
0:23:35
 0:12:40
0:12:40
 0:09:10
0:09:10
 0:17:50
0:17:50
 0:20:03
0:20:03
 0:09:57
0:09:57
 1:14:55
1:14:55