filmov
tv
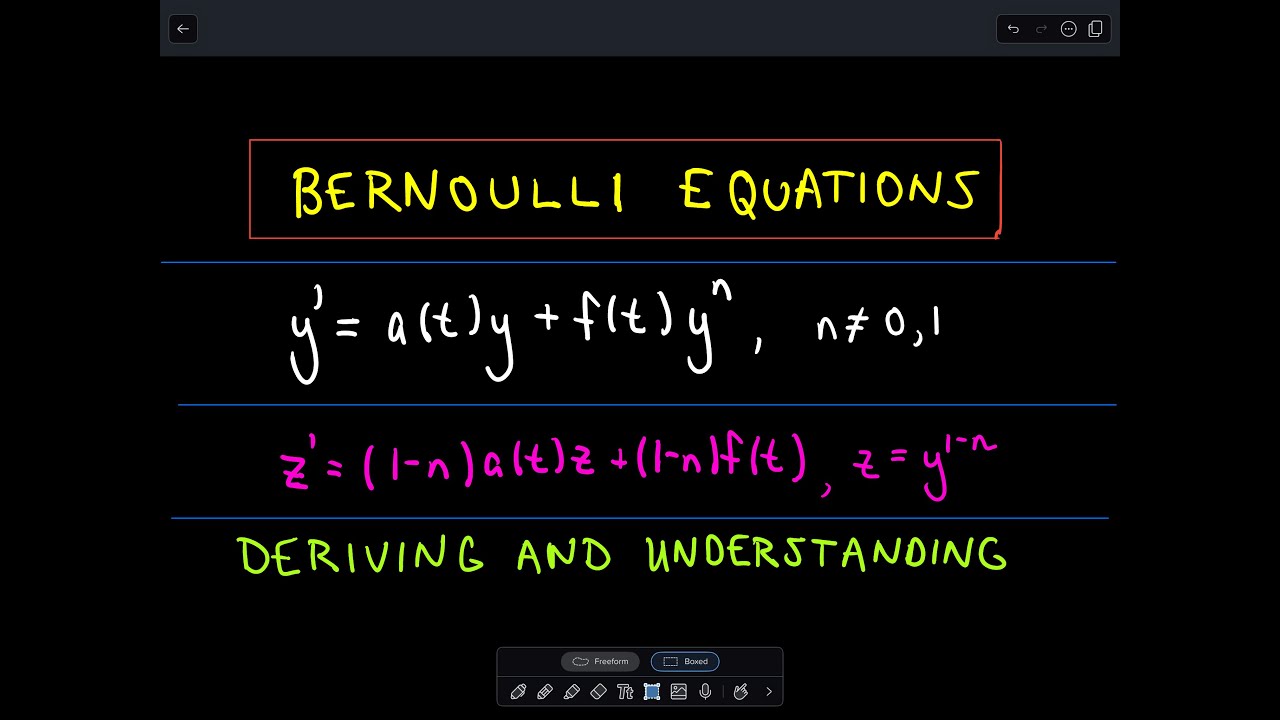
❖ Bernoulli Equation for Differential Equations , Part 1 ❖
Показать описание
In this video, I show how that by using a change of variable it is possible to make some equations into linear differential equations which we can then solve using an integrating factor.
This video show WHY this process works; if you are looking for a concrete example, check out the next part.
In the second video I solve a Bernoulli Equation by going through the complete process.
This video would be suitable for a student learning differential equations and who wanted to see the full derivation and why it works.
This video show WHY this process works; if you are looking for a concrete example, check out the next part.
In the second video I solve a Bernoulli Equation by going through the complete process.
This video would be suitable for a student learning differential equations and who wanted to see the full derivation and why it works.
The Bernoulli Equation // Substitutions in Differential Equations
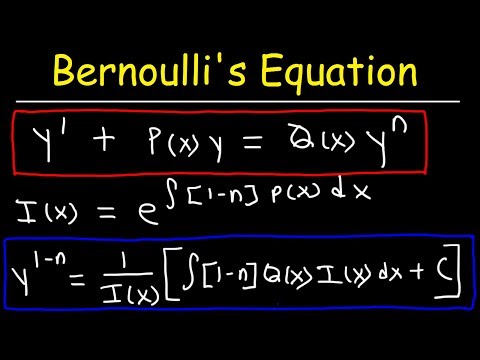
Bernoulli's Equation For Differential Equations
Bernoulli Differential Equations: Solution Methods and Exercises
How to Solve Bernoulli Differential Equations (Differential Equations 23)
How to solve Bernoulli differential equations
🔵16 - Bernoulli Differential Equations (dy/dx + p(x)y = q(x)y^n)
❖ Bernoulli Equation for Differential Equations , Part 1 ❖
Bernoulli's Equation in Differential Equation Solved Problems - Differential Equation
Bernoulli Differential Equations
Bernoulli's Equation
How to solve differential equations
Bernoulli Differential Equation (with a missing solution)
Bernoulli's Principle Explained (Differential Equation)
Bernoulli’s Differential Equations Part 1 (Live Stream)
Bernoulli's Differential Equation | Reducible to linear Differential Equation | Problem 1 | Mat...
Bernoulli Differential Equation dy/dx = y(xy^2 - 1)
Bernoulli First Order Equations - Intro
Bernoulli First Order Equations - Example 1
Diferansiyel Denklemler : Bernoulli Diferansiyel Denklemi (www.buders.com)
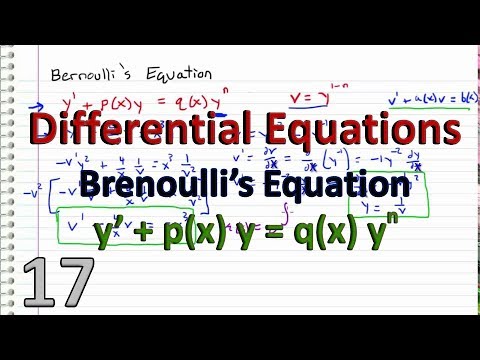
Differential Equations - 17 - Bernoulli's Equation
Bernoulli's Differential Equation | How to solve Bernoulli Differential Equation in urdu-ODE
❖ Bernoulli Equation for Differential Equations , Part 2 ❖
BERNOULLI'S EQUATION for DIFFERENTIAL EQUATIONS | Integrating Factor | TAGALOG-ENGLISH
BERNOULLI EQUATION || How to reduce it into the linear differential equation ?? || Mathematics ||
Комментарии
 0:09:19
0:09:19
 0:20:03
0:20:03
 0:11:16
0:11:16
 1:43:35
1:43:35
 0:05:30
0:05:30
 0:28:21
0:28:21
 0:10:26
0:10:26
 0:33:19
0:33:19
 0:09:27
0:09:27
 0:08:22
0:08:22
 0:00:46
0:00:46
 0:09:26
0:09:26
 0:02:03
0:02:03
 1:27:06
1:27:06
 0:10:35
0:10:35
 0:14:22
0:14:22
 0:10:04
0:10:04
 0:04:50
0:04:50
 0:14:33
0:14:33
 0:07:39
0:07:39
 0:13:20
0:13:20
 0:10:55
0:10:55
 0:35:48
0:35:48
 0:07:26
0:07:26