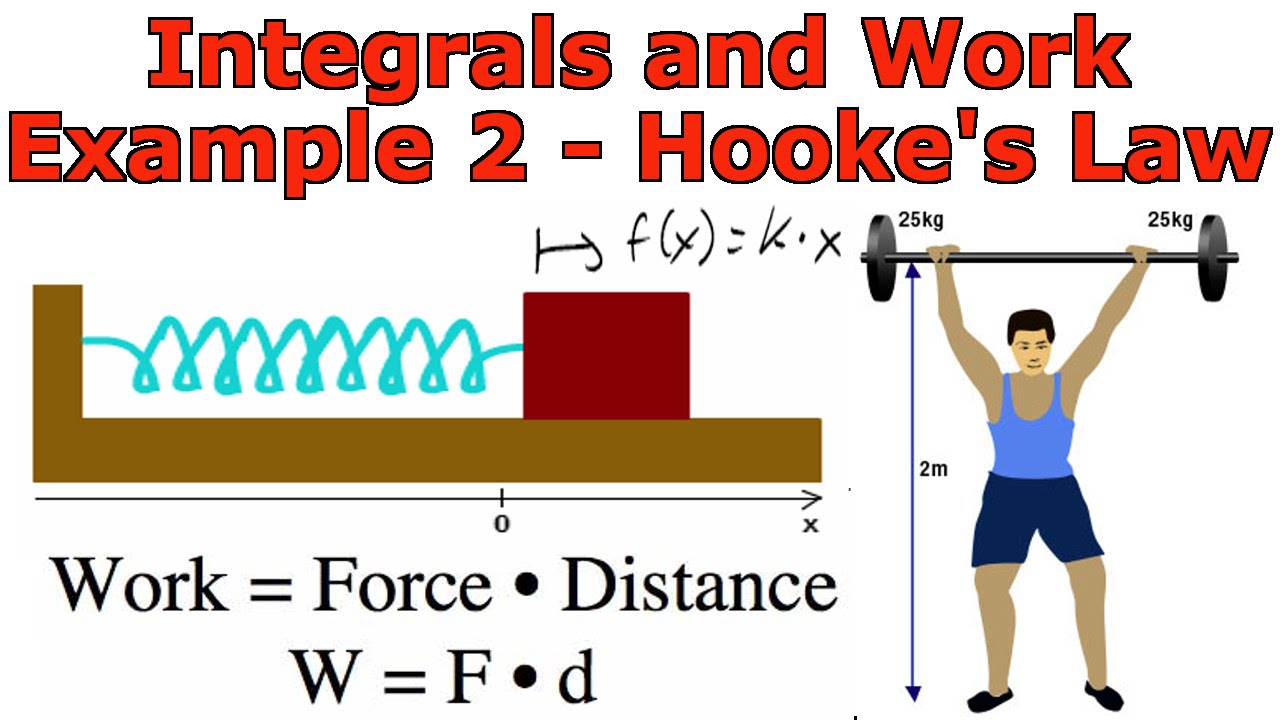
Integrals and Work: Example 2 - Hooke's Law

In this video I go over another example on work and this time deal with Hooke's Law. Hooke's Law states that the force required to maintain a spring stretched x units beyond its natural length is proportional to x and can be written as f(x) = kx where k is a positive constant and is known as the spring constant. The example that I cover is an example involving stretching a spring and determining the amount of work that was required.

Related Videos:

------------------------------------------------------

[mes]

I don't always determine the amount of work required to stretch a spring but when I do usually use Hooke's Law ;)

[Tony-nlpf]

Didn't need to be 10 minutes long but 30 seconds of skipping through helped so thank you.