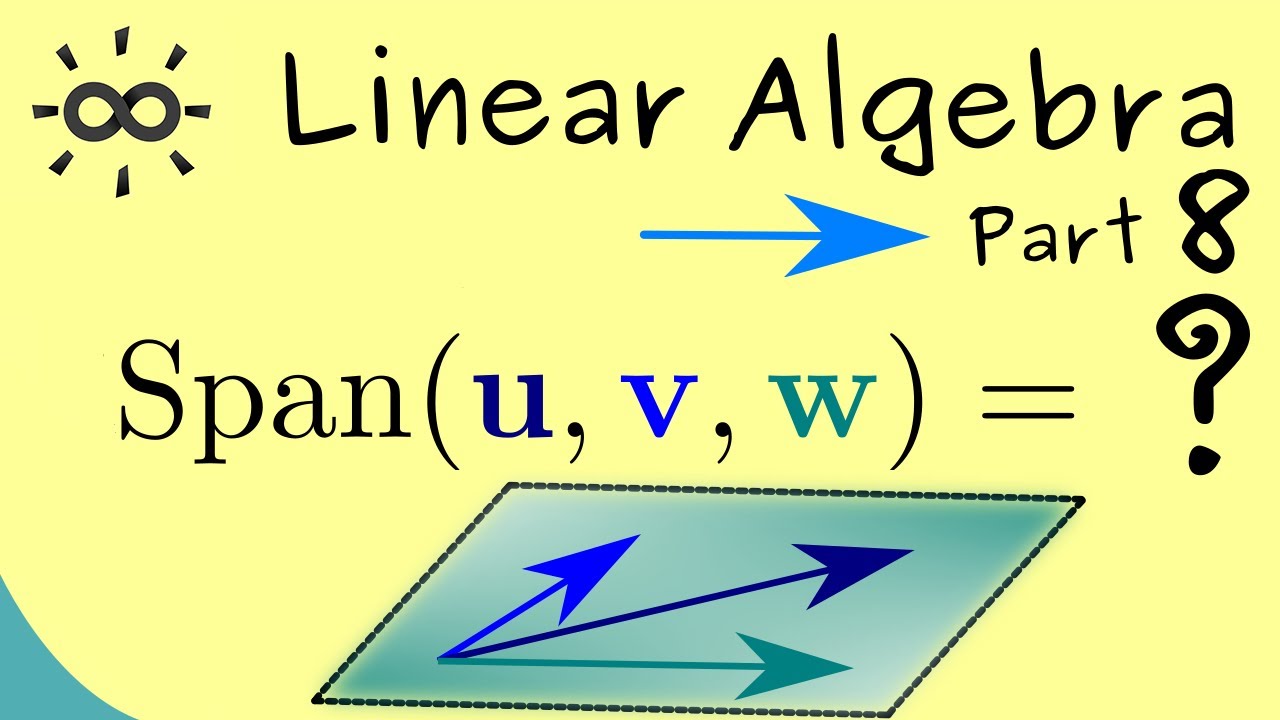
Linear Algebra 8 | Linear Span

Thanks to all supporters! They are mentioned in the credits of the video :)

This is my video series about Linear Algebra. I hope that it will help everyone who wants to learn about it.

#LinearAlgebra
#Vectors
#Matrices
#MachineLearning
#Eigenvalues
#Calculus
#Mathematics
x

(This explanation fits to lectures for students in their first year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)

[cagi]

A wonderful and intuituve explanation of such concepts. As an engineering student who is curious about mathematics I am glad that you decided to dedicate a playlist for linear algebra. I hope you post more advanced topics about it soon:)

[zyugyzarc]

This explanation makes a LOT more sense - that span(M) is a subspace in R^n

[punditgi]

Glad to see another one of your videos! 😃

[kirikouestpetit]

Thank you so much for these ! A friend recommended your channel to me last summer and I've been binging your videos ever since. I started a college math heavy course as someone who used to be terrified of maths. You and some other channels made me love them :-) I am so grateful and someday I hope to be able to contribute a bigger amount to your channel monthly.

Maybe I missed them but do you ever plan on covering dedekind cuts ? We're studying them right now, along with "ideal polynomials" (if that is the term in English...), would be curious to see your take on it. :-)


Great work !❤

[gmpkcpz]

Why haven't I discovered this cannel before ? vielen danke

[GeoffryGifari]

is it possible to assign equality to span{ }? from your example of span{ (1 0 0), (1 1 0)} being the entire XY plane, i imagine span{(1/2 0 0), (0 1/2 0)} is also the XY plane, so many sets of vectors can span a set equally

[RiseUp]

which software do you use bro, for explaining

[GeoffryGifari]

and also, is it *not* possible to find the *largest* scalar with which we can multiply the vectors of set M when we're making span{M} ? (so that one ray built from one of our vectors don't extend forever)

i see that if this is the case its not possible to have a span inside a closed, finite area in R² for example (like the blob drawn early in the video)