filmov
tv
Jacobian Matrix and Singularities | Robotics | Introduction | Part 1
Показать описание
Jacobian Matrix and Singularities | Robotics | Introduction | Part 1
In this video we will run through an introduction to using and finding the #Jacobian Matrix for #robotic systems. The Jacobian can be used to find:
1) Joint Velocities
2) Singularities
3) Torques and Forces on a robot's joint
--------------------------------------------------------------------------------------------------------------------
Intro music:
Happy Rock
----------------------------------------------------------------------------------------------------------------------
Subject material is obtained from:
John. J. Craig, Introduction to Robotics (Mechanics and Control). Pearson Education International.
-----------------------------------------------------------------------------------------------------------------------
Thumbs up and Subscribe Icons are made by
-----------------------------------------------------------------------------------------------------------------------
Hope this video helps.
If you have any questions, please leave a comment, and don't forget to like and subscribe.
Cheers everyone!
In this video we will run through an introduction to using and finding the #Jacobian Matrix for #robotic systems. The Jacobian can be used to find:
1) Joint Velocities
2) Singularities
3) Torques and Forces on a robot's joint
--------------------------------------------------------------------------------------------------------------------
Intro music:
Happy Rock
----------------------------------------------------------------------------------------------------------------------
Subject material is obtained from:
John. J. Craig, Introduction to Robotics (Mechanics and Control). Pearson Education International.
-----------------------------------------------------------------------------------------------------------------------
Thumbs up and Subscribe Icons are made by
-----------------------------------------------------------------------------------------------------------------------
Hope this video helps.
If you have any questions, please leave a comment, and don't forget to like and subscribe.
Cheers everyone!
Комментарии
 0:08:42
0:08:42
 0:20:21
0:20:21
 0:06:37
0:06:37
 0:13:49
0:13:49
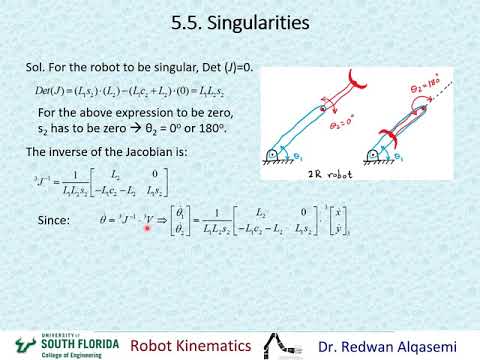 0:13:59
0:13:59
 0:11:09
0:11:09
 0:55:14
0:55:14
 0:15:24
0:15:24
 0:12:32
0:12:32
 0:09:58
0:09:58
 0:09:07
0:09:07
 0:58:22
0:58:22
 0:16:12
0:16:12
 0:05:45
0:05:45
 0:16:41
0:16:41
 0:25:02
0:25:02
 0:58:22
0:58:22
 0:03:52
0:03:52
 0:01:31
0:01:31
 0:52:02
0:52:02
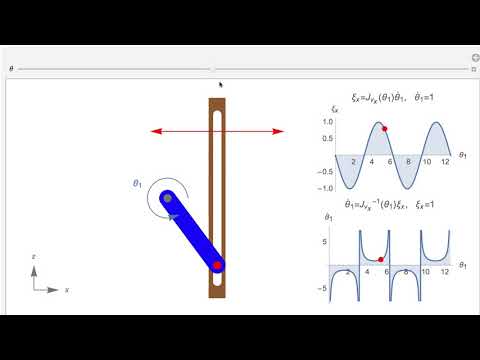 0:19:12
0:19:12
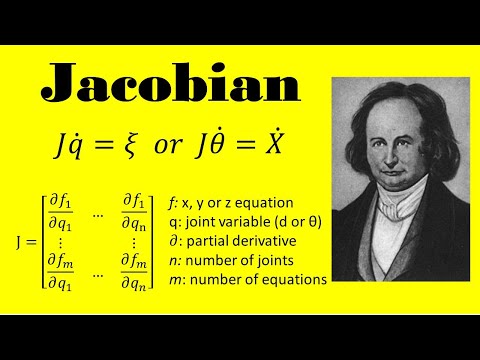 0:11:21
0:11:21
 0:07:33
0:07:33
 0:46:10
0:46:10