filmov
tv
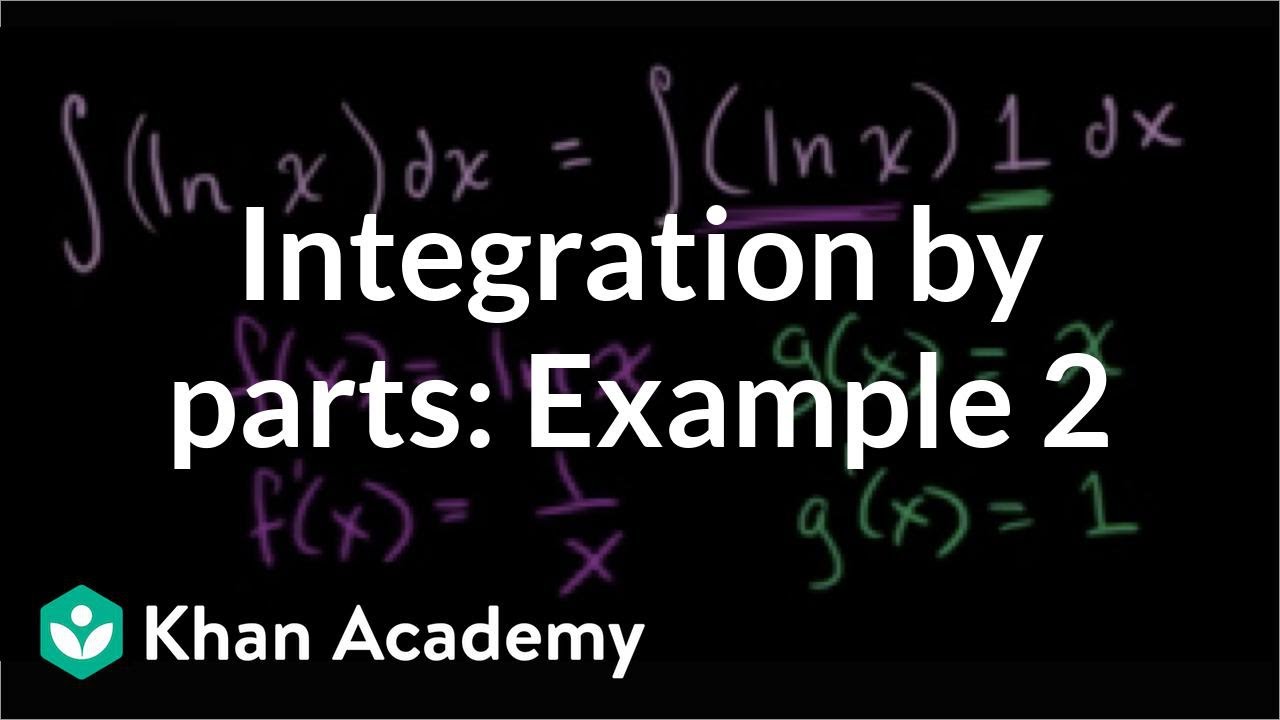
Integration by parts: ºln(x)dx | AP Calculus BC | Khan Academy
Показать описание
Worked example of finding an indefinite integral using integration by parts, where the integrand isn't a product. Created by Sal Khan.
AP Calculus BC on Khan Academy: Learn AP Calculus BC - everything from AP Calculus AB plus a few extra goodies, such as Taylor series, to prepare you for the AP Test
For free. For everyone. Forever. #YouCanLearnAnything
Integration by parts: ºln(x)dx | AP Calculus BC | Khan Academy
Integration by Parts the Integral of xlnx
Integration by Parts: Example including ln(x)
Integration By Parts Simple Problem X ln X dx
AQA Core 3 7.04d Integration by Parts: Integrating ln(x)
Integration by Parts x^2 ln x AP Calculus
Integration by parts of (x lnx)dx
Integrate x ln x dx; u = lnx, dv = x dx
Integral of ln(x)/x^2, integration by parts, DI method
Integration by Parts The Integral of lnx
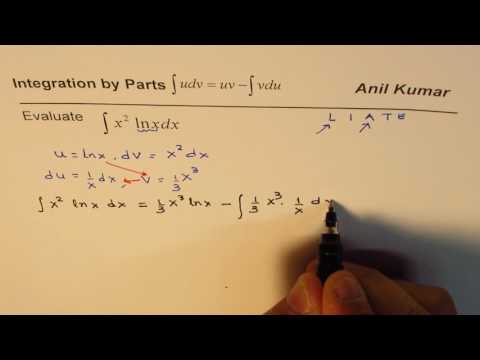
How to integrate x^2lnx using Integration by Parts
Integration by Parts - Example 3: ln x
Integration by Parts Ln x
Integration by parts: ºx_cos(x)dx | AP Calculus BC | Khan Academy
Integral of (ln x)^2 || Integration by parts
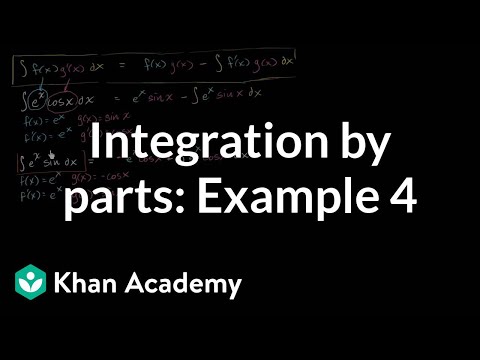
integration by parts x^2e^x
Integration by Parts: Example 2: ln(x)
Integration by Parts
How to integrate ln x (Integration by Parts)
Integration by parts: º___cos(x)dx | AP Calculus BC | Khan Academy
Integral of lnx/x
Integration by Parts for (ln x)^2 by Traditional Method | Integration | Calculus | Glass of Numbers
Integral of (lnx)^2
Integration by parts xln(x)
Комментарии
 0:03:50
0:03:50
 0:02:07
0:02:07
 0:04:00
0:04:00
 0:04:50
0:04:50
 0:02:59
0:02:59
 0:04:05
0:04:05
 0:04:24
0:04:24
 0:01:03
0:01:03
 0:03:56
0:03:56
 0:01:24
0:01:24
 0:02:53
0:02:53
 0:02:11
0:02:11
 0:04:22
0:04:22
 0:03:52
0:03:52
 0:04:27
0:04:27
 0:04:55
0:04:55
 0:03:51
0:03:51
 0:09:36
0:09:36
 0:01:39
0:01:39
 0:07:16
0:07:16
 0:02:35
0:02:35
 0:07:15
0:07:15
 0:03:42
0:03:42
 0:06:59
0:06:59