Davide Spriano, Hyperbolic models for CAT(0) spaces

A very successful approach in geometric group theory is to construct
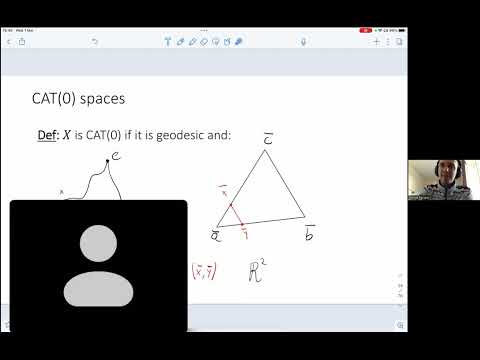
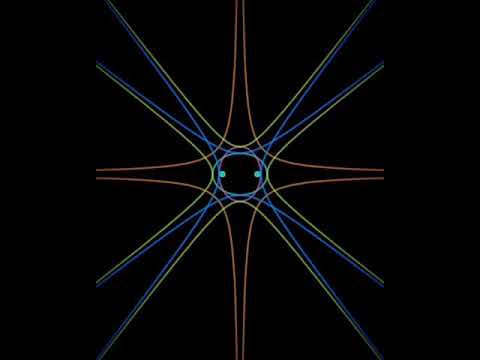
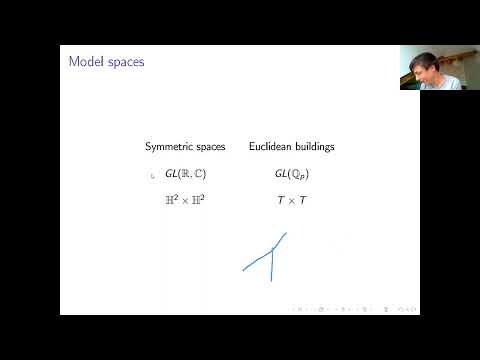
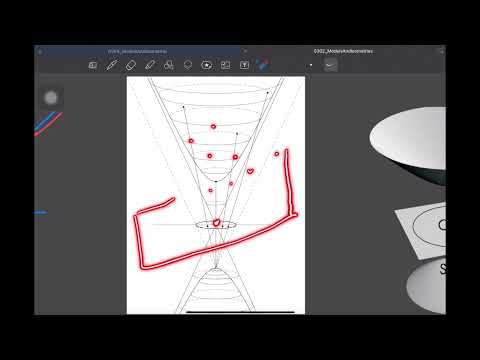
hyperbolic models'' for interesting groups, namely a hyperbolic space on which a (non-hyperbolic) group acts in a nice enough way. The earliest example of this philosophy is the Bass-Serre, and other more recent examples include the curve graph for mapping class groups, contact graph for cubical groups, free factor/free splitting/cyclic splitting complex for Out(F_n) and so on. In this talk we will describe the curtain model, a hyperbolic space on which CAT(0) groups act, and discuss several results about it. This is joint work with H. Petyt and A. Zalloum.