Eduard Einstein: Relatively Hyperbolic Groups and their Actions on CAT(0) Cube Complexes

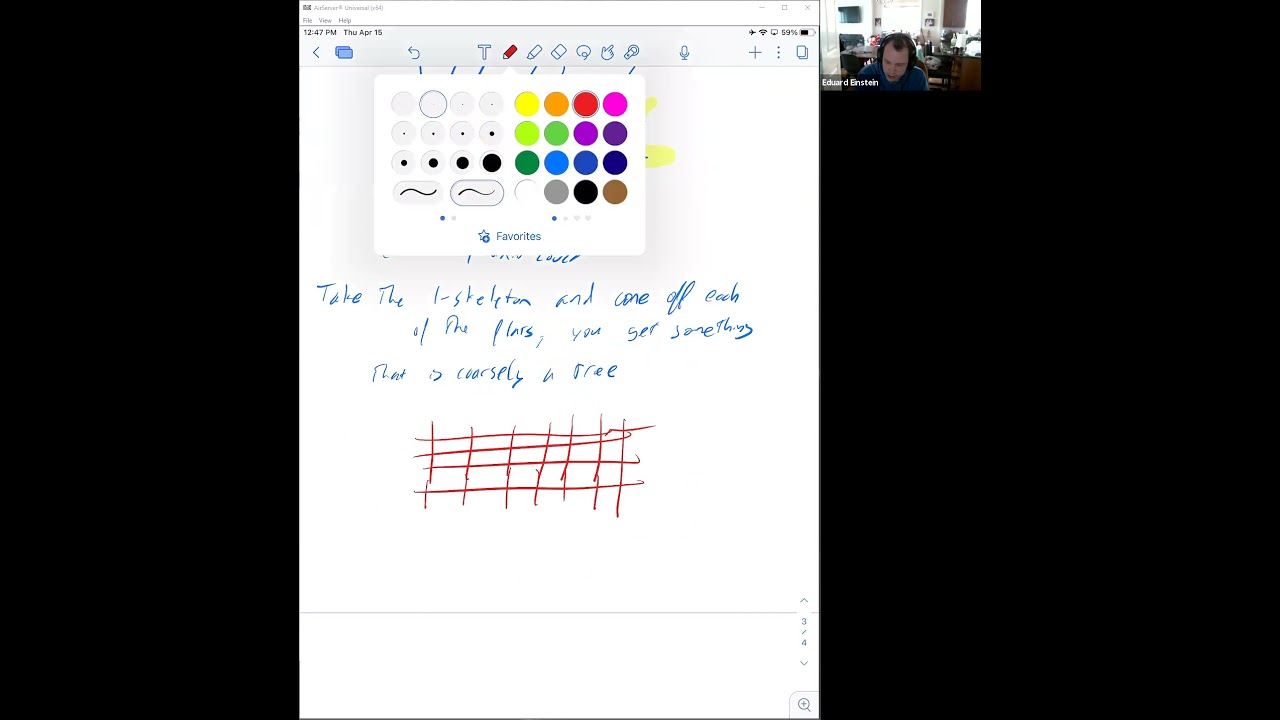
Relatively hyperbolic groups, first introduced by Gromov, generalize hyperbolic groups and have natural applications. For example, the fundamental group of a finite volume hyperbolic 3-manifold is hyperbolic relative to the cusp subgroups. In this introductory talk, I will discuss some of the many equivalent formulations of relative hyperbolicity and the relationship between a relatively hyperbolic group and its Bowditch boundary. I will also introduce CAT(0) cube complexes and give a brief survey of efforts to extend the machinery developed by Agol, Wise and others for cubical hyperbolic groups to relatively hyperbolic groups that act nicely on a CAT(0) cube complex.

Note: The first minute of the talk was not recorded, sorry about that.