filmov
tv
Right-angled Coxeter groups and affine actions (Lecture 02) by Francois Gueritaud

Показать описание
DISCUSSION MEETING
SURFACE GROUP REPRESENTATIONS AND PROJECTIVE STRUCTURES
ORGANIZERS: Krishnendu Gongopadhyay, Subhojoy Gupta, Francois Labourie, Mahan Mj and Pranab Sardar
DATE: 10 December 2018 to 21 December 2018
VENUE: Ramanujan Lecture Hall, ICTS Bangalore
The study of spaces of complex structures on a Riemann surface, the so-called Moduli space and Teichmüller space is a classical and well-studied area of mathematics, with relations and interconnections with different areas of mathematics and also theoretical physics. In the case of surfaces with genus at least two, complex structures can be uniformized to hyperbolic structures, which are discrete, faithful representations of surface groups in the group of isometries of the hyperbolic plane. A natural generalization is to consider surface group representations in other semisimple Lie groups. In the last few years, spectacular advances have been made towards generalizing existing tools and techniques to the study of these representations, and their moduli spaces. Remarkably, in many cases there is a natural generalization of discrete, faithful representations which provides an analogue of Teichmüller space. Our program shall focus on two perspectives:
1.The dynamic point of view, leading to Anosov surface group representations (in the sense of Labourie).
2.The complex geometric point of view coming from complex projective structures on surfaces.
The program may be thought of as a focused follow-up of two programs held at ICTS in November 2017:
1.Geometry, groups and dynamics (GGD-2017)
2.Surface group representations and geometric structures (SGGS)
While the 2017 program had some introductory talks on the subject of “Higher Teichmuller theory” (also known as the theory of surface group representations in semi-simple Lie groups of higher rank), the purpose of the present program is to take the subject further.
We expect that a number of the participants of the former program (GGD and SGGS), particularly some of the younger participants, will be focusing on this area in their research. The follow-up program would have these researchers as the target audience. The meeting will thus be aimed at a specialised audience, and only focused researchers and scholars of these areas with prior background in these subjects will be included.
0:00:00 Surface Group Representations and Projective Structures
0:00:10 Right-angled Coxeter groups and affine actions (Lecture 02)
0:00:51 Reminder
0:03:48 Theorem I
0:07:25 Theorem II
0:10:14 Theorem III
0:20:52 Recall
0:21:39 Idea of proof of III
0:27:27 Application of II: Theorem
0:28:26 Proof
0:31:18 Quasi fuchsian groups
0:36:11 Property
0:37:52 Wedge Deletion
0:45:06 Lipschitz extension theorem
0:48:58 Coxeter groups
0:52:40 Remark
1:03:06 Theorem (Vinberg)
1:06:47 Example
1:10:09 Theorem (Danciger-G-Kassel)
SURFACE GROUP REPRESENTATIONS AND PROJECTIVE STRUCTURES
ORGANIZERS: Krishnendu Gongopadhyay, Subhojoy Gupta, Francois Labourie, Mahan Mj and Pranab Sardar
DATE: 10 December 2018 to 21 December 2018
VENUE: Ramanujan Lecture Hall, ICTS Bangalore
The study of spaces of complex structures on a Riemann surface, the so-called Moduli space and Teichmüller space is a classical and well-studied area of mathematics, with relations and interconnections with different areas of mathematics and also theoretical physics. In the case of surfaces with genus at least two, complex structures can be uniformized to hyperbolic structures, which are discrete, faithful representations of surface groups in the group of isometries of the hyperbolic plane. A natural generalization is to consider surface group representations in other semisimple Lie groups. In the last few years, spectacular advances have been made towards generalizing existing tools and techniques to the study of these representations, and their moduli spaces. Remarkably, in many cases there is a natural generalization of discrete, faithful representations which provides an analogue of Teichmüller space. Our program shall focus on two perspectives:
1.The dynamic point of view, leading to Anosov surface group representations (in the sense of Labourie).
2.The complex geometric point of view coming from complex projective structures on surfaces.
The program may be thought of as a focused follow-up of two programs held at ICTS in November 2017:
1.Geometry, groups and dynamics (GGD-2017)
2.Surface group representations and geometric structures (SGGS)
While the 2017 program had some introductory talks on the subject of “Higher Teichmuller theory” (also known as the theory of surface group representations in semi-simple Lie groups of higher rank), the purpose of the present program is to take the subject further.
We expect that a number of the participants of the former program (GGD and SGGS), particularly some of the younger participants, will be focusing on this area in their research. The follow-up program would have these researchers as the target audience. The meeting will thus be aimed at a specialised audience, and only focused researchers and scholars of these areas with prior background in these subjects will be included.
0:00:00 Surface Group Representations and Projective Structures
0:00:10 Right-angled Coxeter groups and affine actions (Lecture 02)
0:00:51 Reminder
0:03:48 Theorem I
0:07:25 Theorem II
0:10:14 Theorem III
0:20:52 Recall
0:21:39 Idea of proof of III
0:27:27 Application of II: Theorem
0:28:26 Proof
0:31:18 Quasi fuchsian groups
0:36:11 Property
0:37:52 Wedge Deletion
0:45:06 Lipschitz extension theorem
0:48:58 Coxeter groups
0:52:40 Remark
1:03:06 Theorem (Vinberg)
1:06:47 Example
1:10:09 Theorem (Danciger-G-Kassel)
 1:14:29
1:14:29
 1:16:02
1:16:02
 1:01:40
1:01:40
 1:19:32
1:19:32
 0:37:16
0:37:16
 0:13:01
0:13:01
 0:55:37
0:55:37
 0:26:02
0:26:02
 0:48:09
0:48:09
 0:41:35
0:41:35
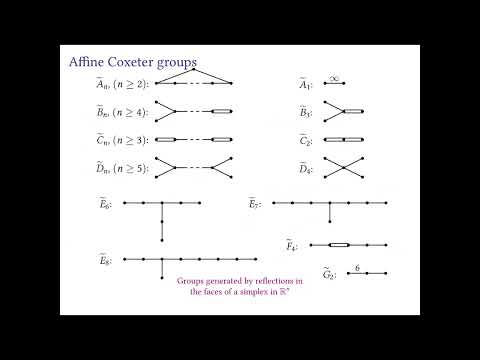 0:24:04
0:24:04
 0:51:16
0:51:16
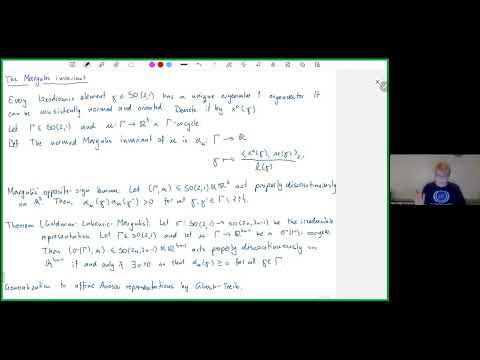 0:22:02
0:22:02
 0:50:12
0:50:12
 0:36:07
0:36:07
 1:00:34
1:00:34
 0:47:16
0:47:16
 1:15:38
1:15:38
 0:44:40
0:44:40
 0:42:20
0:42:20
 0:31:34
0:31:34
 0:55:49
0:55:49
 0:44:33
0:44:33
 0:34:23
0:34:23