Find the Length of the Chord | Important Geometry Skills Explained

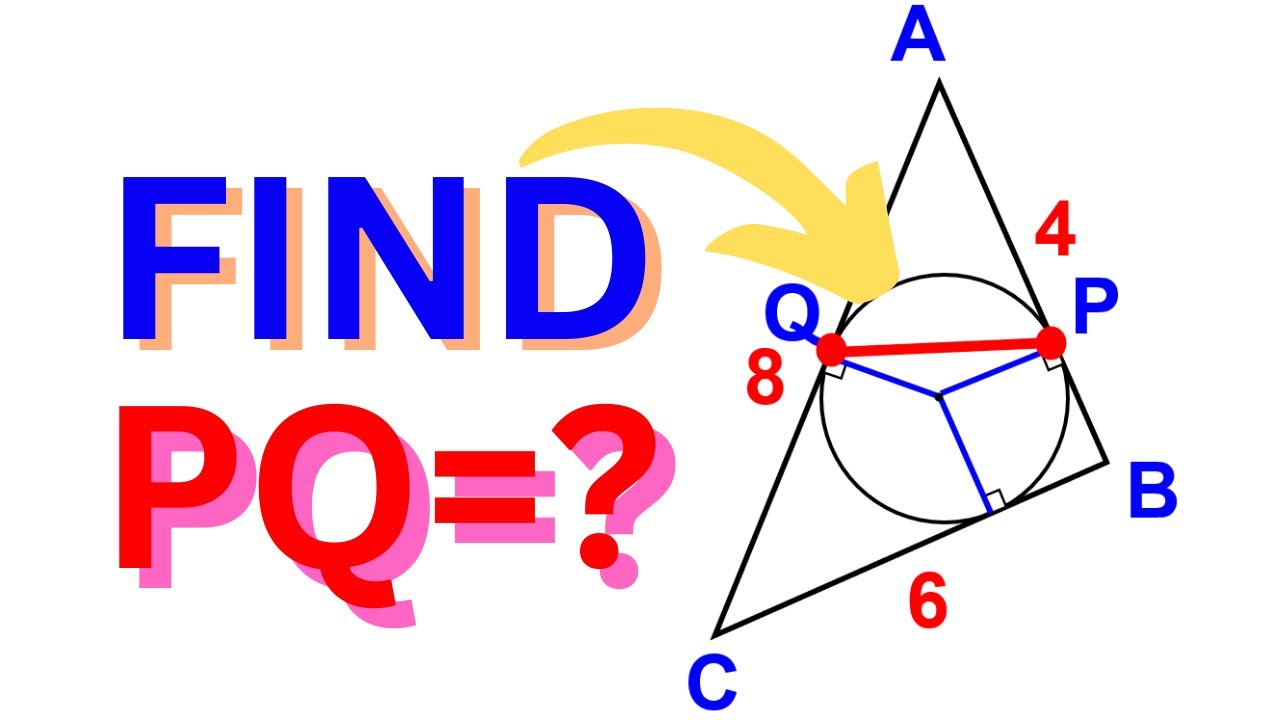
A circle is inscribed in Triangle ABC with sides AB = 4, BC = 6, and
AC = 8. If P and Q are the respective points of tangency of AB and
AC with the circle, determine the length of chord PQ.

In this video, we'll be solving problems involving trigonometry, algebra, circles, and geometry. We'll be using the congruence of triangles, the solving systems of linear equations, and the Law of Cosines.

If you're looking to improve your trigonometry, algebra, geometry, or circle skills, then this video is for you! By the end of this video, you'll be able to solve problems faster and more effectively than ever before.

Please like and watch the video until the end and let us learn together.

[GrayYeonMath]

Sorry for typo error the given is A+C = 8.

I copied equation 3 wrong. I'm really sorry.

I just only solved for A because it is what we only need for PQ.

a+b=4

since we calculated a = 3, then b = 4-a, b = 1

and since a+c = 8 then c=8-a, c=8-3 = 5, c=5

a=3, b=1, c=5

5:34 here are the correct set of system of equations.

[bradylawrence]

I’m slightly confused at how a=3, if b+c=6 and a+c=6, then b and a should be equal, but a=3 and b=3 doesn’t satisfy a+b=4