filmov
tv
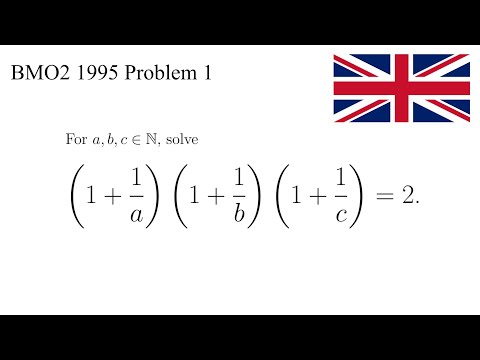
Symmetric Diophantine Equation | British Mathematical Olympiad 1995 Problem 1

Показать описание
#DiophantineEquations #NumberTheory #MathOlympiad
Here is the solution to BMO2 1995 Problem 1!!
————————————————————————————————————————————————
Follow my Instagram page for more Maths :)
————————————————————————————————————————————————
I share Maths problems and Maths topics from well-known contests, exams and also from viewers around the world. Apart from sharing solutions to these problems, I also share my intuitions and first thoughts when I tried to solve these problems.
Here is the solution to BMO2 1995 Problem 1!!
————————————————————————————————————————————————
Follow my Instagram page for more Maths :)
————————————————————————————————————————————————
I share Maths problems and Maths topics from well-known contests, exams and also from viewers around the world. Apart from sharing solutions to these problems, I also share my intuitions and first thoughts when I tried to solve these problems.
Symmetric Diophantine Equation | British Mathematical Olympiad 1995 Problem 1
Symmetric Diophantine Equations & Divisibility | IMO SL 2019 Problem 2 | Number Theory | Cheenta
A Great Book on Diophantine Equations
Number Theory From BMO Round 2 | Diophantine Equation | Math Olympiad Training
Solve Diophantine Equations by Factoring
Learn This Simple Trick | Math Olympiad Training | Diophantine Equations
Hilbert's 10th Problem: Decision Problem on Solvability of Diophantine Equations
Diophantine Equation on Powers and Factorials | Singapore Mathematical Olympiad 2008
British Mathematical Olympiad 1988 Problem 4
The British Mathematical Olympiad Diophantine Equation
Can You Find Integer Solutions? | Diophantine Equations | Math Olympiad Training
The making of an exponential Diophantine equation.
A Diophantine Equation | Number Theory
2023 Number Challenge: Solve a Diophantine equation with 2023
A Symmetric Problem on Divisibility
A system of diophantine equations | feat. Fermat's Last Theorem | must watch till the end
Solving an Interesting Diophantine Equation in Number Theory - Math Olympiad
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
Diophantine Equation | Number Theory | Olympiad Mathematics
A Nice Symmetric System of Equations | Baltic Way 2021
How to find the positive integers that satisfy a Diophantine equation? Mathematical Olympiad.
Solving A Nice Diophantine Equation from Romania
MEI FPT: Number Theory 4 - Diophantine equations: Introducing Diophantine equations
4 Equations 4 Unknowns | British Mathematical Olympiad 2003
Комментарии
 0:10:16
0:10:16
 0:14:43
0:14:43
 0:00:18
0:00:18
 0:03:03
0:03:03
 0:01:58
0:01:58
 0:00:45
0:00:45
 0:00:49
0:00:49
 0:08:07
0:08:07
 0:07:58
0:07:58
 0:12:48
0:12:48
 0:01:54
0:01:54
 0:10:49
0:10:49
 0:00:42
0:00:42
 0:06:29
0:06:29
 0:10:26
0:10:26
 0:02:25
0:02:25
 0:07:35
0:07:35
 0:00:38
0:00:38
 0:04:11
0:04:11
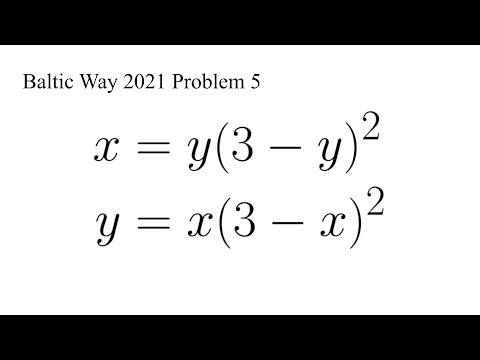 0:11:49
0:11:49
 0:12:11
0:12:11
 0:09:09
0:09:09
 0:05:17
0:05:17
 0:08:14
0:08:14