Aliakbar Daemi: Lagrangians, SO(3)-instantons and the Atiyah-Floer Conjecture

Aliakbar Daemi (WUSTL): Lagrangians, SO(3)-instantons and the Atiyah-Floer Conjecture (7/10/20 4pm GMT)

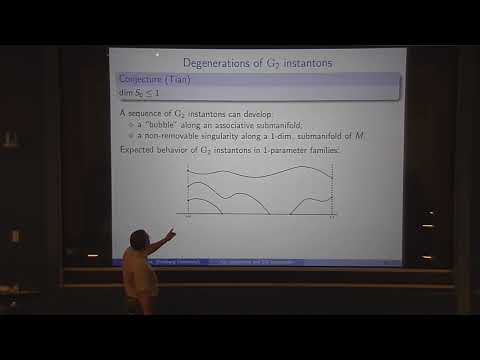
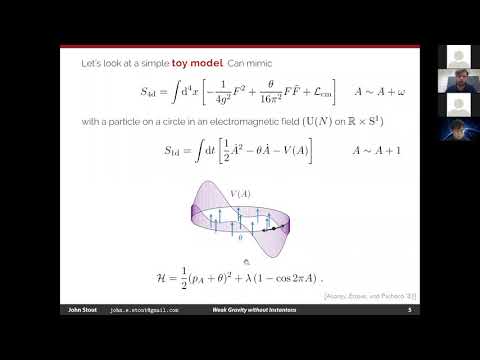
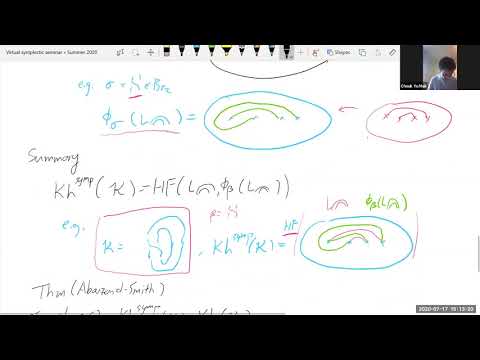
A useful tool to study a 3-manifold is the space of representations of its fundamental group into a Lie group. Any 3-manifold can be decomposed as the union of two handlebodies. Thus representations of the 3-manifold group into a Lie group can be obtained by intersecting representation varieties of the two handlebodies. Casson utilized this observation to define his celebrated invariant. Later Taubes introduced an alternative approach to define Casson invariant using more geometrical objects. By building on Taubes' work, Floer refined Casson invariant into a 3-manifold invariant which is known as instanton Floer homology. The Atiyah-Floer conjecture states that Casson's original approach can be also used to define a graded vector space and the resulting invariant of 3-manifolds is isomorphic to instanton Floer homology. In this talk, I will discuss a variation of the Atiyah-Floer conjecture, which states that framed Floer homology (defined by Kronheimer and Mrowka) is isomorphic to symplectic framed Floer homology (defined by Wehrheim and Woodward). I will explain how the closed-open string map is related to framed Floer homology. Finally I comment on how earlier works of Seidel and Smith might provide useful computational tools for framed Floer homology. This talk is based on a joint work with Kenji Fukaya and Maksim Lipyanskyi.

Questions

00:53:27 Rohil Prasad: So should this be thought of as “stretching” the cylinder Y x R across the Heegaard surface \Sigma?

00:55:16 Paolo Ghiggini: What are the asymptotic conditions for x \to \pm \infty?

01:17:57 Paolo Ghiggini: Can a similar strategy be used to reprove HM=HF?

01:18:18 Rohil Prasad: regarding the four-color theorem story - what would the symplectic side look like for Kronheimer-Mrowka’s instanton homology of webs? Are the relevant orbifold representation varieties smooth/symplectic in this setting or are there singularities to take care of?