Cheuk Yu Mak: Symplectic annular Khovanov homology

Cheuk Yu Mak (Cambridge): Symplectic annular Khovanov homology (7/17/20 7pm GMT)

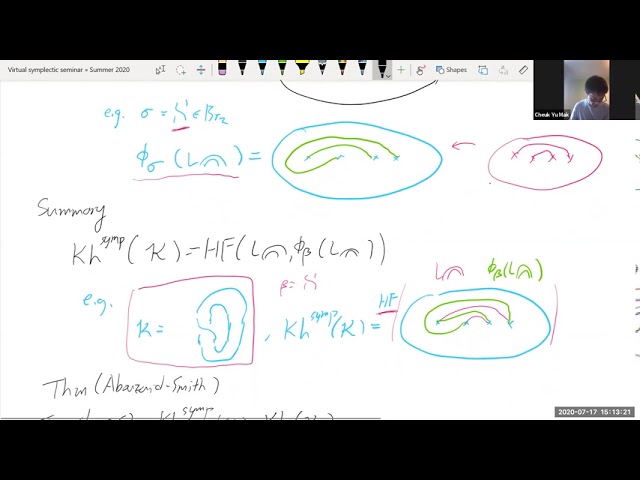
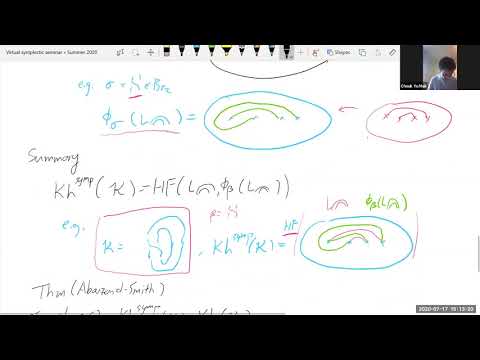
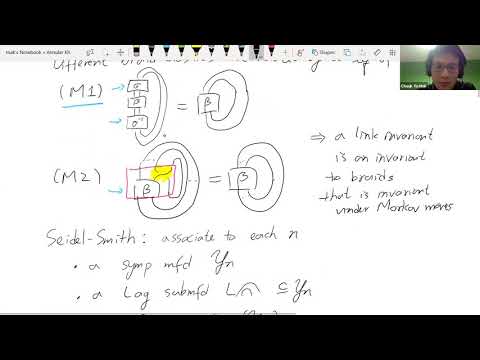
Annular Khovanov homology is an invariant of annular links (links in a solid torus) introduced by Asaeda-Przytycki-Sikora as an analogue of Khovanov homology for links. Auroux-Grigsby-Wehrli showed that the first piece of the annular Khovanov homology can be identified with the Hochschild homology of the Fukaya-Seidel category of A_n Milnor fibers with coefficients in braid bimodules. In this talk, we will introduce a symplectic version of annular Khovanov homology using Hochschild homology of the Fukaya-Seidel category of more general type A nilpotent slices. Building on the work of Abouzaid-Smith and Beliakova-Putyra-Wehrli, we show that the symplectic version is isomorphic to the ordinary version. Finally, we will explain how to derive a spectral sequence from the symplectic annular Khovanov homology to the symplectic Khovanov homology directly using symplectic geometry. This is based on a joint work with Ivan Smith.

Questions

00:37:39 Kyler Siegel: Is anything known about characteristic p?

00:43:16 Y. Baris Kartal: Is it easier to compute than fixed pt HF?

00:58:13 Denis Auroux: I am confused. Why is K in the image of I_2 ? and why is this hom zero ?

01:06:06 Denis Auroux: I am now confused again about the geometry of the map D.

01:14:33 Mohammed Abouzaid: Can you say something about the relationship with Honda et al

01:17:05 Semen Rezchikov: Can you say something about the relation to representation theory (e.g. category O) and potential generalizations of this result?