filmov
tv
Integral of 1/(t^2*sqrt(t^2-16)), trig sub, know this for your calculus 2 class

Показать описание
Integral of 1/(t^2*sqrt(t^2-16)), trig substitution, calculus 2 tutorial.
----------------------------------------
#blackpenredpen #math #calculus #apcalculus
----------------------------------------
#blackpenredpen #math #calculus #apcalculus
Integral of 1/(t^2*sqrt(t^2-16)), trig sub, know this for your calculus 2 class
Integral 1/t^2sqrt(t^2-16)
Evaluate the Integral dt/(t^2(sqrt(t^2 -16)). Sketch Label the associated right triangle
Evaluate the integral.
integral of 1/sqrt(x^2+16)
Trigonometric Substitution
integral of 1/(x^2*sqrt(x^2-1), trig substitution, calculus 2 tutorial.
Integration By Trigonometric Substitution
Trigonometric ratios of inverse trig functions
Trigonometric substitution for a definite integral sqrt(x^2-1)/x^2 on [1,2].
Integration of Square Root Function (1 of 2: Using a Trig Substitution to help with integration)
when calculus students use trig identities too early
Integral 9: int sqrt(1+t^2)/t dt
Integration by Trigonometric Substitution | Step-by-step explanation
Integration - HOW TO: Trigonometric Substitution (Difficult Level, Part 1)
Trig sub definite integral x^2*sqrt(1-x^2), substitution x=sin(theta), requires trig identities.
Calculus 2 Lecture 7.3: Integrals By Trigonometric Substitution
∫ Integral by Trigonometric Substitution problem ! ! ! ! !
integral t*sqrt(t^2 + 1) dt
Integration of Rational Functions By Completing The Square - Calculus
Trig Substitution | Final Step | y=(1/16)*cos(theta)
A Trigonometric Party - Integral of sqrt(x^2-1) via trig substitution
Calculus | Integration by Trigonometric Substitution | ∫√(1-x²)dx
Integral of 1/(1+√x) - Integration by Substitution, Calculus 2
Комментарии
 0:07:21
0:07:21
 0:03:32
0:03:32
 0:06:49
0:06:49
 0:11:09
0:11:09
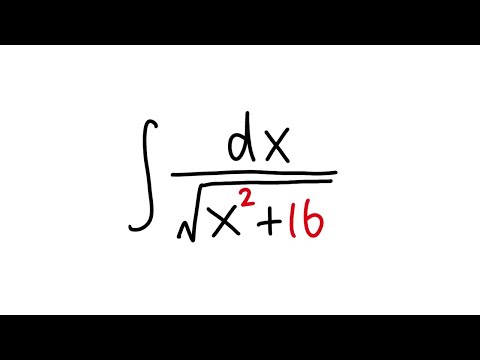 0:08:38
0:08:38
 0:19:50
0:19:50
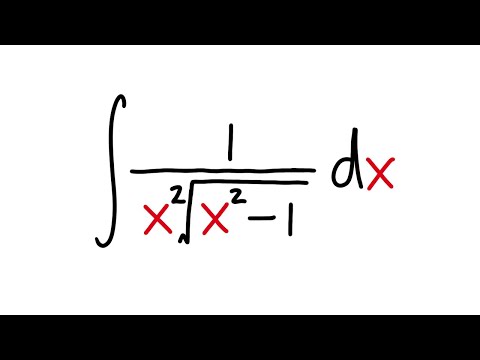 0:04:23
0:04:23
 0:15:55
0:15:55
 0:06:51
0:06:51
 0:07:12
0:07:12
 0:14:14
0:14:14
 0:00:43
0:00:43
 0:14:20
0:14:20
 0:19:05
0:19:05
 0:18:17
0:18:17
 0:05:41
0:05:41
 2:09:24
2:09:24
 0:05:15
0:05:15
 0:01:36
0:01:36
 0:26:06
0:26:06
 0:01:00
0:01:00
 0:19:51
0:19:51
 0:10:16
0:10:16
 0:01:37
0:01:37