filmov
tv
Integration by Substitution: Definite Integrals

Показать описание
Calculus: We note how integration by substitution works with definite integrals using the First Fundamental Theorem of Calculus. Two methods are given, and examples used are (a) int_0^1 x(x^2+1)^5 dx, (b) int_0^{pi/4} tan(x) sec^2(x) dx, (c) int_{-1}^1 x(1-x^2)^2 dx, and (d) int_0^2 x(1-x^2)^2 dx.
U-substitution With Definite Integrals
_-substitution: definite integrals | AP Calculus AB | Khan Academy
How To Integrate Using U-Substitution
Integration Using The Substitution Rule
Definite Integral With U-Substitution
U-substitution with definite integral
Substitution Method for Definite Integrals **careful!**
Substitution with Definite Integrals Problem 1 (Calculus 2)
Week 13 review problems for Calculus 1 (Fall 2024)
Integration by Substitution: Definite Integrals
_-substitution: definite integral of exponential function | AP Calculus AB | Khan Academy
A-Level Maths: H5-20 Further Integration: Definite Integrals with Integration by Substitution
Integration by Substitution: Definite Integral
How to Use the Substitution Rule for Integrals #shorts
Definite Integrals by Substitution
Integration by Substitution (2 of 2: Definite integrals)
Integration by Substitution (Introduction)
Properties of Definite Integrals (6 of 6: Using Integration by substitution to evaluate integral)
Killer Tricks and Shortcuts | Definite Integration | Bhoomika Ma'am | BYJU'S
How to Integrate Using U-Substitution (NancyPi)
The Substitution Rule for Definite Integrals
Calculus I - 4.5.2 Integration by Substitution: Definite Integrals
How to evaluate the definite integral with trig and u substitution
DEFINITE INTEGRATION SHORTCUT- Trick to calculate Definite Integrals in 3 seconds
Комментарии
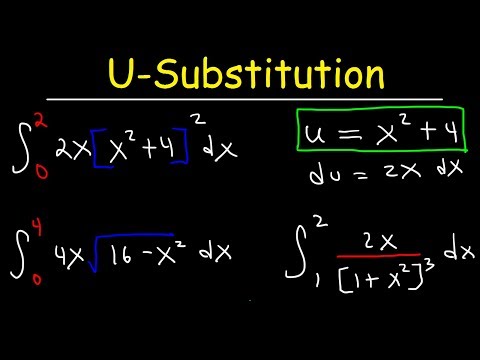 0:11:03
0:11:03
 0:05:53
0:05:53
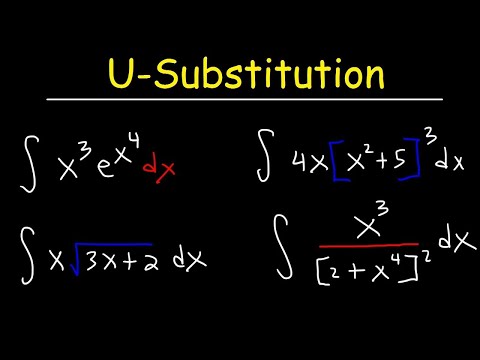 0:21:35
0:21:35
 0:10:40
0:10:40
 0:12:56
0:12:56
 0:05:39
0:05:39
 0:04:51
0:04:51
 0:05:39
0:05:39
 1:36:20
1:36:20
 0:09:45
0:09:45
 0:07:53
0:07:53
 0:05:29
0:05:29
 0:03:05
0:03:05
 0:00:39
0:00:39
 0:11:18
0:11:18
 0:09:53
0:09:53
 0:14:49
0:14:49
 0:08:23
0:08:23
 0:01:00
0:01:00
 0:25:48
0:25:48
 0:10:39
0:10:39
 0:09:31
0:09:31
 0:02:27
0:02:27
 0:00:59
0:00:59