Comprehension: \( \quad: \) Consider the real valued function \( f: R \rightarrow R \) defined a...

Comprehension: \( \quad: \) Consider the real valued function \( f: R \rightarrow R \) defined as \( f(x)=x^{2} e^{-x} \)
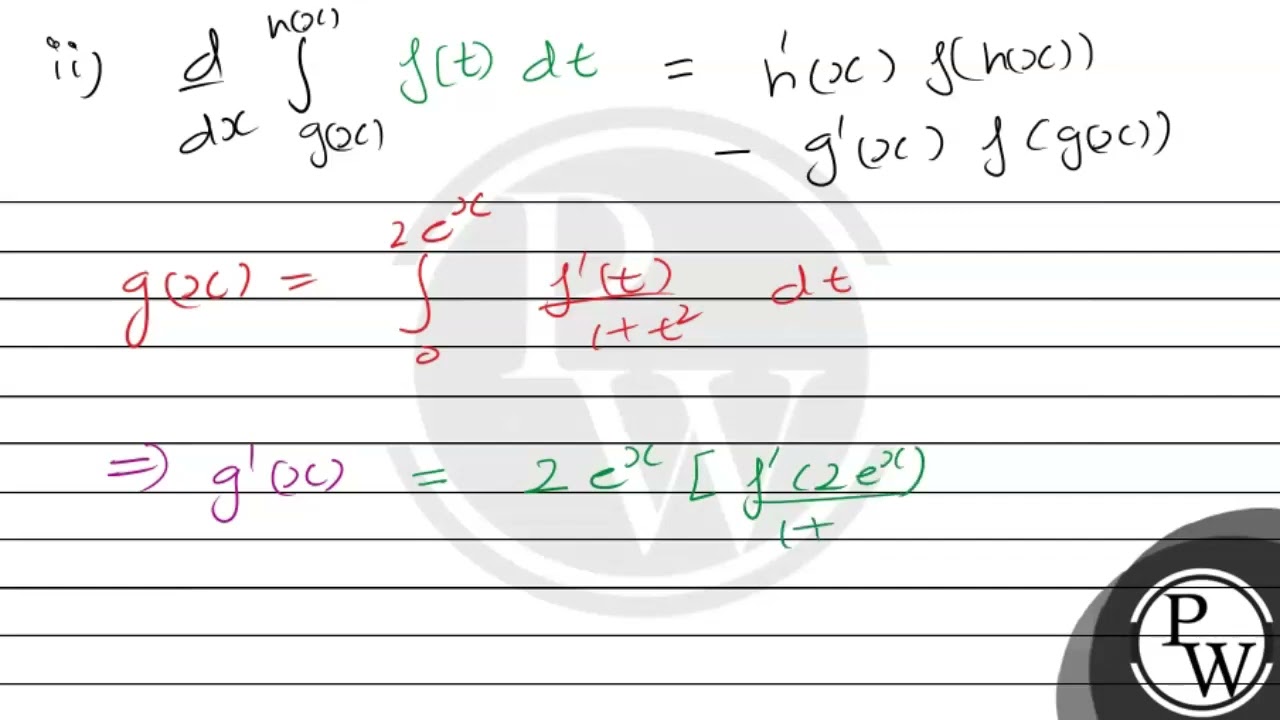
Let \( g(x)=\int_{0}^{2 e^{x}} \frac{f^{\prime}(t)}{1+t^{2}} d t \), then
(a) \( g(x) \) increases on \( (-\infty, 0) \) and decreases on \( (0, \infty) \)
(b) \( g(x) \) has a local minimum at \( x=0 \)
(c) \( g(x) \) decreases on \( (-\infty, 0) \) and increases on \( (0, \infty) \)
(d) \( g(x) \) has neither maximum nor minimum at \( x=0 \)