filmov
tv
Calculus 2: The Integral of 1/x and a Rant Against Absolute Values
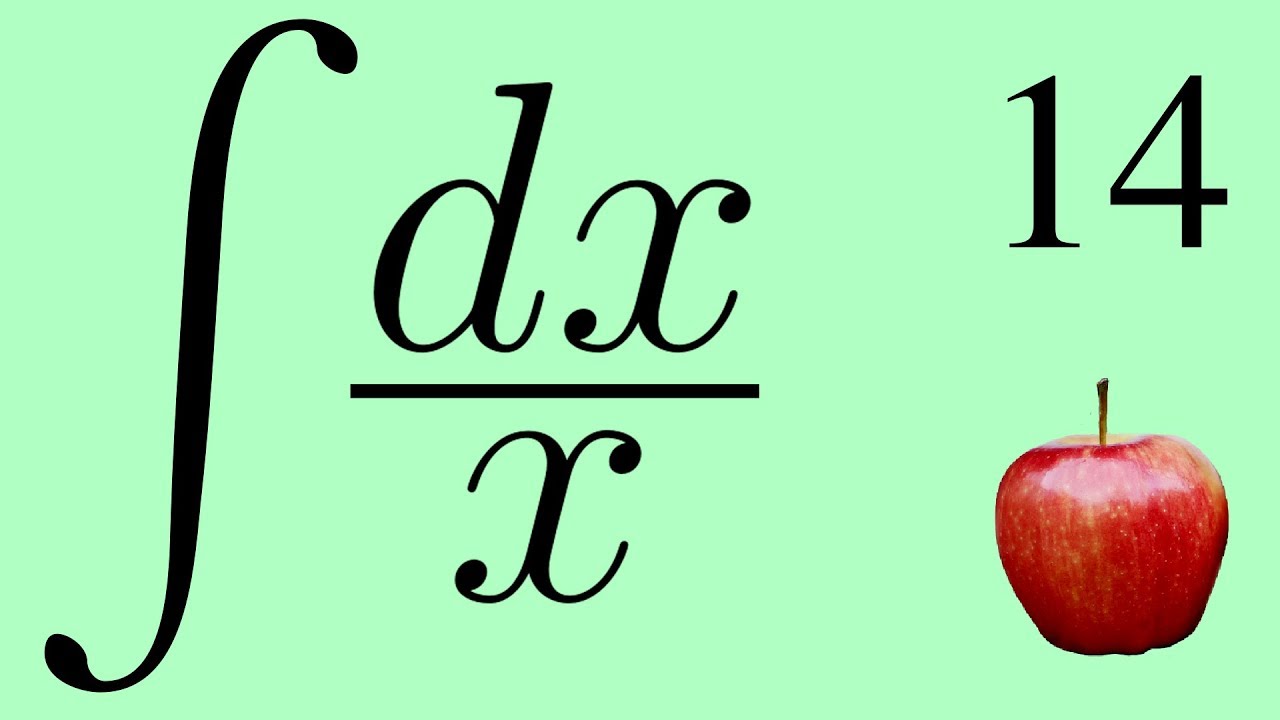
Показать описание
Calculus 2 - Basic Integration
i did another 100 integrals!
Introduction to integral calculus | Accumulation and Riemann sums | AP Calculus AB | Khan Academy
ALL OF Calculus 2 in 5 minutes
Calculus 2 Lecture 7.1: Integration By Parts
Integration (Calculus)
What Integration Technique Should I Use? (trig sub, u sub, DI method, partial fractions) calculus 2
Improper Integrals - Convergence and Divergence - Calculus 2
Calculus II ep34: Taylor series (Dec 3, 2024)
Welcome to Calculus II
the ultimate integral starter (u sub, IBP, trig sub, partial fractions & more)
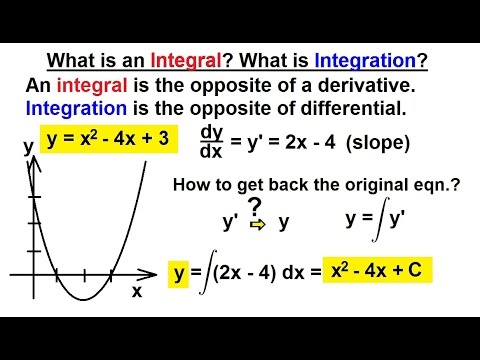
Calculus 2: Integration (1 of 9) What is an Integral? What is Integration?
Calculus 2 - Integral Test For Convergence and Divergence of Series
Calculus 2 - Full College Course
Integration By Parts
Legendary Calculus Book for Self-Study
Finding the Area Between Two Curves by Integration
Calculus 2: Strategy for Integration (Video #5) | Math with Professor V
Calculus 2 In Less Than 20 Minutes (Complete Overview Of Integral Calculus)
GCE 2018/2019 Paper 2 - Integration Calculus
Calculus 1 - Integration & Antiderivatives
Integration By Partial Fractions
Definite Integral
Math Integration Timelapse | Real-life Application of Calculus #math #maths #justicethetutor
Комментарии
 0:26:12
0:26:12
 0:00:16
0:00:16
 0:04:52
0:04:52
 0:06:09
0:06:09
 1:54:37
1:54:37
 0:07:04
0:07:04
 0:22:40
0:22:40
 0:13:56
0:13:56
 1:11:50
1:11:50
 0:08:48
0:08:48
 5:56:58
5:56:58
 0:07:15
0:07:15
 0:28:17
0:28:17
 6:52:53
6:52:53
 0:13:17
0:13:17
 0:00:23
0:00:23
 0:07:52
0:07:52
 0:30:31
0:30:31
 0:19:35
0:19:35
 0:10:25
0:10:25
 0:40:04
0:40:04
 0:41:07
0:41:07
 0:11:05
0:11:05
 0:00:09
0:00:09