filmov
tv
Derivative of arcsec(x)

Показать описание
Derivative of arcsec(x)
Derivative of arcsec x
derivative of inverse secant
68 Derivative of arcsec(x)
Derivative of arcsec(x)
Inverse Trigonometric Derivatives f(x) = arcsec(x/2)
Derivative of arccsc(x)
5 - The derivative of arcsec(x)
The derivative of arcsec(x)
Implicit differentiation of arcsec x in under 5 minutes (Calculus 1)
Derivative of arcsec(x) (or inverse sec(x) or arcsecant(x)) - Simple Intro and Proof
Understand the arccsc(x) & arcsec(x) Derivatives
Derivative of arcsec(x)
Derivative of arcsec(2x - x)
Derivative of arcsec(x) + x
Derivative of Inverse Secant
HOW DO YOU DIFFERENTIATE arcsec(x)? (INVERSE TRIGONOMETRIC DERIVATIVES, INVERSE SECANT FUNCTION)
Derivative of Arcsec x Proof (Using Implicit Differentiation)
derivative of arcsec(9x)
Derivative of arcsec(x)/2
derivative of arcsec(x) formula rule, arcsec'(x) // inverse trig, arctrig #Shorts
Derivative of arcsec(x)
derivative of arcsec(9x)
Derivative of an Arcsec Function
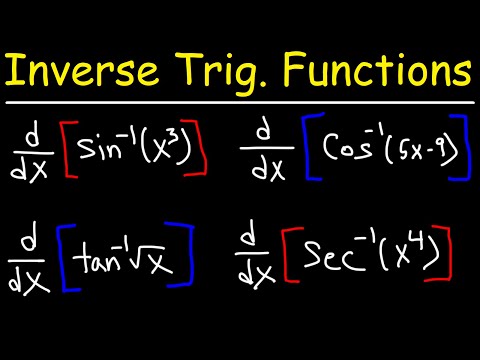
Derivatives of Inverse Trigonometric Functions
Комментарии
 0:01:46
0:01:46
 0:04:42
0:04:42
 0:01:07
0:01:07
 0:04:28
0:04:28
 0:03:35
0:03:35
 0:11:10
0:11:10
 0:01:03
0:01:03
 0:09:09
0:09:09
 0:04:52
0:04:52
 0:12:46
0:12:46
 0:10:47
0:10:47
 0:13:01
0:13:01
 0:01:40
0:01:40
 0:01:49
0:01:49
 0:05:46
0:05:46
 0:17:31
0:17:31
 0:05:58
0:05:58
 0:01:13
0:01:13
 0:02:18
0:02:18
 0:00:25
0:00:25
 0:06:39
0:06:39
 0:01:01
0:01:01
 0:07:32
0:07:32
 0:06:19
0:06:19