filmov
tv
QED Prerequisites Geometric Algebra 5- Multivectors
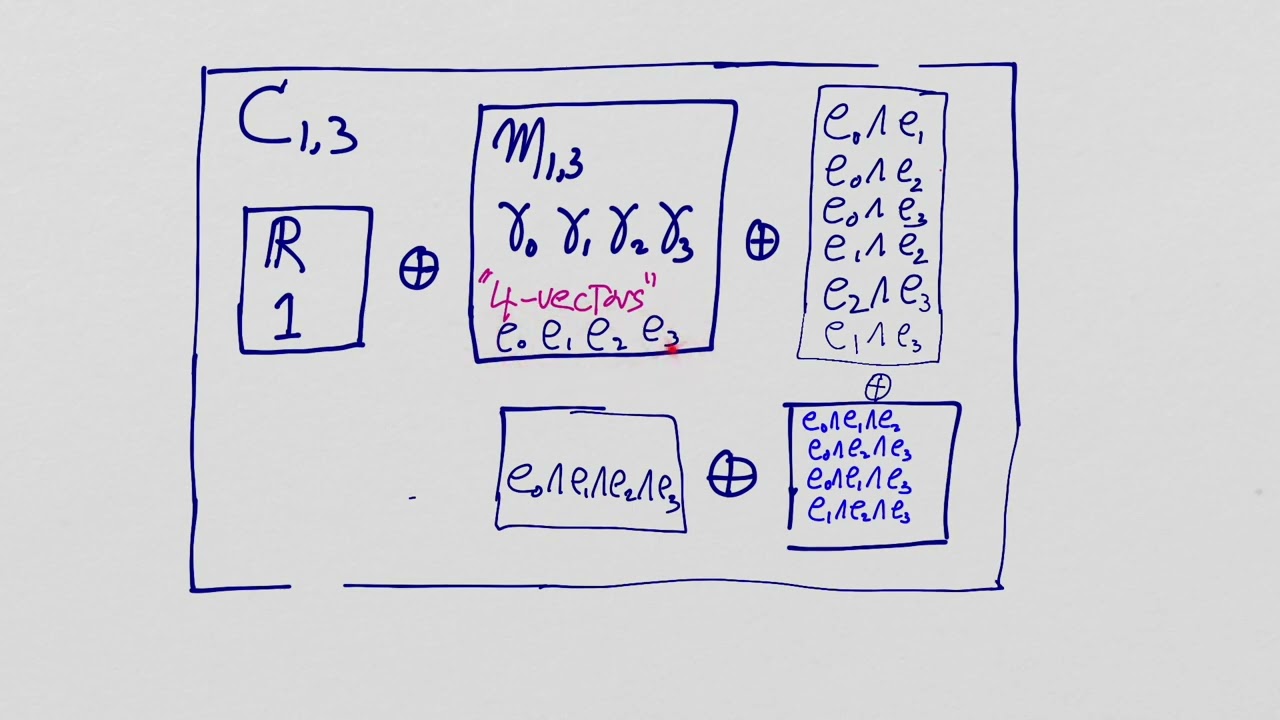
Показать описание
In this lesson we introduce the idea of multivectors and emphasize the need to understand how to take the spacetime product of any two multivectors in the Spacetime Algebra. We demonstrate how this is done for the product between a vector and a bivector and we interpret the meaning of each part of the resulting multivector.
The software I usually use to produce the lectures is:
The software I usually use to produce the lectures is:
QED Prerequisites Geometric Algebra 5- Multivectors
QED Prerequisites Geometric Algebra: Spacetime.
QED Prerequisites Geometric Algebra 15: Complex Structure
QED Prerequisites Geometric Algebra 7 - Multivector Addition
QED Prerequisites Geometric Algebra: Introduction and Motivation
QED Prerequisites Geometric Algebra 6 - Multivector Products
QED Prerequisites -Geometric Algebra 12: Reciprocal basis, calculation of components
QED Prerequisites Geometric Algebra 4: The antisymmetric part
QED Prerequisites Geometric Algebra 17 - Bivector products
QED Prerequisites Geometric Algebra 16: Canonical Bivectors
QED Prerequisites Geometric Algebra 29 - Relativistic velocity
QED Prerequisites 20 Setup For Rotations
QED Prerequisites Geometric Algebra 21 Rotors
QED Prerequisites Geometric Algebra 9: Multivector Structure
QED Prerequisites 30 Geometric Algebra - SPINORS
QED Prerequisites :Geometric Algebra 32: Amplitudes and eigenvalues
QED Prerequisites : Geometric algebra 31 - More about spinors (corrected!)
ICACGA 2022: Geometric Algebra and Distance Matrices
Jeff Bezos Quit Being A Physicist
Duality transformations in geometric algebra
Intro to clifford, a python package for geometric algebra
Spherical basis vectors in geometric algebra
ICACGA 2022: Illustrating Geometric Algebra and Differential Geometry in 5D Color Space
[Lesson 15 and 1/3] QED Prerequisites Lorentz Transformations: Rod and Ring Paradox
Комментарии
 0:56:36
0:56:36
 0:37:21
0:37:21
 0:32:18
0:32:18
 0:31:04
0:31:04
 0:38:31
0:38:31
 0:58:35
0:58:35
 0:44:10
0:44:10
 0:39:51
0:39:51
 0:53:30
0:53:30
 0:49:30
0:49:30
 0:53:38
0:53:38
 0:51:53
0:51:53
 0:52:55
0:52:55
 0:58:11
0:58:11
 1:00:51
1:00:51
 0:50:14
0:50:14
 0:53:05
0:53:05
 0:18:15
0:18:15
 0:00:56
0:00:56
 0:18:23
0:18:23
 0:10:08
0:10:08
 0:08:06
0:08:06
 0:28:41
0:28:41
 0:47:10
0:47:10