results on supremum and infimum in real numbers university iit jam 2021 gate mathematics csir net

For Notes and Practice set WhatsApp @ 8130648819 or visit our Website

Real Analysis: Interior points, limit points, open sets, closed sets, bounded sets, connected sets, compact sets, completeness of R. Power series (of real variable), Taylor's series, radius and interval of convergence, term-wise differentiation and integration of power series.

results on supremum and infimum in real numbers university iit jam 2021 gate mathematics4

Concept Used:
Results on supremum and infimum
1. The lub (if it exists) of a set S, may or may not belongs to S.
2. If the set S is bounded below, it admits an infinite number of lower bounds because every number less than a lower bound is also a lower bound.
3. The glb (if it exists) of a set S, may or may not belong to the set S.
4. A non-empty bounded closed set contains its supremum as well as infimum.
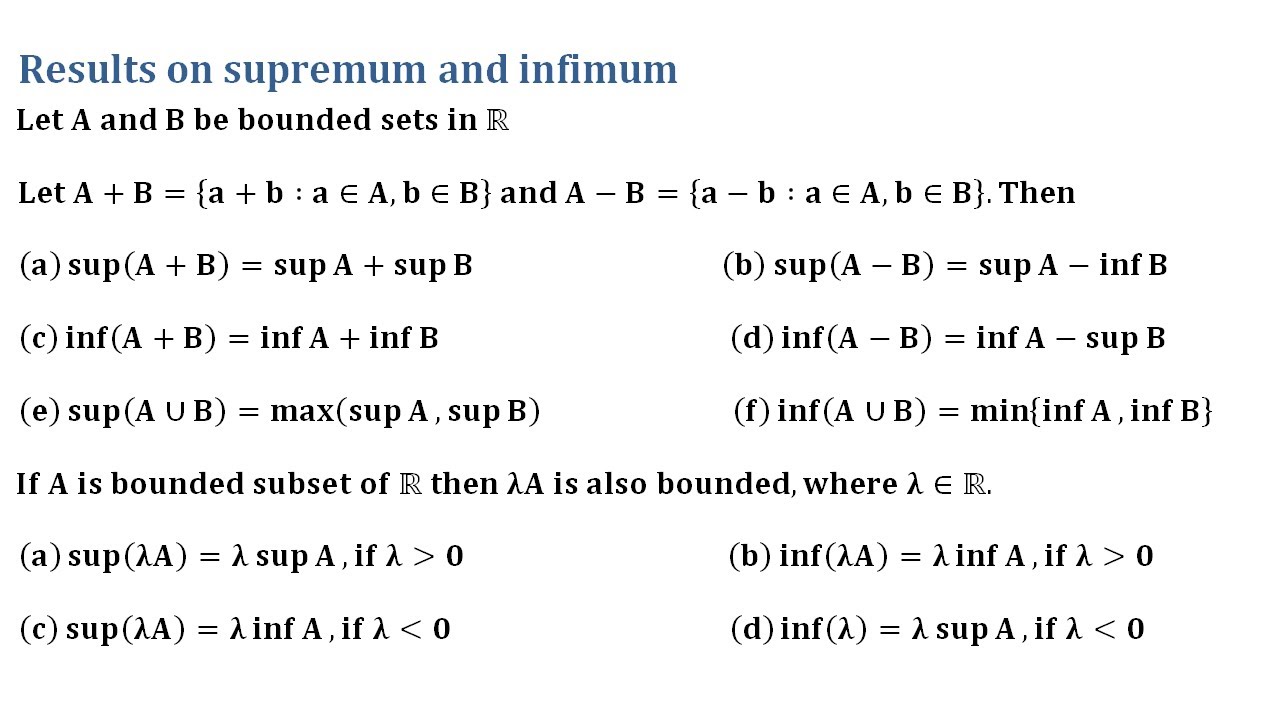
5. Let A and B be bounded sets in R
Let A+B={a+b∶a∈A,b∈B} and A-B={a-b∶a∈A,b∈B}.Then
(a) sup⁡(A+B)=sup⁡A+sup⁡B (b) sup⁡(A-B)=sup⁡A-inf⁡B
(c) inf⁡(A+B)=inf⁡A+inf⁡B (d) inf⁡(A-B)=inf⁡A-sup⁡B
(e) sup⁡(A∪B)=max⁡(sup⁡A,sup⁡B ) (f) inf⁡(A∪B)=min⁡{inf⁡A,inf⁡B }
6. If A is bounded subset of R then λA is also bounded,where λ∈R.
(a) sup⁡(λA)=λ sup⁡A,if λ is positive (b) inf⁡(λA)=λ inf⁡A,if λ is positiv
(c) sup⁡(λA)=λ inf⁡A,if λ is negative (d) inf⁡(λ)=λ sup⁡A, if λ is negative