A Fun One from the Bulgarian IMO

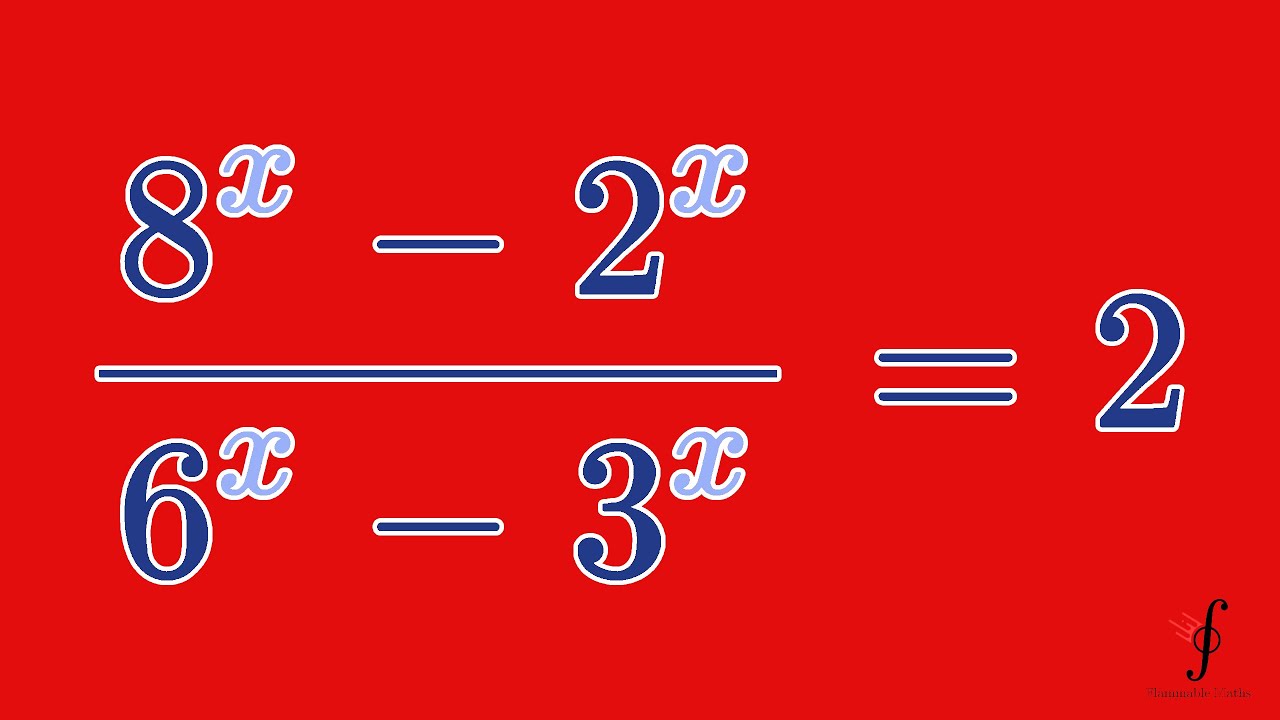
Today a fun one from the 2007 Bulgarian Maths Olympiad: Find all solutions x in the integers satisfying 8^x-2^x/6^x-3^x=2 :D Enjoy! =)

Help me create more free content! =)

--------------------------------------------------------------------------------
Wanna send me some stuff? lel:

Postfach 11 15
06731 Bitterfeld-Wolfen
Saxony-Anhalt
Germany
--------------------------------------------------------------------------------

[Yogi]

Introduction was so trivial that he left it as an exercise for viewers.

[MartiniComedian]

Me looking at the thumbnail:
8 - 2 = 6
6 - 3 = 3
6 / 3 = 2
so
x = 1 and that's it.

Now I'll watch the video

[ReallyAmateurPianist]

Great video and nice solution! I solved it pretty much the same way as you.

Only difference was, that for "case 3" I used parity as well to show that x cannot be > 1. For x > 1, the LHS ( 3*x ) is always odd, and the RHS ( 2^( 2x-1 ) + 2^( x-1 ) ) is always even.

[petrie]

Once you're at 2^x[(2^x+1)/3^x] = 2, you can use unique prime factorization of rationals. The term in brackets is a ratio of odd numbers, so it doesn't contribute to the 2 exponent in the factorization. Thus x = 1.

[osmanfb]

thanks for the video. when you divided the numerator and the denominator by 2^x-1 you need add x is not equal to 0. So that you start guessing from 1 for the positive integers.

[emilsriram]

nice to see when my small country gets mentioned somewhere

[yeezyjeezy]

That's cool and all but
(8^x-2^x)/(6^x-3^x)
=(8-2)^x/(6-3)^x
=6^x/3^x
=(6/3)^x
=2^x=2
therefore x=1
You really gotta start looking for shortcuts to make these problems easier

[lordmatthew]

If there was only 1 question which is this on the olympiad, I believe 99% won the competition.

[aaronpumm]

you can also note that (2^x+1) is an integer and must be equal to 3^x/2^(x-1) which can only be an integer if 2^(x-1) = 1 => x = 1

[theanist]

If hit and trial is accepted then

x=1

[sebby]

a much interesting solution for 3^x=2^(2x-1) + 2^(x-1)
you can rewrite the equation as 2*(3^x) = 4^x + 2^x
that is equivalent to 4^x - 3^x = 3^x - 2^x
the lagrange theorem says that for a differentiable function on the interval [a, b] there is a c in the interval (a, b) that satisfies the equation
f'(c) = f(b) - f(a) / b - a
so let f(t) be t^x where x is a solution of the problem
lets chose a variable m in (2, 3) and n in (3, 4)
according to lagrange, the equation becomes
f(4) - f(3) = f(3) - f(2) ant that is equivalent to
f'(n) = f'(m)
that means that
x*n^(x-1) = x*m(x-1)
and now there are only two cases
x=0 that is obvious
and x different to 0 and that means x=1 or m=n
but n>m so x=0 and x=1 are the only solutions

[quixata]

When you remove 2^x - 1 from the numerator and denominator, you are essentially assuming that x <> 0

[RexxSchneider]

When you cancel (2^x - 1) at 4:55, your "cool trick" doesn't work if (2^x - 1) is zero. That is, you've now disallowed the case when x=0, so you simply can't "take a look at x=0 for example" at 6:38. It's a common mistake to make, but best avoided when you're trying to teach.
Also, when you're dealing with integers, "the limit as x approaches zero" (at 7:37) is meaningless. You already know that x=0 is not a meaningful solution anyway. Similarly L'Hospital's rule is only usable for continuous functions. If you're going to simply work trial and error from 8:15 onwards, you might as well have saved all the prior manipulation and just done that from the start.

[txikitofandango]

(8^x - 2^x) / (6^x - 3^x) = 2
2^x(2^(2x) - 1) / 3^x(2^x - 1) = 2
2^x(2^x + 1) / 3^x = 2

Any integer bigger than 2 won't work because the numerator will be more than twice the denominator. Plugging in x=0 doesn't work. Negative exponents don't work because then you get a power of 3 in the numerator with no power of 3 in the denominator. Also, (3/2)^x grows too big very fast. Pretty confident x=1 is the only solution.

[thelmexsniper]

This question was reused in the Instituto Militar de Engenharia (Military Engennering Institute) in the 2022 edition of their admission text... luckly i saw the solution of this one and was able to destroy that one in the exam!

[StepanSmith]

I challenge you to solve the same equation over complex numbers (with Lambert W)

[ignacioarroyo]

A random guy I know from my English class showed me this problem and he told me 'solve for x in R', so I came up with a bunch of inequalities and concluded X should be between -2 and 3 if I remember correctly. When I googled the actual problem, I felt so good about myself since it was just enough to test those 5 remaining cases 😅

[aweebthatlovesmath]

hi papa flammy i have question I hate guessing, i like to approach it the math way how about you?

[Davidthesolver]

Hi pappy ! Have you considered solving some problems from the JBMO or the BMO

[fidgetspinner]

you can also just take log2 on both sides and then simplify until you're left with x=1