Minimum Spanning Trees with Prim's and Kruskal's Algorithms

preview_player
Показать описание
A complete graph with three or more nodes is full of cycles allowing multiple paths or walks between nodes or vertices. When a weight is added to each edge of a walk, we can determine the optimal walk. This has applications ranging from networking to travel planning.

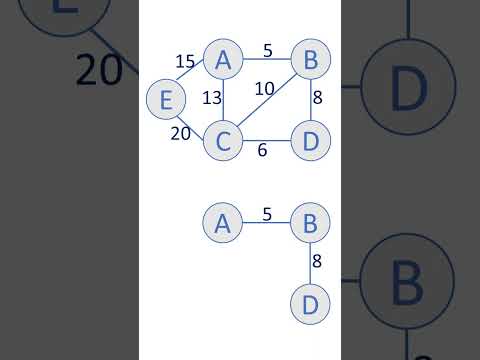
After defining spanning trees, weighted graphs, and minimum spanning trees, we present the Prim and Kruskal algorithms, two tools we can use to determine the minimum spanning tree from a connected graph.

Timestamps
00:00 | Intro
00:16 | Tree review
01:27 | Spanning tree description
02:40 | Spanning trees from complete graphs
04:56 | Ethernet "Time to Live" element
06:04 | Weighted graph description
09:15 | What could the weights represent?
10:13 | Minimum spanning tree description
11:53 | Presenting a complete graph example - airline connections
13:28 | Prim's algorithm description
15:24 | Prim's algorithm example
18:06 | Kruskal's algorithm description
19:44 | Kruskal's algorithm example

Hashtags
#tree #prim #kruskal
  1. Intermation
  2. nodes
  3. vertex
  4. vertices
  5. edge
  6. tree
Рекомендации по теме
3.5 Prims and 0:20:12

3.5 Prims and Kruskals Algorithms - Greedy Method

Prim's Algorithm 0:07:18

Prim's Algorithm

L-4.9: Prim's Algorithm 0:09:55

L-4.9: Prim's Algorithm for Minimum Cost Spanning Tree | Prims vs Kruskal

How does Prim's 0:00:49

How does Prim's Algorithm work? #shorts

Prim's Minimum Spanning 0:14:53

Prim's Minimum Spanning Tree Algorithm | Graph Theory

#2 Prims Algorithm 0:05:42

#2 Prims Algorithm Minimum | How to find Spanning Tree Greedy Search Algorithm by Dr. Mahesh Huddar

How Do You 0:11:12

How Do You Calculate a Minimum Spanning Tree?

Prim's Algorithm for 0:11:32

Prim's Algorithm for Minimum Spanning Trees (MST) | Graph Theory

NPTEL Design and 0:01:32

NPTEL Design and analysis of algorithms Week 1 Assignment 1 Answers Solution Quiz | 2025 - Jan

Networks: Prim's Algorithm 0:07:40

Networks: Prim's Algorithm for finding Minimum spanning trees

Prim's Algorithm for 0:00:47

Prim's Algorithm for Creating a Minimum Spanning Tree (MST) - Min Cost to Connect Points (LC 15...

Minimum Cost Spanning 0:22:35

Minimum Cost Spanning Trees Prims Algorithm

31251 Lecture 12.1: 0:28:47

31251 Lecture 12.1: Minimum spanning trees and Prim's algorithm

Prim's Algorithm: Minimal 0:06:46

Prim's Algorithm: Minimal Spanning Tree

Prim's Algorithm - 0:22:08

Prim's Algorithm - Minimum Spanning Tree - Min Cost to Connect all Points - Leetcode 1584 - Pyt...

Prim's Algorithm | 0:13:02

Prim's Algorithm | Minimum Spanning tree | MST | DAA | Lec-26 | Bhanu Priya

Prim's Algorithm for 0:18:29

Prim's Algorithm for Minimum Spanning Tree | Graph Theory #13

Kruskal's Algorithm: Minimum 0:06:01

Kruskal's Algorithm: Minimum Spanning Tree (MST)

OCR MEI MwA 0:05:34

OCR MEI MwA E: Minimum Spanning Trees: 04 Prim’s Algorithm Example 1

Prim's Algorithm to 0:05:15

Prim's Algorithm to Find Minimum Spanning Tree Example

Proof of Prim's 0:08:21

Proof of Prim's MST algorithm using cut property

Min Cost to 0:00:38

Min Cost to Connect All Points - Minimum Spanning Tree (MST) - Leetcode 1584

G-45. Prim's Algorithm 0:19:10

G-45. Prim's Algorithm - Minimum Spanning Tree - C++ and Java

Prims Algorithm Minimum 0:04:57

Prims Algorithm Minimum Spanning Tree

Комментарии
Автор

wow, cant believe how helpful this was. thank you!

joseeduardo-thzn
Автор

Great video!I think the TTL field should be in the IP header, so L3 routers can drop those packets, but Ethernet is susceptible to broadcast loops so L2 switches use other mechanisms like the spanningntree protocol to ensure the connectivity is dropped. BTW how does this compare to Djikstra's algorithm?

asleepawake
Автор

6:47 - "I actually don't have any loops." Wanna'

zunkman