filmov
tv
Music And Measure Theory

Показать описание
A connection between a classical puzzle about rational numbers and what makes music harmonious.
Thanks to these viewers for their contributions to translations
German: Josh
Russian: e-p-h
Thanks to these viewers for their contributions to translations
German: Josh
Russian: e-p-h
Music And Measure Theory
The Math of Music - TWO MINUTE MUSIC THEORY #32
Music Theory for Beginners: The Measure
Basic Music Theory Concepts That All Beginning Musicians NEED to Know
Music Lesson 3 - What is a Measure?
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
Why Music Theory Has NO RULES (And How To Learn It)
How Pythagoras Broke Music (and how we kind of fixed it)
The Geometry of Music
Why Measure Theory? -- a short introduction
Music Theory Minute #2.1 - Intro to Melodic Structure
Understanding Meter in Music Theory: Simple Meters
Let's Read Music 6 - The Measure (aka Bar)
Music Theory for Beginners: Bar Line
How to Identify Musical Intervals (Music Theory)
Piano Theory: Notes, Time Signature, Measure, Barline, and Ledger Line - Music Theory
Music Theory Lecture - What Every Pro Musician Needs To Know
Music Theory Distilled - Part 3: Rhythm
The Best Book on Measure Theory
Math of Musical Scales, Part 1 of 3
Building Music: Phrases and Periods - TWO MINUTE MUSIC THEORY #35
Measure Theory series to come…
TIME SIGNATURES EXPLAINED // Learn Music Theory
Anacrusis Notation in Music Theory: Understanding the Importance of Pickup Notes
Комментарии
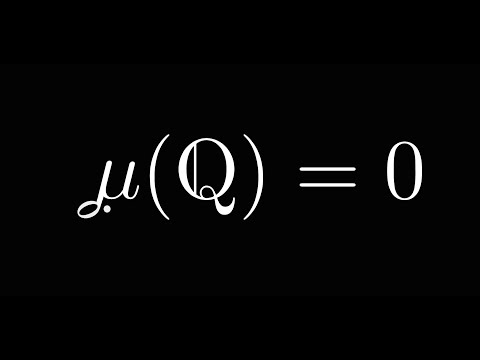 0:13:13
0:13:13
 0:03:44
0:03:44
 0:01:08
0:01:08
 0:06:02
0:06:02
 0:09:34
0:09:34
 0:00:38
0:00:38
 0:12:33
0:12:33
 0:17:40
0:17:40
 0:02:42
0:02:42
 0:01:53
0:01:53
 0:01:11
0:01:11
 0:08:05
0:08:05
 0:01:40
0:01:40
 0:01:15
0:01:15
 0:11:02
0:11:02
 0:07:13
0:07:13
 0:41:33
0:41:33
 0:04:09
0:04:09
 0:01:46
0:01:46
 0:13:52
0:13:52
 0:02:45
0:02:45
 0:21:51
0:21:51
 0:17:03
0:17:03
 0:02:43
0:02:43