filmov
tv
Incircle area in 3-4-5 triangle?
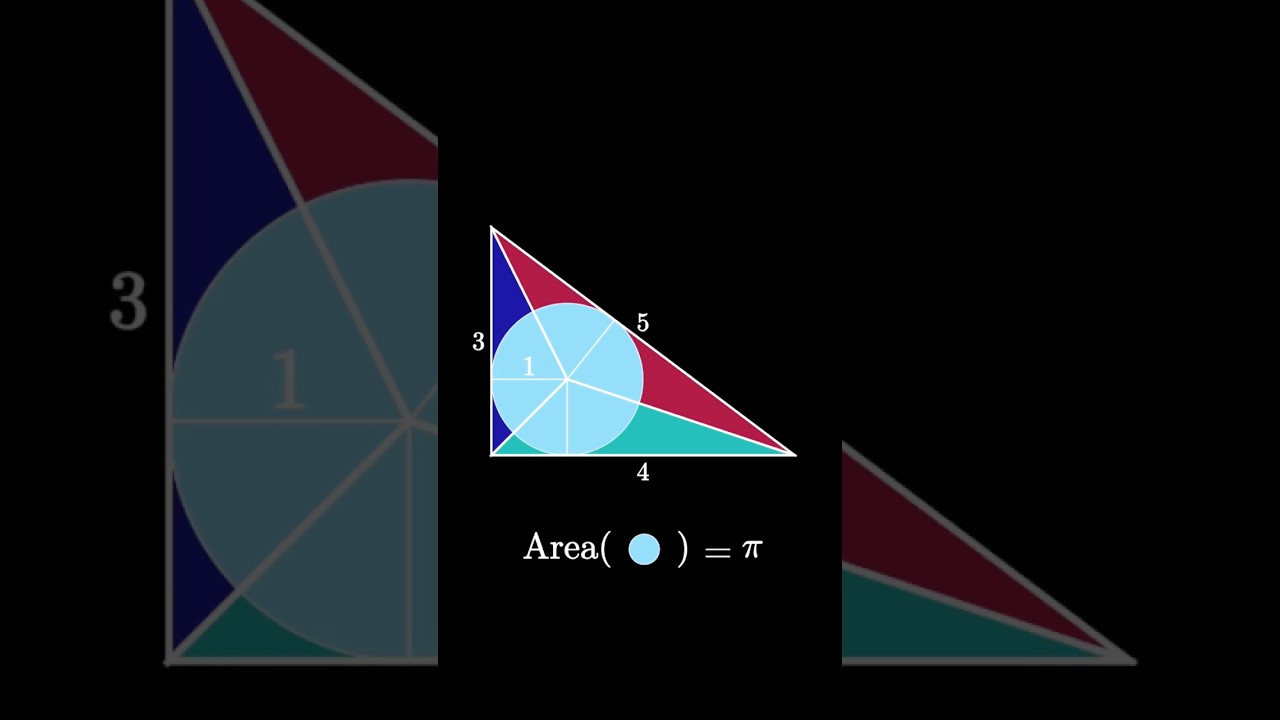
Показать описание
This short demonstrates an intriguing fact about the area of the incircle of the 3-4-5 right triangle. Using two different methods of computing the area of the 3-4-5 rectangle, we find that the inradius has a length of one so that the area of the incircle is Pi!
Thanks!
#manim #math #mathvideo #mathshorts #geometry #circle #triangle #animation #theorem #pww #proofwithoutwords #visualproof #proof #incircle #inradius #incircle #semiperimeter #incenter #pi #righttriangle #area
To learn more about animating with manim, check out:
Thanks!
#manim #math #mathvideo #mathshorts #geometry #circle #triangle #animation #theorem #pww #proofwithoutwords #visualproof #proof #incircle #inradius #incircle #semiperimeter #incenter #pi #righttriangle #area
To learn more about animating with manim, check out:
Incircle area in 3-4-5 triangle?
Incircle Area 3-4-5 Triangle🏆 #incircle #area #maths #tution #ai #puzzle #aisolution #theatozclasses...
COOL!! | Area of the Incircle of a Right Triangle? | Pythagorean Triple (3, 4, 5)
Find the Radius of inscribed circle using Area and sides of Triangle | Find the Radius of circle
Incircle of triangle with sides 4cm,5cm and 6cm
Two Circles Inscribed in a 3-4-5 Triangle
Incircle of a Triangle | Geometric Construction
Can you find the Radius of the circle? | (Triangle inscribed in a circle) | #math #maths
Inscribed Circle in a Right Triangle | Daily Math Geometry
Given a Circle Inscribed in a Triangle, Find the Radius of the Circle.
Hidden Circles | Incircle of a Triangle (V3)
Find Side (h) Of Given Triangle | Radius of InCircle is 2- Important Geometry Skills Explained
EGD Inscribed Circle
Geometry Marvels: Calculating the Incircle Area Within a Triangle
Area of an Isosceles Triangle with Incircle of Radius r
Radius=? Isosceles triangle inscribed in circle #shorts
Radius of incircle and circumcircle in a right triangle as well as general case
Hidden Circles | Incircle of a Triangle (V2)
Find Area Of InCircle Of An Equilateral Triangle with side length 10 by 2 Methods
finding the radius of a circle inscribed in a right triangle
Shortcuts of Quant- Radius of Incircle and Circumcircle
In-radius & Circum-radius of Equilateral Triangle || Equilateral Triangle Inradius and Circumrad...
Regular Pentagon #youtube #viral #trending #geometry #shorts
There is an incircle of triangle ABC. Two circles touch this incircle and sides of the triangle.
Комментарии
 0:00:51
0:00:51
 0:00:51
0:00:51
 0:03:16
0:03:16
 0:03:42
0:03:42
 0:01:30
0:01:30
 0:10:11
0:10:11
 0:00:24
0:00:24
 0:10:25
0:10:25
 0:03:51
0:03:51
 0:04:31
0:04:31
 0:00:09
0:00:09
 0:05:16
0:05:16
 0:02:14
0:02:14
 0:04:00
0:04:00
 0:11:06
0:11:06
 0:00:50
0:00:50
 0:06:56
0:06:56
 0:00:12
0:00:12
 0:06:59
0:06:59
 0:04:06
0:04:06
 0:06:56
0:06:56
 0:00:40
0:00:40
 0:00:18
0:00:18
 0:10:29
0:10:29