How To Solve An MIT Admissions Question From 1869

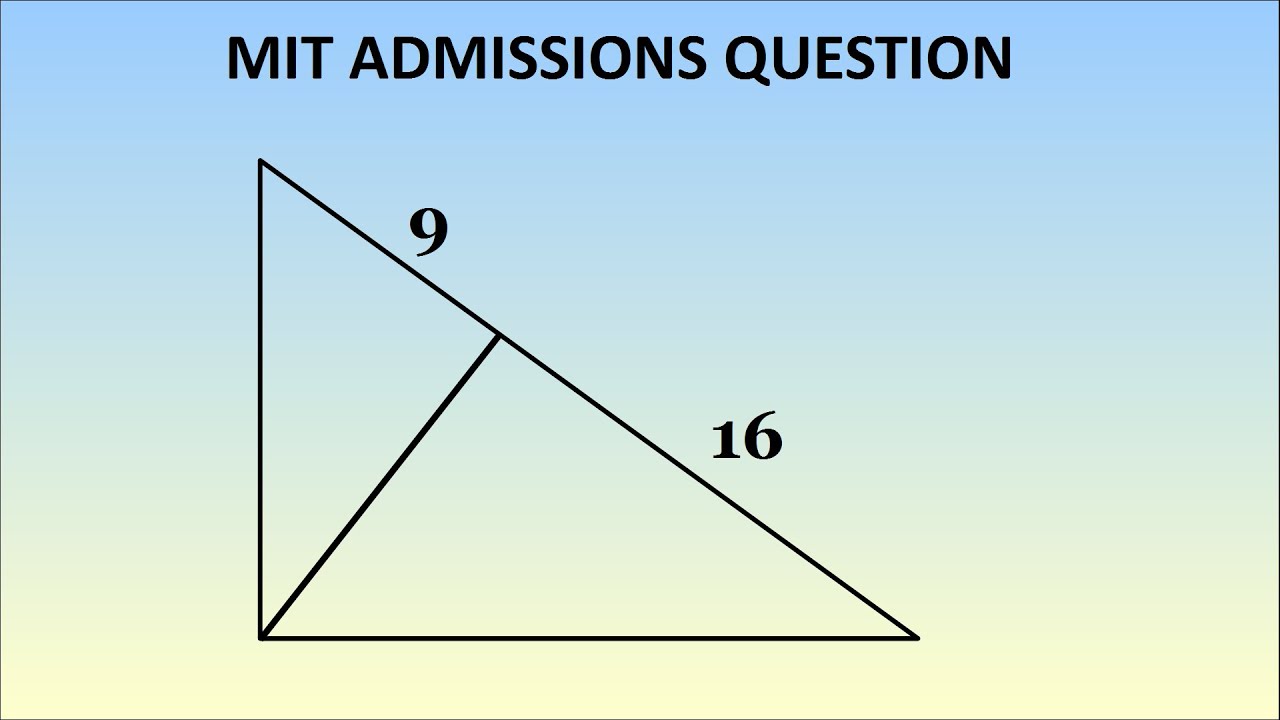
The Massachusetts Institute of Technology (MIT) is one of the top ranked universities in the world. This question appeared on its admissions exam nearly 150 years ago. "The perpendicular dropped from the vertex of the right angle upon the hypotenuse divides it into two segments of 9 and 16 feet respectively. Find the lengths of the perpendicular, and the two legs of the triangle." The video presents a solution.

Sources

Send me suggestions by email (address in video). I consider all ideas though can't always reply!

Why are there comments before the video is published? Get early access and support the channel on Patreon

If you buy from the links below I may receive a commission for sales. This has no effect on the price for you.

Show your support! Get a mug, a t-shirt, and more at Teespring, the official site for Mind Your Decisions merchandise:

My Books
Mind Your Decisions: Five Book Compilation
A collection of 5 books:
"The Joy of Game Theory" rated 4.1/5 stars on 44 reviews
"The Irrationality Illusion: How To Make Smart Decisions And Overcome Bias" rated 3.5/5 stars on 4 reviews
"40 Paradoxes in Logic, Probability, and Game Theory" rated 4.4/5 stars on 13 reviews
"The Best Mental Math Tricks" rated 4.7/5 stars on 8 reviews
"Multiply Numbers By Drawing Lines" rated 4.3/5 stars on 6 reviews

Mind Your Puzzles: Collection Of Volumes 1 To 3
A collection of 3 books:
"Math Puzzles Volume 1" rated 4.4/5 stars on 13 reviews
"Math Puzzles Volume 2" rated 4.5/5 stars on 6 reviews
"Math Puzzles Volume 3" rated 4.1/5 stars on 7 reviews

Connect with me

2017 Shorty Awards Nominee. Mind Your Decisions was nominated in the STEM category (Science, Technology, Engineering, and Math) along with eventual winner Bill Nye; finalists Adam Savage, Dr. Sandra Lee, Simone Giertz, Tim Peake, Unbox Therapy; and other nominees Elon Musk, Gizmoslip, Hope Jahren, Life Noggin, and Nerdwriter.

[MirandaHall]

We're all going to MIT guys, great work.

[motokuchoma]

I used Phytagoras for every step because I'm a fan:

9²+h²=x²
16²+h²=y²
x²+y²=(9+16)²=25²
9²+16²+2h²=25²
2h² = 288
h² = 144
h = 12
16²+12²=y²
400 = y²
y = 20
25²-20² = x²
x² = 225
x = 15

[Mike-evyn]

I solved it differently, using the pythagorean theorem for the whole solution. I labelled the unknown legs as x, y and z. The large triangle formed by combining the 2 smaller triangles is represented with this equation: x²+y² = 25². The triangle to the left is represented by : 9²+z²=x². The 3rd triangle is represented as: z²+16²=y². Now we have 3 unknowns and 3 equations so we can solve by substituting the values of x and y into the first equation and that looks like this: z²+81+z²+256=625 and this reduces to 2z²=288 and then z²=144 so z=12. From here it is easy to solve for x and y.

[ChrisAbbey]

Once you realize it’s a 3-4-5 triangle, you can just sum the segments to get 25 for the outer triangle, see that it is a quintuple and get 15 and 20.

[mxamiss]

MIT would be very crowded if this still existed

[victherocker]

people are like "Wth is this MIT?" "It would be so crowded if it were this easy.."
yo it was 200 years back then, what do you expect?

[brandonpetersen]

Well cool looks like I can just drop out of middle school and go join MIT now

[keshavagarwal]

I was able to solve it. Now I can clear MIT admissions.

Just need to go 150 yrs back in time.
Has anybody got a time machine?

[rafaelliman]

This is certainly one of my favorite puzzles in this channel, i discovered many things here. Apparently, in a right triangle, when you draw the perpendicular from the right angle to the hypotenuse, it divides the triangle into 2 similar triangles, both of which are also similar to the main triangle.

[markusskenins]

You can easily find a sequence of equations -> 9^2 + p^2 = a^2, a^2 + b^2 = 25^2, (b^2=25^2-a2), 16^2 + p2 = b2. 16^2+p2=25^2-(9^2+p2) => p=12, using the pythagorean theorem, you can find the legs - a, b.

[kameshkotwani]

Finally, the first ever question from Mind your decisions, that I did on my own. Thankyou for your service, my rusted brain has started working again.

[aaiishuameta]

Notation
Perpendicular of biggest triangle=a
Base of biggest triangle=b
By Pythagoras theorem solve for 3 triangle we will get
25²=a²+b²
a²=9²+p²
b²=16²+p²
By the help of eq.1 and by adding eq.2 and eq.3 we get
9²+p²+16²+p² =a²+b²=25²
81+256+2p²=625
2p²=625-337=288
p²=288/2=144
p=√144=12
So perpendicular
p=12

[prav]

I just did this and i am not even born yet.

[aliesneo]

simply label the sides as 'a', 'b' and the perpendicular as 'c'. Then solve the system of questions:
1. a^2 = 9^2 + c^2
2. b^2 = 16^2 + c^2
3. a^2 + b^2 = (9+16)^2

use substitution (1) and (2) in (3) and get c = 12, and then a = 15 and b = 20.

none of this scale up and down nonsense. simply use the Pythagorus theorem from grade 5.

[jfb-]

I did it a different way, I called the legs a and b, and applied pythag. 3 times to get
a^2 + b^2 = 25^2,
p^2 + 9^2 = a^2,
p^2 + 16^2 = b^2
Adding equations 2 and 3 gives 2p^2 + 9^2 + 16^2 = a^2 + b^2 = 25^2, rearranging gives p^2 = 144 and p=12, then a and b from there.

[daniilmukhachev]

Using the pythagorean triple you can solve this in a sec.
If we have the triple 3^(2)+4^(2)=5^(2) we can multiply every number by five and again obtain another triple: 15^(2)+20^(2)=25^(2) that gives us the length of the legs of the triangle.

[zahidluqman]

a2+b2=25x25
9x9+p2=a2
16x16+p2=b2
Substituting a and b in terms of p
81+256+2p2=25x25
P2=144. P=12
Substituting value of p
9x9+p2=a2. a=15
16x16+p2=b2.b=20.

[shailiparikh]

Thus actually is the first sum of this channel that I’ve solved on my own. Finally I’ve improved. Thanks for these sums. I tried both Pythagoras theorem as well as similar triangles. Both work

[ricky]

I solved this using the fact that the radius of outer circle is in the middle of the hypotenuse, so it's 12.5 from the middle to every vertex of triangle. so you get another triangle with sides 12.5, 16-12.5=3.5 and p, and you get the p using pythagorrean theorem. once you get the p, similarly you get remaining two legs :)

[Muscleduck]

I would have figured this out 15-20 years ago, but not today. The knowledge was tucked away too deeply.