Introduction to additive combinatorics lecture 7.3 -- dual groups and the discrete Fourier transform

preview_player
Показать описание
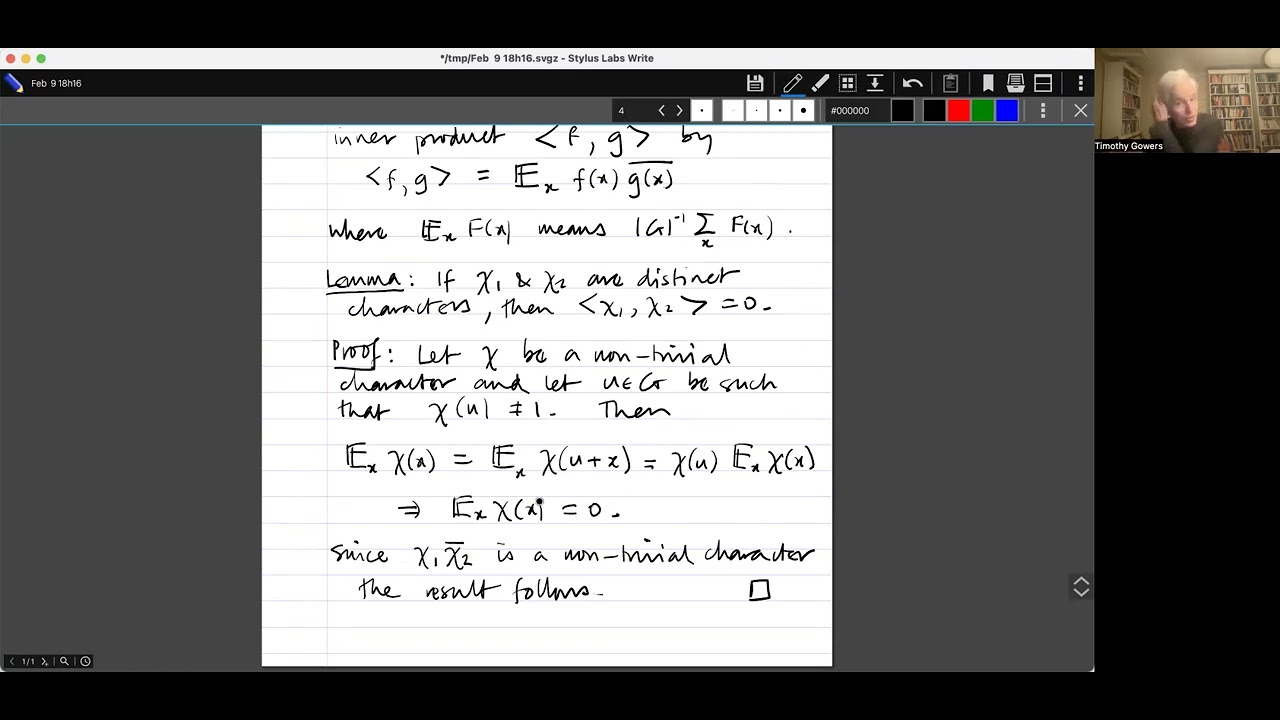
The discrete Fourier transform is a fundamental tool in additive combinatorics that makes it possible to prove many interesting results that would be very hard or even impossible to prove otherwise. Here I discuss the characters on a finite Abelian group G, prove that they are orthogonal and form a group that is (non-naturally) isomorphic to G, known as the dual group of G, and define the Fourier transform, which takes functions defined on G to functions defined on the dual group.

0:00 Introduction
1:22 Characters on finite Abelian groups
3:22 The characters form an Abelian group under pointwise multiplication
6:02 Notation concerning inner products
7:27 Distinct characters are orthogonal
10:53 The characters span the space of functions on G
17:41 G is isomorphic to the dual group
20:39 Notation for and definitions of p-norms and inner products
25:50 Definition of the Fourier transform and discussion of two basic properties

  1. Timothy Gowers
Рекомендации по теме
Introduction to additive 0:40:09

Introduction to additive combinatorics lecture 1.0 --- What is additive combinatorics?

Introduction to additive 0:35:14

Introduction to additive combinatorics lecture 10.1 --- the structure and properties of Bohr sets.

Introduction to additive 0:42:07

Introduction to additive combinatorics lecture 3.7 --- using dependent random selection

Introduction to additive 0:30:37

Introduction to additive combinatorics lecture 7.3 -- dual groups and the discrete Fourier transform

Introduction to additive 0:52:53

Introduction to additive combinatorics lecture 13.0 --- The U2 norm and progressions of length 3.

Introduction to additive 0:43:54

Introduction to additive combinatorics lecture 16.5 --- Szemerédi's theorem for 4APs in F_p^n.

MathSoc Galois LS 0:41:09

MathSoc Galois LS - 'A short introduction to additive combinatorics' by Borys Kuca

Introduction to additive 0:46:26

Introduction to additive combinatorics lecture 11.2 --- Part of the proof of Roth's theorem

Introduction to additive 0:43:40

Introduction to additive combinatorics lecture 1.8 --- Plünnecke's theorem

Introduction to additive 0:40:13

Introduction to additive combinatorics lecture 8.7 --- Bohr sets and Bogolyubov's lemma.

Introduction to additive 0:36:55

Introduction to additive combinatorics lecture 15.8 -- Using earlier tools, and a symmetry argument.

Introduction to additive 0:52:44

Introduction to additive combinatorics lecture 2.7 --- Khovanskii's theorem

Introduction to additive 0:20:56

Introduction to additive combinatorics lecture 10.8 --- A weak form of Freiman's theorem

Introduction to additive 0:44:48

Introduction to additive combinatorics lecture 12.1 --- Finishing the proof of Roth's theorem

Introduction to additive 0:41:52

Introduction to additive combinatorics lecture 7.9 --- Basic Fourier transform properties

Introduction to additive 0:38:36

Introduction to additive combinatorics lecture 5.8 --- Freiman homomorphisms and isomorphisms.

Peter Varju: Additive 0:52:19

Peter Varju: Additive combinatorics methods in fractal geometry - lecture 1

Introduction to additive 0:31:43

Introduction to additive combinatorics lecture 9.5 --- Freiman's theorem for subsets of F_p^N.

Introduction to additive 0:30:24

Introduction to additive combinatorics lecture 14.0 --- The U3 norm and progressions of length 4

Introduction to additive 0:29:53

Introduction to additive combinatorics lecture 5.3 --- Proof of the Balog-Szemerédi theorem

Information Theory and 0:30:54

Information Theory and Additive Combinatorics

Pablo Shmerkin: Additive 0:53:36

Pablo Shmerkin: Additive combinatorics methods in fractal geometry - lecture 1

Introduction to additive 0:30:59

Introduction to additive combinatorics lecture 15.2 --- A large U3 norm leads to additive quadruples

Introduction to additive 0:37:48

Introduction to additive combinatorics lecture 4.5 --- getting all pairs joined by many P3s.