filmov
tv
Computing the exterior derivative of a 1--form | Geometric Algebra
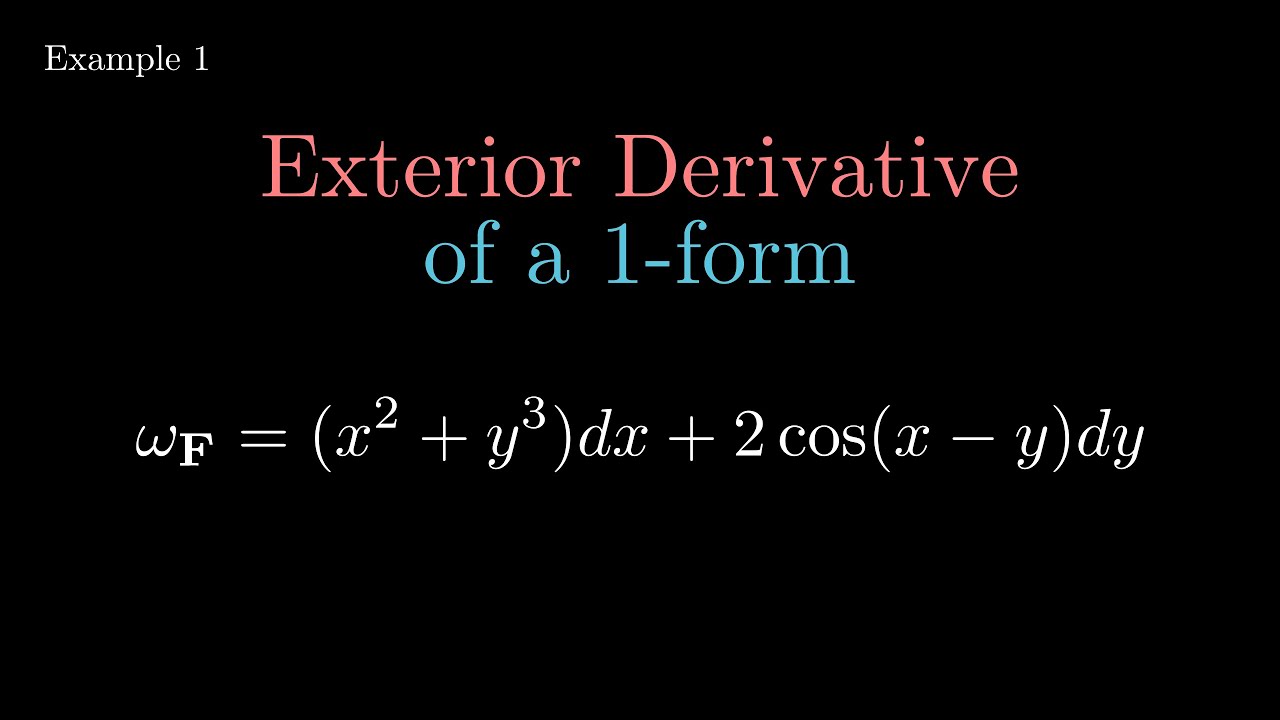
Показать описание
In this video, we look at computing the exterior derivative of a differential form. This will be important when looking at the curl of a vector field, and later, Stokes' theorem.
More videos on geometric algebra and the exterior algebra:
👍 To support the channel, hit the like button and subscribe.
Ways to support the channel:
FYI, these are affiliate links 💪🙏
These videos are separate from my research and teaching roles at the Australian National University, University of Sydney, and Beijing University.
Hi, my name is Kyle and I'm currently doing my doctoral mathematics degree in complex differential geometry under the supervision of Professor Gang Tian and Professor Ben Andrews.
If you would like free access to the manim course without signing up to Skillshare, send me an email and I'll send you a free link to the course :)
These videos are separate from my research and teaching roles at the Australian National University, University of Sydney, and Beijing University.
Vector calculus is an important subject within mathematics, and is an important component of any young mathematician's career, and is sometimes the endpoint of their mathematics degree. This is a subject taken by those with a mathematics major, those doing mathematics for computer science, e.g., artificial intelligence and machine learning, and more broad areas of applied mathematics.
More videos on geometric algebra and the exterior algebra:
👍 To support the channel, hit the like button and subscribe.
Ways to support the channel:
FYI, these are affiliate links 💪🙏
These videos are separate from my research and teaching roles at the Australian National University, University of Sydney, and Beijing University.
Hi, my name is Kyle and I'm currently doing my doctoral mathematics degree in complex differential geometry under the supervision of Professor Gang Tian and Professor Ben Andrews.
If you would like free access to the manim course without signing up to Skillshare, send me an email and I'll send you a free link to the course :)
These videos are separate from my research and teaching roles at the Australian National University, University of Sydney, and Beijing University.
Vector calculus is an important subject within mathematics, and is an important component of any young mathematician's career, and is sometimes the endpoint of their mathematics degree. This is a subject taken by those with a mathematics major, those doing mathematics for computer science, e.g., artificial intelligence and machine learning, and more broad areas of applied mathematics.
Комментарии
 0:03:54
0:03:54
 0:04:45
0:04:45
 0:16:27
0:16:27
 0:05:30
0:05:30
 0:20:18
0:20:18
 0:59:51
0:59:51
 0:59:51
0:59:51
 0:32:38
0:32:38
 0:09:45
0:09:45
 0:11:31
0:11:31
 0:19:40
0:19:40
 0:32:38
0:32:38
 0:45:43
0:45:43
 1:04:22
1:04:22
 0:05:54
0:05:54
 0:14:32
0:14:32
 0:10:23
0:10:23
 0:06:21
0:06:21
 0:11:09
0:11:09
 0:00:33
0:00:33
 0:05:18
0:05:18
 0:06:54
0:06:54
 0:20:34
0:20:34
 0:33:08
0:33:08