filmov
tv
Lecture 6: Exterior Derivative (Discrete Differential Geometry)
Показать описание
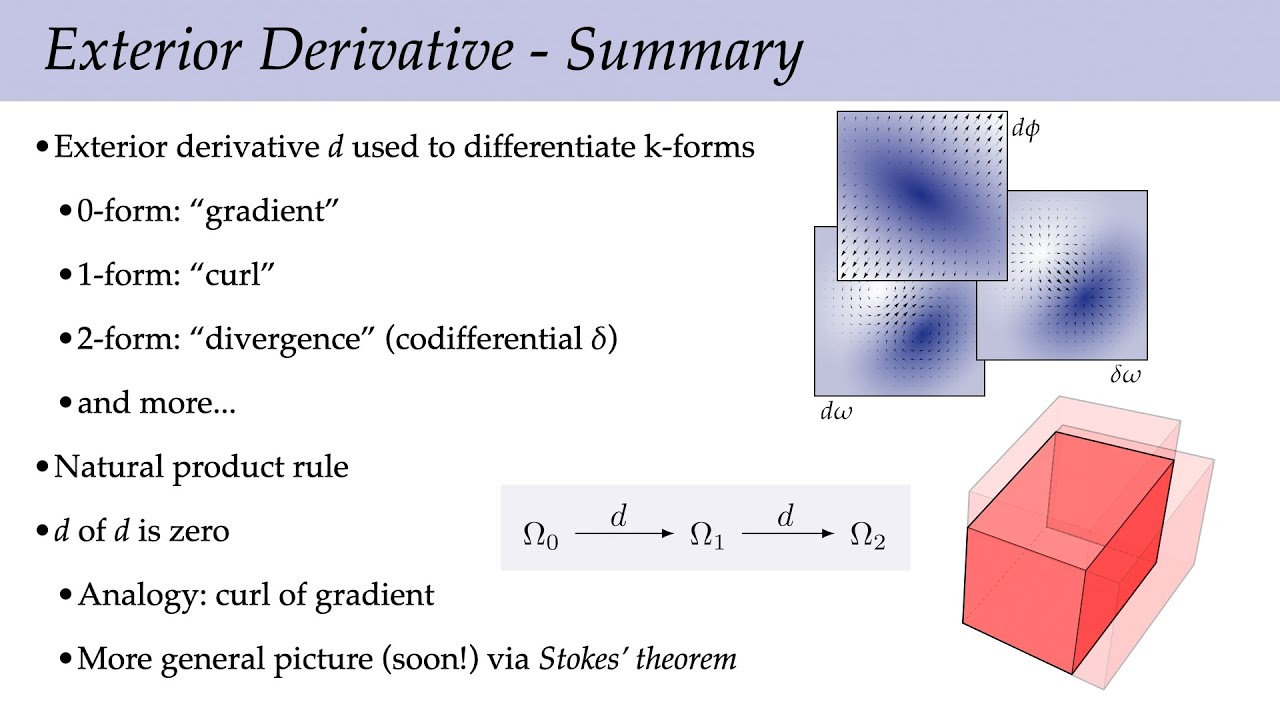
Lecture 6: Exterior Derivative (Discrete Differential Geometry)
Lecture 5: Differential Forms (Discrete Differential Geometry)
BEST DEFENCE ACADEMY IN DEHRADUN | NDA FOUNDATION COURSE AFTER 10TH | NDA COACHING #shorts #nda #ssb
CalcBLUE 4 : Ch. 17.2 : Exterior Derivative Rules
Lecture 9: Discrete Exterior Calculus (Discrete Differential Geometry)
Differential Forms | The exterior derivative.
IIT Bombay Lecture Hall | IIT Bombay Motivation | #shorts #ytshorts #iit
Lecture 3: Exterior Algebra (Discrete Differential Geometry)
Exterior Derivative
noc20 ma01 lec64 The Exterior derivative 5
11 years later ❤️ @shrads
How to eat Roti #SSB #SSB Preparation #Defence #Army #Best Defence Academy #OLQ
Differential Forms | The exterior derivative and vector calculus.
Comment yes for more body language videos! #selfhelp #personaldevelopment #selfimprovement
Differential Forms | The product rule for the exterior derivative.
Trying transition video for the first time 💙😂 || #transformation #transition #shorts #viralvideo...
How much does B.TECH pay?
Bro’s hacking life 😭🤣
Cosplay by b.tech final year at IIT Kharagpur
Lecture 7: Integration (Discrete Differential Geometry)
Tamasha Dekho 😂 IITian Rocks Relatives Shock 😂😂😂 #JEEShorts #JEE #Shorts
Webinar: Discrete Exterior Calculus for Non-smooth Solid Mechanics on Supercomputers
Lecture 4: k-Forms (Discrete Differential Geometry)
The differential form of a derivative.
Комментарии
 1:04:22
1:04:22
 0:45:20
0:45:20
 0:00:15
0:00:15
 0:05:54
0:05:54
 1:09:10
1:09:10
 0:16:27
0:16:27
 0:00:12
0:00:12
 1:02:16
1:02:16
 0:32:38
0:32:38
 0:33:20
0:33:20
 0:00:11
0:00:11
 0:00:16
0:00:16
 0:20:18
0:20:18
 0:00:22
0:00:22
 0:11:31
0:11:31
 0:00:15
0:00:15
 0:00:34
0:00:34
 0:00:20
0:00:20
 0:00:15
0:00:15
 0:57:18
0:57:18
 0:00:13
0:00:13
 1:11:23
1:11:23
 0:55:14
0:55:14
 0:00:53
0:00:53