Uniqueness theorem for Poisson's equation

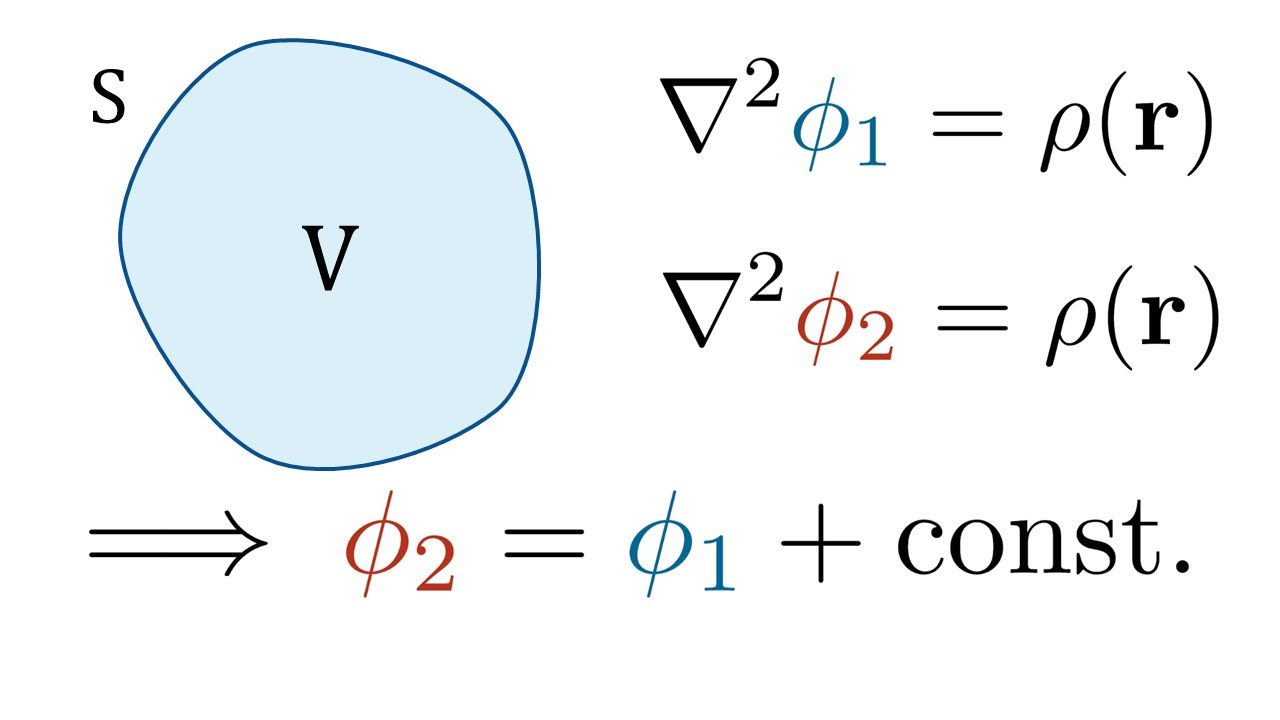
Показать описание

Proving an important theorem that has applications in electrostatics and Newtonian gravity. The theorem states that solutions to Poisson's equation are unique, possibly up to an additive constant (depending on whether Dirichlet or Neumann boundary conditions are given).

About me: I studied Physics at the University of Cambridge, then stayed on to get a PhD in Astronomy. During my PhD, I also spent four years teaching Physics undergraduates at the university. Now, I'm working as a private tutor, teaching Physics & Maths up to A Level standard.

#physics #mathematics #maths #math #poisson #equation #PDEs #laplacian #operator #vectorcalculus #vectors #calculus #boundaryconditions #dirichlet #neumann #uniqueness #theorem #gradient #divergence #gravity #electromagnetism #electrostatics #science #education

Dr Ben Yelverton

Рекомендации по теме

Комментарии

Love your work! You explained well in this video, thank you so much for uploading this.

黃-xh

You should've mentioned that this is one of several uniqueness theorems (this one is for Dirichlet problem). In particular, in Electrostatics there is another one for the case when the charges on the conductors are fixed . For example, if you place uncharged sphere in the electric field, the theorem you presented is not useful.

xgx

Thank you Dr. for your lecture . My question is is this uniqueness theorem is holds for sobolev space? If it is please one video for the proof of it.

Mathswithali

Uniqueness theorem for Poisson's equation

Uniqueness theorem for Poisson's equation

Uniqueness Theorems in Electrostatics | Laplace and Poisson Equation

Poisson's & Laplace's equation and Proof of Uniqueness theorem

Uniqueness proof for Laplace equation (or Poisson equation), Dirichlet problem

Uniqueness: The Physics Problem That Shouldn't Be Solved

Boundary conditions and the uniqueness theorems

Laplace's Equation and Poisson's Equation

Boundary Conditions and Uniqueness Theorems

Laplace's Equation and The First Uniqueness theorem

Lecture - 2.1| Poisson's equation | Laplace's equation | Uniqueness Theorem | Electromagne...

Existence and Uniqueness theorem and how to use it in an example

Special techniques Part 5 First Uniqueness Theorem

Special Techniques Part 6 Second Uniqueness Theorem

Module 3 Poisson's and Laplace's equations, Uniqueness Theorem

Poisson and Laplace Equation, Uniqueness Equation

Uniqueness Theorem: Proof and Derivation Explained

Poisson's Equation and Laplace's Equation

EMT W8 (Uniqueness Theorem)

Lecture Video1 18EE45 Module 3 Laplace equation, Poissons equation, Uniqueness Theorem Derivation Ra

EMT Chapter 6: Laplace, Poisson, and Uniqueness Theorem (Part 2 - Solving Problem using Laplace etc)

EMF (Complete) #22 Poisson's, Laplace Equations and Uniqueness Theorem

IIT Bombay CSE 😍 #shorts #iit #iitbombay

The Existence and Uniqueness Theorem

This chapter closes now, for the next one to begin. 🥂✨.#iitbombay #convocation