filmov
tv
Inverse of a 3x3 Matrix - (THE SIMPLE WAY)

Показать описание
#matrix #inverse #3x3
INVERSE OF A MATRIX
Definition
Let A be any square matrix. If there exists another square matrix B Such that AB = BA = I (I is a unit matrix) then B is called the inverse of the matrix A and is denoted by A-1.
The cofactor method is used to find the inverse of a matrix. Using matrices, the solutions of simultaneous equations are found.
Working Rule to find the inverse of the matrix
Step 1: Find the determinant of the matrix.
Step 2: If the value of the determinant is non zero proceed to find the inverse of the matrix.
Step 3: Find the cofactor of each element and form the cofactor matrix.
Step 4: The transpose of the cofactor matrix is the adjoint matrix.
Step 5: Apply the formula for the inverse
INVERSE OF A MATRIX
Definition
Let A be any square matrix. If there exists another square matrix B Such that AB = BA = I (I is a unit matrix) then B is called the inverse of the matrix A and is denoted by A-1.
The cofactor method is used to find the inverse of a matrix. Using matrices, the solutions of simultaneous equations are found.
Working Rule to find the inverse of the matrix
Step 1: Find the determinant of the matrix.
Step 2: If the value of the determinant is non zero proceed to find the inverse of the matrix.
Step 3: Find the cofactor of each element and form the cofactor matrix.
Step 4: The transpose of the cofactor matrix is the adjoint matrix.
Step 5: Apply the formula for the inverse
Inverse of a 3x3 Matrix
Finding the Inverse of a 3 x 3 Matrix using Determinants and Cofactors - Example 1
Inverse of a 3x3 Matrix - (THE SIMPLE WAY)
Inverse of a 3x3 Matrix | Co-factor Method
Inverse of a 3x3 Matrix using Adjoint | Don't Memories
3x3 Matrizen invertieren | garantiert schneller als dein Dozent
How to Find the Inverse of a 3x3 Matrix
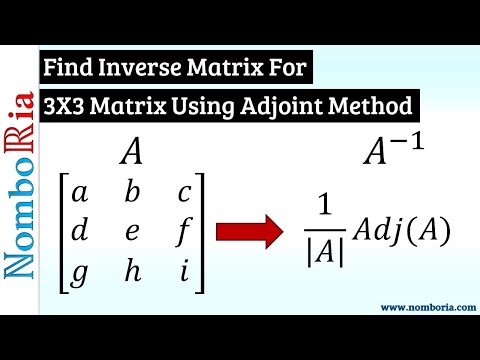
Find Inverse for 3x3 matrix using Adjoint Method
Echelon form of matrix shortcut |Row reduction in 60 SECONDS | Linear Algebra| Matrices
Find the Inverse of a 3x3 Matrix - Use the Elementary Row Operation Method
Find the Inverse of a 3x3 Matrix Using Row Operations and an Augmented Matrix
INVERSE MATRIX 3x3 berechnen – Gauß-Jordan Verfahren, Matrizen invertieren
Finding the Inverse of a 3x3 Matrix
Inverse Matrix bestimmen (Simultanverfahren,3X3-Matrix) | Mathe by Daniel Jung
How to find the inverse of a 3 by 3 matrix (3 methods you need to know)
Learn to Find the Inverse of a 3x3 Matrix | Step-by-Step Tutorial
Ex 1: Inverse of a 3x3 Matrix Using an Augmented Matrix
MATRICES: INVERSE OF A 3x3 MATRIX (determinant, matrix of cofactors, adjoints, Inverse)
Inverting 3x3 part 1: Calculating matrix of minors and cofactor matrix | Precalculus | Khan Academy
Finding Inverse of a 3x3 Matrix Using Determinant, Adjoint(Adjugate),Cofactors|Matrices|Math Dot Com
A-Level Further Maths: C6-10 Inverse Matrices: Finding the Inverse of a 3x3 Matrix
Matrices - Inverse of a 3x3 matrix | ExamSolutions - maths problems answered
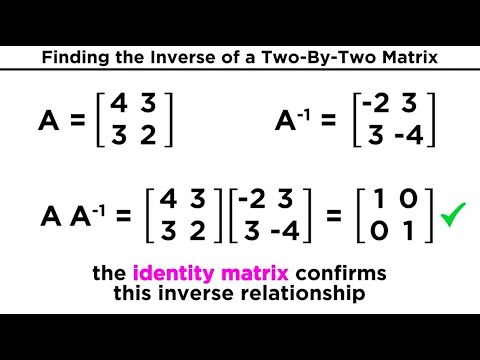
Inverse Matrices and Their Properties
Inverse of 3x3 Matrix using Row Reduction
Комментарии
 0:15:21
0:15:21
 0:06:46
0:06:46
 0:15:14
0:15:14
 0:13:55
0:13:55
 0:05:01
0:05:01
 0:06:20
0:06:20
 0:05:36
0:05:36
 0:09:57
0:09:57
 0:03:13
0:03:13
 0:08:20
0:08:20
 0:10:48
0:10:48
 0:10:07
0:10:07
 0:05:16
0:05:16
 0:08:02
0:08:02
 0:24:48
0:24:48
 0:09:44
0:09:44
 0:07:10
0:07:10
 0:07:23
0:07:23
 0:08:47
0:08:47
 0:10:35
0:10:35
 0:09:09
0:09:09
 0:06:54
0:06:54
 0:12:00
0:12:00
 0:18:00
0:18:00