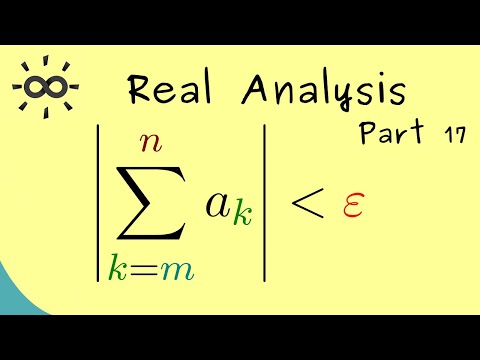
Real Analysis 17 | Cauchy Criterion

Please consider to support me if this video was helpful such that I can continue to produce them :)

🙏 Thanks to all supporters! They are mentioned in the credits of the video :)

This is my video series about Real Analysis. We talk about sequences, series, continuous functions, differentiable functions, and integral. I hope that it will help everyone who wants to learn about it.

x

00:00 Intro
00:36 Properties of series
02:20 Cauchy Criterion
03:46 Proof
05:29 Example
07:15 Conclusion
08:47 Credits

#RealAnalysis
#Mathematics
#Calculus
#LearnMath
#Integrals
#Derivatives

I hope that this helps students, pupils and others. Have fun!

(This explanation fits to lectures for students in their first and second year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)

[qiaohuizhou]

0:35 properties of series
2:22 Cauchy criterion
3:45 use completeness axiom to prove
7:25 necessary condition for the convergent series

[douglasstrother]

So far, Real Analysis seems pretty straightforward.

Understanding the nuances of the definitions takes the most work.

I'm interested to see what unfolds.

[zachsiegel]

"it's not a restriction at all because the only case we really miss is when we have the same index here: (5:01). Why do we not need to consider the case where m < n?

[hipolitdobrohna]

I have one problem with a reformulation of being Cauchy sequence: for n>=m>=N and taking m=N, we have |s_n - s_(N-1)|<epsilon, which i think is not guaranteed by the definition, if we want to have the same index N. Is it here something what I don't see?

[sayan]

Love from India. You are doing a great job. Please cover complex analysis, PDE, differential geometry etc. Also, if you could give sketch of the proofs of the major theorems (like open mapping), that would be great. Your lectures are crystal clear. Thanks a lot for uploading.

[thealphanigga]

How you say "calculate" fascinates me and keeps me interested.

[bishwajitsarma]

If N=1 (suppose), then S_m-1 foe m=1 is not defined. Am i correct?

[MrOvipare]

In the beginning you said something like "we can do this because the series are convergent" after explaining their properties (a) and (b)... This reminded me of the famous result that 1 + 2 + 3 + 4 + ... = - 1/12 which I always found suspicious. I know we can have this result by using the Riemann zeta function, which is an analytic continuation... I can accept that, but 0n the wiki of "1 + 2 + 3 + 4 + ..." they show that Ramanujuan expressed the sum of natural numbers as c, then multiplied it by 4 and expressed c - 4c, then solved for c to obtain c = - 1/12.
Don't you agree that those operations were not really "legal" since he manipulates divergent series as if they were numbers? If we stayed domain of natural numbers, it seems to me that the result would simply not be true, or at least it would be "undefined".

[belfiore]

I didnt understand ... why did zou change the series into that at 5:42 ?