Proof: Sequence is Cauchy if and only if it Converges | Real Analysis

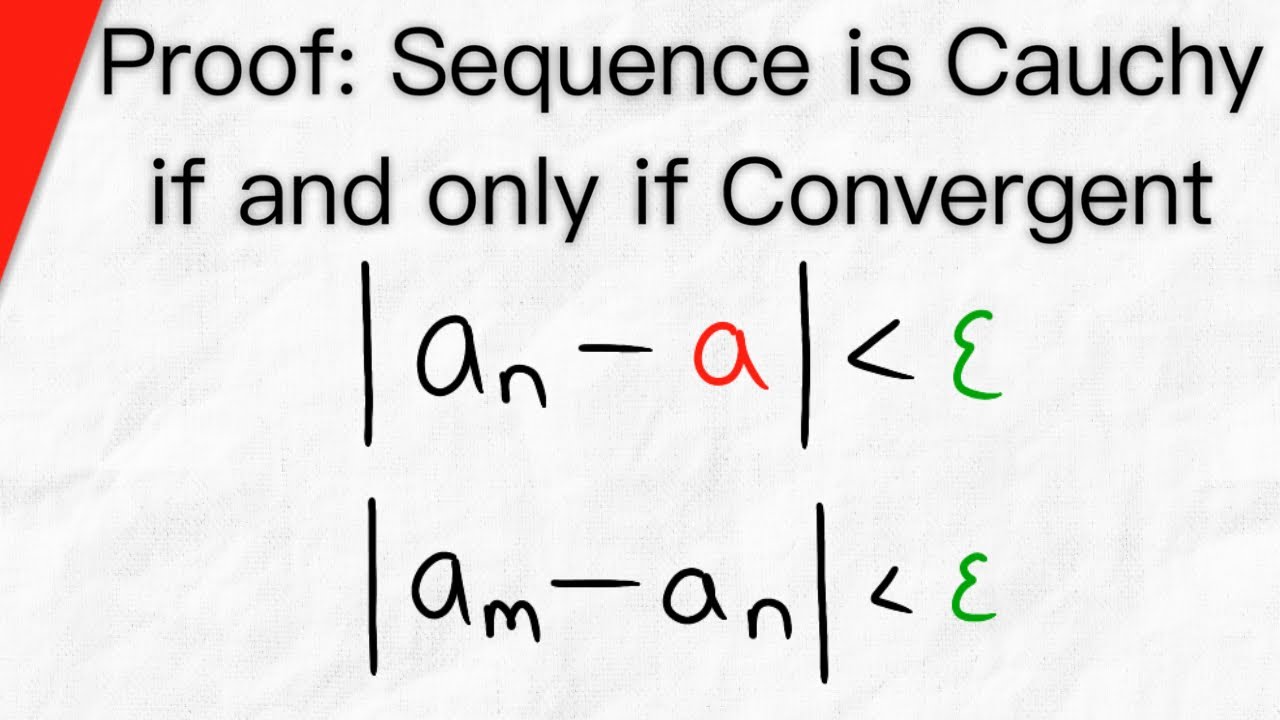
We prove that a sequence converges if and only if it is Cauchy! This means that if a sequence converges then it is Cauchy, and if a sequence is Cauchy then it converges. Note that the definition of a Cauchy sequence has nothing to do with the particular limit of the sequence, so this gives us a way to prove a sequence converges without knowing its limit - we simply prove it is Cauchy! Before we prove either direction of this result we will prove that Cauchy sequences are bounded, since we use this result in the proof. With this proof done, we have got an awful lot of value from the Cauchy criterion for sequences!

Table of Contents
0:00 Intro
2:14 Every Cauchy Sequence is Bounded
7:17 Every Convergent Sequence is Cauchy
12:31 Every Cauchy Sequence is Convergent

#RealAnalysis #Math

Proof of Bolzano-Weierstrass Theorem (coming soon):

★DONATE★

Thanks to Robert Rennie, Barbara Sharrock, and Rolf Waefler for their generous support on Patreon!

Follow Wrath of Math on...

[WrathofMath]

Please share this video if it is helpful and you'd like to see more real analysis lessons!

[mikechen]

Let's claim this is the most easy-to-understand video on Cauchy criterion on youtube!

[like_that]

bounded directly mean convergent right???
why not just simply apply that?

[alishashoukat]

Can you please explain it by graph too?

[tulikellyklaudia]

but how did a sub n become positive and a sub m become negative?

[iitian]

Every cauchy sequence is convergent
Is it true always?

[spacemanjr]

its not if and only if, only Converge -> Cauchy.