filmov
tv
Calculus - The Most Difficult Integral - sqrt(tan(x)) (Request)
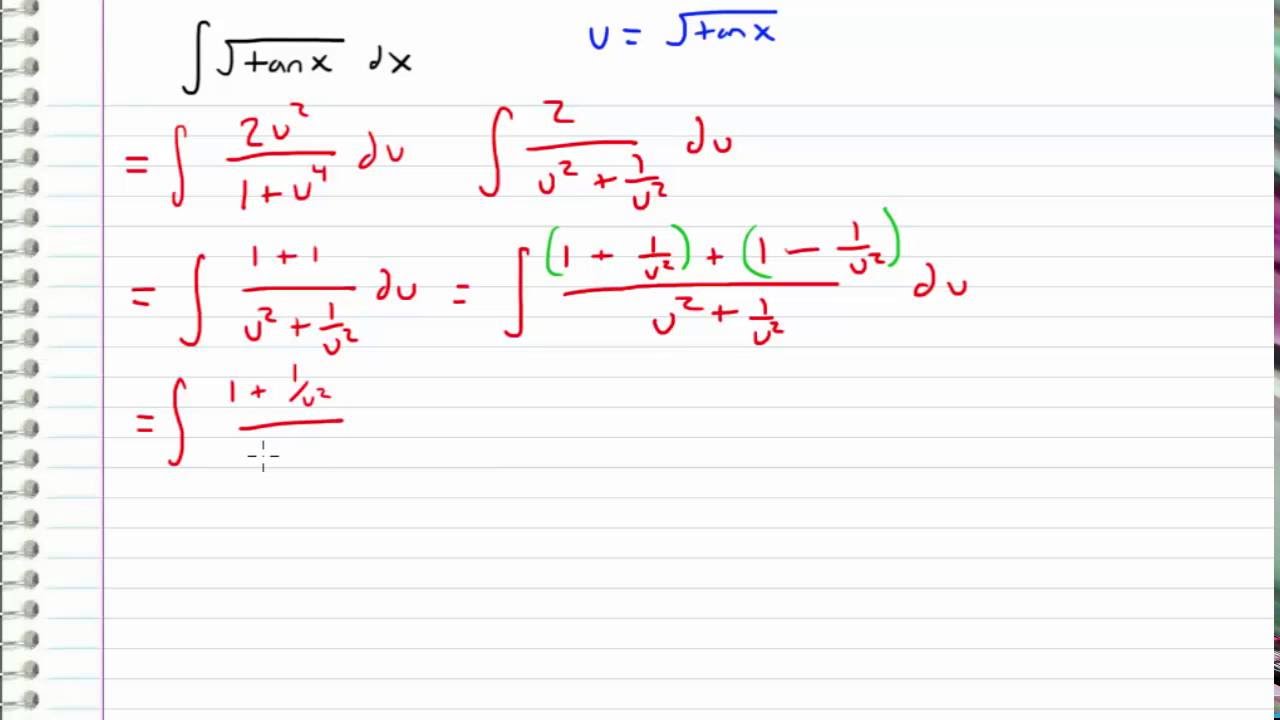
Показать описание
**MISTAKE**
**************************************
I miscopied the signs on the last term the final answer when I combined both parts of the integral. The computations of the two integrals is correct, so please refer to those! The final term should go: +,- on numerator and +,+ on denominator. Annotations have been added to show these corrections.
***********************************
This is the most difficult integral that I have solved so far. If you can think of harder one, let me know! Remember to submit any questions / requests that you may have to get your own solution video like this one! Enjoy.
**************************************
I miscopied the signs on the last term the final answer when I combined both parts of the integral. The computations of the two integrals is correct, so please refer to those! The final term should go: +,- on numerator and +,+ on denominator. Annotations have been added to show these corrections.
***********************************
This is the most difficult integral that I have solved so far. If you can think of harder one, let me know! Remember to submit any questions / requests that you may have to get your own solution video like this one! Enjoy.
What is the Hardest Calculus Course?
Math Integration Timelapse | Real-life Application of Calculus #math #maths #justicethetutor
The Hardest Math Test
The 7 Levels of Math
The World’s Hardest Math Class
The hardest problem on the hardest test
Calculus is the Hardest Math Class People Take
The Hardest Math Class in the World?!?!
Mathematical chit-chat : Some random thoughts about doing mathematics.
The most dangerous problem in math
The Hardest Problem on the SAT📚 | Algebra | Math
I visited the world's hardest math class
Hardest Question on the SAT Explained | Math & Algebra Made Easy | JusticeTheTutor #shorts
Can you guess the math formula?
Calculus in a nutshell
What Makes Calculus Hard #shorts
The Simplest Math Problem No One Can Solve - Collatz Conjecture
The Hardest Calculus 2 Test I've Ever Given(Nobody got an A)
When mathematicians get bored (ep1)
This Is the Calculus They Won't Teach You
the 5 hardest exams in the world
We Need To Talk About Calculus 2
5 simple unsolvable equations
How REAL Men Integrate Functions
Комментарии
 0:01:44
0:01:44
 0:00:09
0:00:09
 0:00:28
0:00:28
 0:08:44
0:08:44
 0:00:34
0:00:34
 0:11:15
0:11:15
 0:02:46
0:02:46
 0:03:58
0:03:58
 0:29:08
0:29:08
 0:01:00
0:01:00
 0:00:31
0:00:31
 0:12:50
0:12:50
 0:00:46
0:00:46
 0:00:53
0:00:53
 0:03:01
0:03:01
 0:00:29
0:00:29
 0:22:09
0:22:09
 0:07:06
0:07:06
 0:00:37
0:00:37
 0:30:17
0:30:17
 0:17:14
0:17:14
 0:08:55
0:08:55
 0:00:50
0:00:50
 0:00:35
0:00:35