filmov
tv
Classical Mechanics | Lecture 7

Показать описание
(November 7, 2011) Leonard Susskind discusses the some of the basic laws and ideas of modern physics. In this lecture, he focuses on Liouville's Theorem, which he describes as one of the basis for Hamiltonian mechanics. He works to prove the reversibility of classical mechanics.
This course is the beginning of a six course sequence that explores the theoretical foundations of modern physics. Topics in the series include classical mechanics, quantum mechanics, theories of relativity, electromagnetism, cosmology, and black holes.
Stanford University
Stanford Continuing Studies
Stanford University Channel on YouTube:
This course is the beginning of a six course sequence that explores the theoretical foundations of modern physics. Topics in the series include classical mechanics, quantum mechanics, theories of relativity, electromagnetism, cosmology, and black holes.
Stanford University
Stanford Continuing Studies
Stanford University Channel on YouTube:
Classical Mechanics | Lecture 7
Statistical Mechanics Lecture 7
Lecture 7: More on Energy Eigenstates
Lecture 7 | The Theoretical Minimum
Classical Mechanics | Lecture-7 | GATE | IIT JAM | Physics Academy
Special Relativity | Lecture 7
Brian Cox explains quantum mechanics in 60 seconds - BBC News
Symplectic geometry & classical mechanics, Lecture 7
Advanced Quantum Mechanics Lecture 7
The Biggest Ideas in the Universe | 7. Quantum Mechanics
Lecture 7: A Political History of Gravity
CSIR NET /JRF 2023 I CLASSICAL MECHANICS I LECTURE-7
General Relativity Lecture 7
GENERALISED VELOCITY AND ACCELERATION || KINETIC ENERGY || LECTURE 7 || CLASSICAL MECHANICS
Classical Mechanics, Lecture 7: Noether's Theorem. Two Body Problem.
7. Classical Mechanical Harmonic Oscillator
Classical mechanics; Lecture-7: Hamilton's Principle for Holonomic Constraints
METU - Quantum Mechanics II - Week 7 - Lecture 2
Lagrangian and Hamiltonian Mechanics in Under 20 Minutes: Physics Mini Lesson
Lagrangian Part 2 - #7 Classical Mechanics Lecture Series
Lecture 6: Time Evolution and the Schrödinger Equation
Classical Mechanics | Lecture 8
Lecture 7: Applications of Relativity (Special Relativity - English) | Pervez Hoodbhoy
Lecture 7 - DYNAMICS - Kinematics of Particles - Part 1
Комментарии
 1:47:17
1:47:17
 1:50:27
1:50:27
 1:15:54
1:15:54
 2:11:22
2:11:22
 0:56:07
0:56:07
 1:46:27
1:46:27
 0:01:22
0:01:22
 1:11:33
1:11:33
 1:27:17
1:27:17
 1:05:28
1:05:28
 1:19:35
1:19:35
 2:21:16
2:21:16
 1:54:50
1:54:50
 0:23:10
0:23:10
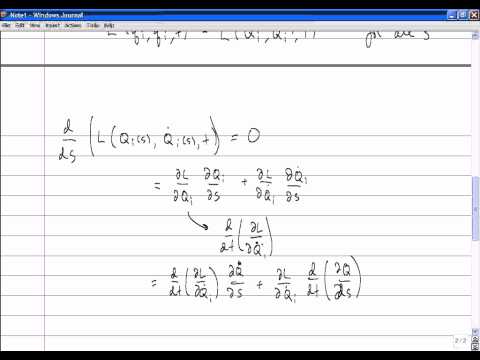 0:48:58
0:48:58
 0:51:11
0:51:11
 0:53:26
0:53:26
 0:50:13
0:50:13
 0:18:33
0:18:33
 1:54:30
1:54:30
 1:22:19
1:22:19
 1:38:07
1:38:07
 0:43:11
0:43:11
 1:20:50
1:20:50